
AllQuestion and Answers: Page 555
Question Number 162814 Answers: 2 Comments: 0
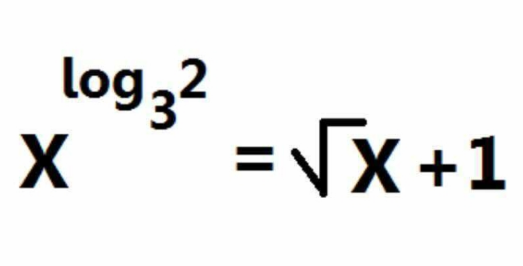
Question Number 162811 Answers: 1 Comments: 0
Question Number 162809 Answers: 2 Comments: 0
Question Number 162804 Answers: 2 Comments: 0
Question Number 162794 Answers: 0 Comments: 0
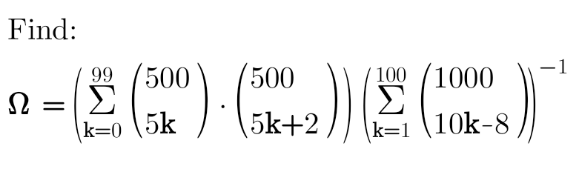
Question Number 162792 Answers: 1 Comments: 0
Question Number 162791 Answers: 3 Comments: 0

Question Number 162790 Answers: 1 Comments: 0
Question Number 162788 Answers: 1 Comments: 0
Question Number 162787 Answers: 1 Comments: 0
Question Number 162783 Answers: 0 Comments: 0
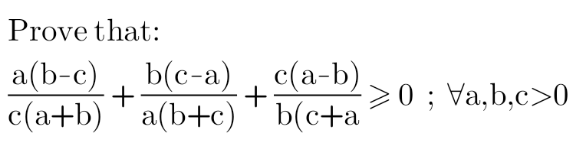
Question Number 162782 Answers: 0 Comments: 0

Question Number 162776 Answers: 3 Comments: 0
Question Number 162775 Answers: 2 Comments: 0
Question Number 162785 Answers: 1 Comments: 0
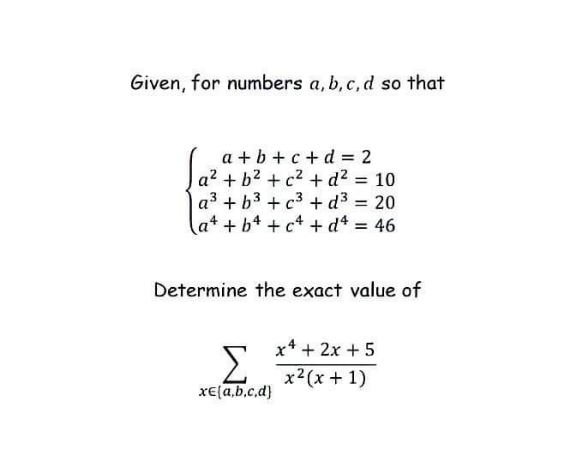
Question Number 162747 Answers: 1 Comments: 0

Question Number 162744 Answers: 0 Comments: 0

Question Number 162734 Answers: 1 Comments: 0
Question Number 163110 Answers: 1 Comments: 0

Question Number 162728 Answers: 2 Comments: 2

Question Number 162726 Answers: 1 Comments: 0
Question Number 162718 Answers: 0 Comments: 0
Question Number 162702 Answers: 0 Comments: 0
Question Number 162701 Answers: 1 Comments: 0
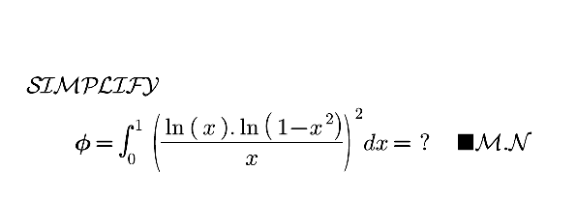
Question Number 164806 Answers: 1 Comments: 7

Question Number 162721 Answers: 3 Comments: 0
Pg 550 Pg 551 Pg 552 Pg 553 Pg 554 Pg 555 Pg 556 Pg 557 Pg 558 Pg 559
