Question and Answers Forum

AllQuestion and Answers: Page 554

Question Number 161281 Answers: 0 Comments: 0

Question Number 161280 Answers: 1 Comments: 0

if x;y;z>0 and (1/(1+x)) + (1/(1+y)) + (1/(1+z)) = 1 then prove that: x + y + z ≥ (3/4) xyz

$$\mathrm{if}\:\:\mathrm{x};\mathrm{y};\mathrm{z}>\mathrm{0}\:\:\mathrm{and}\:\:\frac{\mathrm{1}}{\mathrm{1}+\mathrm{x}}\:+\:\frac{\mathrm{1}}{\mathrm{1}+\mathrm{y}}\:+\:\frac{\mathrm{1}}{\mathrm{1}+\mathrm{z}}\:=\:\mathrm{1} \\ $$$$\mathrm{then}\:\mathrm{prove}\:\mathrm{that}: \\ $$$$\mathrm{x}\:+\:\mathrm{y}\:+\:\mathrm{z}\:\geqslant\:\frac{\mathrm{3}}{\mathrm{4}}\:\mathrm{xyz} \\ $$

Question Number 161272 Answers: 0 Comments: 0

Solve the differential systeme (Σ) below: (Σ) { ((x^. (t)=x(t)+2y(t)+t)),((y^. (t)=−4x(t)−3y(t))) :}

$$\mathrm{Solve}\:\mathrm{the}\:\mathrm{differential}\:\mathrm{systeme}\:\left(\Sigma\right)\:\mathrm{below}: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\Sigma\right)\begin{cases}{\overset{.} {{x}}\left({t}\right)={x}\left({t}\right)+\mathrm{2}{y}\left({t}\right)+{t}}\\{\overset{.} {{y}}\left({t}\right)=−\mathrm{4}{x}\left({t}\right)−\mathrm{3}{y}\left({t}\right)}\end{cases}\: \\ $$

Question Number 161265 Answers: 1 Comments: 2

Question Number 161257 Answers: 2 Comments: 1

Question Number 161256 Answers: 1 Comments: 0

Given f(x)=f(x+2), ∀x∈R If ∫_0 ^2 f(x)dx= p then ∫_0 ^(2020) f(x+2a)dx=? for a∈Z^+

$$\:{Given}\:{f}\left({x}\right)={f}\left({x}+\mathrm{2}\right),\:\forall{x}\in\mathbb{R} \\ $$$$\:{If}\:\underset{\mathrm{0}} {\overset{\mathrm{2}} {\int}}{f}\left({x}\right){dx}=\:{p}\:{then}\:\underset{\mathrm{0}} {\overset{\mathrm{2020}} {\int}}{f}\left({x}+\mathrm{2}{a}\right){dx}=? \\ $$$$\:{for}\:{a}\in\mathbb{Z}^{+} \\ $$

Question Number 161254 Answers: 0 Comments: 1

((4sin (((2π)/7))+sec ((π/(14))))/(cot ((π/7))))=?

$$\:\frac{\mathrm{4sin}\:\left(\frac{\mathrm{2}\pi}{\mathrm{7}}\right)+\mathrm{sec}\:\left(\frac{\pi}{\mathrm{14}}\right)}{\mathrm{cot}\:\left(\frac{\pi}{\mathrm{7}}\right)}=? \\ $$

Question Number 161251 Answers: 0 Comments: 0

Question Number 161248 Answers: 1 Comments: 0

lim_(x→0) (((1+sin^3 x)^4 −(1+tan^3 x)^4 )/x^5 ) =?

$$\:\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\left(\mathrm{1}+\mathrm{sin}\:^{\mathrm{3}} {x}\right)^{\mathrm{4}} −\left(\mathrm{1}+\mathrm{tan}\:^{\mathrm{3}} {x}\right)^{\mathrm{4}} }{{x}^{\mathrm{5}} }\:=?\: \\ $$

Question Number 161242 Answers: 1 Comments: 0

x ; y ; z < 0 (x^3 /( (√(yz)))) = -3 ; (y^3 /( (√(xz)))) = -6 ; (z^3 /( (√(xy)))) = -8 find x∙y∙z = ?

$$\mathrm{x}\:;\:\mathrm{y}\:;\:\mathrm{z}\:<\:\mathrm{0} \\ $$$$\frac{\mathrm{x}^{\mathrm{3}} }{\:\sqrt{\mathrm{yz}}}\:=\:-\mathrm{3}\:\:;\:\:\frac{\mathrm{y}^{\mathrm{3}} }{\:\sqrt{\mathrm{xz}}}\:=\:-\mathrm{6}\:\:;\:\:\frac{\mathrm{z}^{\mathrm{3}} }{\:\sqrt{\mathrm{xy}}}\:=\:-\mathrm{8} \\ $$$$\mathrm{find}\:\:\mathrm{x}\centerdot\mathrm{y}\centerdot\mathrm{z}\:=\:? \\ $$

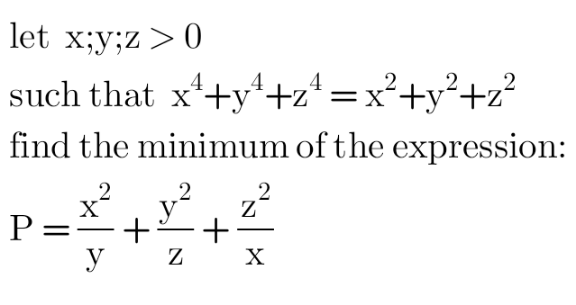
Question Number 161241 Answers: 2 Comments: 0

Question Number 161238 Answers: 0 Comments: 0

Question Number 161234 Answers: 0 Comments: 1

1:!x=? 2:!!x=? 3:x!!=? when X is odd 4:x!!=? when X is evan 5:x!!!=? when X is odd 6:x!!!=? when X is evan

$$\mathrm{1}:!{x}=? \\ $$$$\mathrm{2}:!!{x}=? \\ $$$$\mathrm{3}:{x}!!=?\:\:\:\:{when}\:\:\:{X}\:\:{is}\:\:{odd} \\ $$$$\mathrm{4}:{x}!!=?\:\:\:\:{when}\:\:{X}\:\:{is}\:\:{evan} \\ $$$$\mathrm{5}:{x}!!!=?\:\:\:\:\:\:\:{when}\:{X}\:{is}\:{odd} \\ $$$$\mathrm{6}:\mathrm{x}!!!=?\:\:\:\:\:\:\:{when}\:{X}\:\:{is}\:{evan} \\ $$

Question Number 161233 Answers: 0 Comments: 0

Question Number 161229 Answers: 1 Comments: 0

Given f(x)= { ((1−∣x∣ ; x≤1)),((∣x∣−1 ; x>1)) :} find ∫_(−3) ^( 8) [f(x−1)+f(x+1)] dx.

$$\:{Given}\:{f}\left({x}\right)=\:\begin{cases}{\mathrm{1}−\mid{x}\mid\:;\:{x}\leqslant\mathrm{1}}\\{\mid{x}\mid−\mathrm{1}\:;\:{x}>\mathrm{1}}\end{cases} \\ $$$$\:{find}\:\int_{−\mathrm{3}} ^{\:\mathrm{8}} \left[{f}\left({x}−\mathrm{1}\right)+{f}\left({x}+\mathrm{1}\right)\right]\:{dx}.\: \\ $$

Question Number 161215 Answers: 1 Comments: 1

log x_2 +log2_x =((e^2 +1)/e) x=?

$$\mathrm{log}\:\underset{\mathrm{2}} {{x}}+{log}\underset{{x}} {\mathrm{2}}=\frac{{e}^{\mathrm{2}} +\mathrm{1}}{{e}}\:\:\:\:\:\:\:\:\:\:{x}=? \\ $$

Question Number 161214 Answers: 0 Comments: 6

Question Number 161212 Answers: 2 Comments: 2

∫_( 0) ^( (π/2)) ((x sin x cos x)/(cos^4 x +sin^4 x)) dx =?

$$\:\:\int_{\:\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \:\frac{{x}\:\mathrm{sin}\:{x}\:\mathrm{cos}\:{x}}{\mathrm{cos}\:^{\mathrm{4}} {x}\:+\mathrm{sin}\:^{\mathrm{4}} {x}}\:{dx}\:=? \\ $$

Question Number 161209 Answers: 0 Comments: 1

Differentiate y=e^(−x^2 )

$${Differentiate}\:{y}={e}^{−{x}^{\mathrm{2}} } \\ $$

Question Number 161205 Answers: 0 Comments: 0

Soit f: E→F une application. P(E) est l′ensemble des parties de E. Montrer que: f est surjective⇔∀ B ∈ P(E), f(f^(−1) (B))=B.

$${Soit}\:{f}:\:{E}\rightarrow{F}\:{une}\:{application}.\: \\ $$$$\mathscr{P}\left({E}\right)\:{est}\:{l}'{ensemble}\:{des}\:{parties} \\ $$$${de}\:{E}.\:{Montrer}\:{que}: \\ $$$${f}\:{est}\:{surjective}\Leftrightarrow\forall\:{B}\:\in\:\mathscr{P}\left({E}\right),\:{f}\left({f}^{−\mathrm{1}} \left({B}\right)\right)={B}. \\ $$

Question Number 161204 Answers: 0 Comments: 0

Soit f: E→F , une application. P(E) est l′ensemble des parties de E. Montrer que f est injective ⇔ ∀ A ∈ P(E), A ⊂ f^(−1) (f(A))

$${Soit}\:{f}:\:{E}\rightarrow{F}\:,\:{une}\:{application}. \\ $$$$\mathscr{P}\left({E}\right)\:{est}\:{l}'{ensemble}\:{des}\:{parties} \\ $$$${de}\:{E}. \\ $$$${Montrer}\:{que}\:{f}\:{est}\:{injective}\:\Leftrightarrow\:\forall\:{A}\:\in\:\mathscr{P}\left({E}\right),\:{A}\:\subset\:{f}^{−\mathrm{1}} \left({f}\left({A}\right)\right) \\ $$

Question Number 161203 Answers: 0 Comments: 0

Rashid Shindhi− I have a question to u that how do u cut the x of nominator and denominator?

$${Rashid}\:{Shindhi}−\:{I}\:{have}\:{a}\:{question}\:{to}\:{u}\:{that}\:{how}\:{do}\:{u}\:{cut}\:{the}\:{x}\:{of}\:\:{nominator}\:{and}\:{denominator}? \\ $$

Question Number 161202 Answers: 0 Comments: 0

lim_(n→∞) Σ_(k=1) ^n ((k/n))^n =?

$$\underset{\mathrm{n}\rightarrow\infty} {\mathrm{lim}}\underset{\mathrm{k}=\mathrm{1}} {\overset{\mathrm{n}} {\sum}}\left(\frac{\mathrm{k}}{\mathrm{n}}\right)^{\mathrm{n}} =? \\ $$

Question Number 161196 Answers: 0 Comments: 0

Montrons que B_2 (0, 1) est homeomorphe a^ R^2 . Ou^ B_2 (0, 1)=boule unite^ de R^2 .

$$\mathrm{Montrons}\:\mathrm{que}\:\mathrm{B}_{\mathrm{2}} \left(\mathrm{0},\:\mathrm{1}\right)\:\mathrm{est}\:\mathrm{homeomorphe}\:\grave {\mathrm{a}}\:\mathbb{R}^{\mathrm{2}} . \\ $$$$\mathrm{O}\grave {\mathrm{u}}\:\mathrm{B}_{\mathrm{2}} \left(\mathrm{0},\:\mathrm{1}\right)=\mathrm{boule}\:\mathrm{unit}\acute {\mathrm{e}}\:\mathrm{de}\:\mathbb{R}^{\mathrm{2}} . \\ $$

Question Number 161194 Answers: 0 Comments: 1

TAKEN FROM REAL LIFE if I try to walk into a shop through a super clean closed glass door and get myself a bloody nose then what′s the value of the brake acceleration when we assume 1) my walking speed is 7km/hr 2) the crumple zone of my nose is 1/2′′ 3) the brake acceleration is constant

$$\mathbb{TAKEN}\:\mathbb{FROM}\:\mathbb{REAL}\:\mathbb{LIFE} \\ $$$$\mathrm{if}\:\mathrm{I}\:\mathrm{try}\:\mathrm{to}\:\mathrm{walk}\:\mathrm{into}\:\mathrm{a}\:\mathrm{shop}\:\mathrm{through}\:\mathrm{a}\:\mathrm{super} \\ $$$$\mathrm{clean}\:\mathrm{closed}\:\mathrm{glass}\:\mathrm{door}\:\mathrm{and}\:\mathrm{get}\:\mathrm{myself}\:\mathrm{a} \\ $$$$\mathrm{bloody}\:\mathrm{nose}\:\mathrm{then}\:\mathrm{what}'\mathrm{s}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{brake}\:\mathrm{acceleration}\:\mathrm{when}\:\mathrm{we}\:\mathrm{assume} \\ $$$$\left.\mathrm{1}\right)\:\mathrm{my}\:\mathrm{walking}\:\mathrm{speed}\:\mathrm{is}\:\mathrm{7km}/\mathrm{hr} \\ $$$$\left.\mathrm{2}\right)\:\mathrm{the}\:\mathrm{crumple}\:\mathrm{zone}\:\mathrm{of}\:\mathrm{my}\:\mathrm{nose}\:\mathrm{is}\:\mathrm{1}/\mathrm{2}'' \\ $$$$\left.\mathrm{3}\right)\:\mathrm{the}\:\mathrm{brake}\:\mathrm{acceleration}\:\mathrm{is}\:\mathrm{constant} \\ $$

Question Number 161192 Answers: 0 Comments: 2

Pg 549 Pg 550 Pg 551 Pg 552 Pg 553 Pg 554 Pg 555 Pg 556 Pg 557 Pg 558

Contact: info@tinkutara.com