Question and Answers Forum

AllQuestion and Answers: Page 535

Question Number 164473 Answers: 1 Comments: 0

Show for z_1 ; z_(2 ) ∈ C that: ∣z_1 +z_2 ∣^2 +∣z_1 −z_2 ∣^2 =2(∣z_1 ∣^2 +∣z_2 ∣^2 ).

$${Show}\:{for}\:{z}_{\mathrm{1}} ;\:{z}_{\mathrm{2}\:} \:\in\:\mathbb{C}\:{that}: \\ $$$$\mid{z}_{\mathrm{1}} +{z}_{\mathrm{2}} \mid^{\mathrm{2}} +\mid{z}_{\mathrm{1}} −{z}_{\mathrm{2}} \mid^{\mathrm{2}} =\mathrm{2}\left(\mid{z}_{\mathrm{1}} \mid^{\mathrm{2}} +\mid{z}_{\mathrm{2}} \mid^{\mathrm{2}} \right). \\ $$

Question Number 164462 Answers: 2 Comments: 2

Find x, such that f(x) is minimum. f(x)={((√(c^2 −x^2 ))/(c−x))−(c−x)}^2

$${Find}\:{x},\:{such}\:{that}\:{f}\left({x}\right)\:{is}\:{minimum}. \\ $$$${f}\left({x}\right)=\left\{\frac{\sqrt{{c}^{\mathrm{2}} −{x}^{\mathrm{2}} }}{{c}−{x}}−\left({c}−{x}\right)\right\}^{\mathrm{2}} \\ $$

Question Number 164445 Answers: 0 Comments: 1

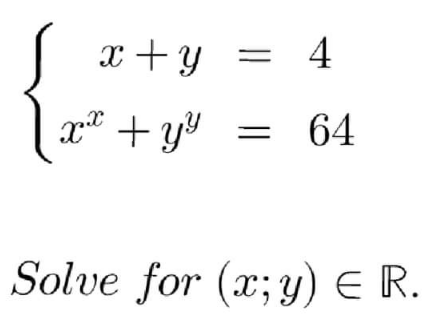
Question Number 164447 Answers: 1 Comments: 0

Question Number 164437 Answers: 1 Comments: 0

Find the max. area of Δle witth sides a,b,c such as 0<a≤1;1≤b≤2;2≤c≤3 is a)1 b)1/2 c)2 d)3/2

$${Find}\:{the}\:{max}.\:{area}\:{of}\:\Delta{le} \\ $$$${witth}\:{sides}\:{a},{b},{c}\:{such}\:{as} \\ $$$$\mathrm{0}<{a}\leqslant\mathrm{1};\mathrm{1}\leqslant{b}\leqslant\mathrm{2};\mathrm{2}\leqslant{c}\leqslant\mathrm{3}\:{is} \\ $$$$\left.{a}\left.\right)\left.\mathrm{1}\left.\:\:\:\:\:\:\:\:{b}\right)\mathrm{1}/\mathrm{2}\:\:\:\:\:\:\:\:\:\:\:\:\:{c}\right)\mathrm{2}\:\:\:\:\:\:\:\:{d}\right)\mathrm{3}/\mathrm{2} \\ $$

Question Number 164434 Answers: 1 Comments: 0

Question Number 164429 Answers: 1 Comments: 0

If C(2x , x) = 70 prove that x = 4

$${If}\:{C}\left(\mathrm{2}{x}\:,\:{x}\right)\:=\:\mathrm{70} \\ $$$${prove}\:{that}\:{x}\:=\:\mathrm{4} \\ $$

Question Number 164426 Answers: 1 Comments: 1

In ΔABC if { ((cot A.cot C = (1/2))),((cot B.cot C = (1/(18)))) :} then tan C = ?

$$\:\:{In}\:\Delta{ABC}\:{if}\:\begin{cases}{\mathrm{cot}\:{A}.\mathrm{cot}\:{C}\:=\:\frac{\mathrm{1}}{\mathrm{2}}}\\{\mathrm{cot}\:{B}.\mathrm{cot}\:{C}\:=\:\frac{\mathrm{1}}{\mathrm{18}}}\end{cases} \\ $$$$\:{then}\:\mathrm{tan}\:\:{C}\:=\:? \\ $$

Question Number 164425 Answers: 1 Comments: 0

What it′s ?? 5×55×555×5555×55555.............∞=

$$\mathrm{What}\:\mathrm{it}'\mathrm{s}\:?? \\ $$$$\mathrm{5}×\mathrm{55}×\mathrm{555}×\mathrm{5555}×\mathrm{55555}.............\infty= \\ $$

Question Number 164419 Answers: 1 Comments: 0

please help me prouve that ∫_0 ^1 ((lnt)/(t^2 −1))dt=(π^2 /8)

$${please}\:{help}\:{me} \\ $$$${prouve}\:{that}\:\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{lnt}}{{t}^{\mathrm{2}} −\mathrm{1}}{dt}=\frac{\pi^{\mathrm{2}} }{\mathrm{8}} \\ $$

Question Number 164418 Answers: 2 Comments: 2

Question Number 164416 Answers: 0 Comments: 0

if a;b;c<0 and a+b+c=3 prove that: (1 + (b/a))^(1/b^2 ) ∙ (1 + (c/b))^(1/c^2 ) ∙ (1 + (a/c))^(1/a^2 ) ≥ 8

$$\mathrm{if}\:\:\mathrm{a};\mathrm{b};\mathrm{c}<\mathrm{0}\:\:\mathrm{and}\:\:\mathrm{a}+\mathrm{b}+\mathrm{c}=\mathrm{3}\:\:\mathrm{prove}\:\mathrm{that}: \\ $$$$\left(\mathrm{1}\:+\:\frac{\mathrm{b}}{\mathrm{a}}\right)^{\frac{\mathrm{1}}{\boldsymbol{\mathrm{b}}^{\mathrm{2}} }} \centerdot\:\left(\mathrm{1}\:+\:\frac{\mathrm{c}}{\mathrm{b}}\right)^{\frac{\mathrm{1}}{\boldsymbol{\mathrm{c}}^{\mathrm{2}} }} \centerdot\:\left(\mathrm{1}\:+\:\frac{\mathrm{a}}{\mathrm{c}}\right)^{\frac{\mathrm{1}}{\boldsymbol{\mathrm{a}}^{\mathrm{2}} }} \geqslant\:\mathrm{8} \\ $$

Question Number 164417 Answers: 1 Comments: 0

Question Number 164405 Answers: 0 Comments: 0

let a, b, c > 0 ; a + b + c = 3 prove that; (a^2 /(b^2 + bc + c^2 )) + (b^2 /(c^2 + ac + a^2 )) + (c^2 /(b^2 +ba + a^2 )) 6 ≥ 2abc + (5/3) (a^2 + b^2 + c^2 ) ≥ 7 ^({Z.A})

$$\boldsymbol{{let}}\:\boldsymbol{{a}},\:\boldsymbol{{b}},\:\boldsymbol{{c}}\:>\:\mathrm{0}\:;\:\boldsymbol{{a}}\:+\:\boldsymbol{{b}}\:+\:\boldsymbol{{c}}\:=\:\mathrm{3} \\ $$$$\boldsymbol{{prove}}\:\boldsymbol{{that}};\:\frac{\boldsymbol{{a}}^{\mathrm{2}} }{\boldsymbol{{b}}^{\mathrm{2}} \:+\:\boldsymbol{{bc}}\:+\:\boldsymbol{{c}}^{\mathrm{2}} }\:\:+\:\:\frac{\boldsymbol{{b}}^{\mathrm{2}} }{\boldsymbol{{c}}^{\mathrm{2}} \:+\:\boldsymbol{{ac}}\:+\:\boldsymbol{{a}}^{\mathrm{2}} \:}\:\:+\:\:\frac{\boldsymbol{{c}}^{\mathrm{2}} }{\boldsymbol{{b}}^{\mathrm{2}} \:+\boldsymbol{{ba}}\:+\:\boldsymbol{{a}}^{\mathrm{2}} }\:\:\mathrm{6}\:\:\geqslant\:\:\mathrm{2}\boldsymbol{{abc}}\:\:+\:\:\frac{\mathrm{5}}{\mathrm{3}}\:\:\left(\boldsymbol{{a}}^{\mathrm{2}} \:+\:\boldsymbol{{b}}^{\mathrm{2}} \:+\:\boldsymbol{{c}}^{\mathrm{2}} \:\right)\:\:\geqslant\:\:\mathrm{7} \\ $$$$\:^{\left\{\boldsymbol{\mathrm{Z}}.\mathrm{A}\right\}} \\ $$

Question Number 164396 Answers: 2 Comments: 1

If f(x)=f(x−1)+x^2 +2x and f(0)=17 , find f(17).

$${If}\:\:{f}\left({x}\right)={f}\left({x}−\mathrm{1}\right)+{x}^{\mathrm{2}} +\mathrm{2}{x} \\ $$$${and}\:\:{f}\left(\mathrm{0}\right)=\mathrm{17}\:\:,\:\:{find}\:{f}\left(\mathrm{17}\right). \\ $$

Question Number 164395 Answers: 2 Comments: 0

Question Number 164391 Answers: 1 Comments: 0

If { ((sin (A−B)=(1/( (√(10)))))),((cos (A+B)=(2/( (√(29)))))) :}; 0<A<(π/4) ; 0<B<(π/4) Find tan 2A.

$$\:\:\mathrm{If}\:\begin{cases}{\mathrm{sin}\:\left(\mathrm{A}−\mathrm{B}\right)=\frac{\mathrm{1}}{\:\sqrt{\mathrm{10}}}}\\{\mathrm{cos}\:\left(\mathrm{A}+\mathrm{B}\right)=\frac{\mathrm{2}}{\:\sqrt{\mathrm{29}}}}\end{cases};\:\mathrm{0}<\mathrm{A}<\frac{\pi}{\mathrm{4}}\:;\:\mathrm{0}<\mathrm{B}<\frac{\pi}{\mathrm{4}} \\ $$$$\:\mathrm{Find}\:\mathrm{tan}\:\mathrm{2A}. \\ $$

Question Number 164386 Answers: 0 Comments: 8

CH_3 −CH_∣_(CH_3 ) −CH=C^∣^(C^(∣CH_3 ) H_2 ) −CH_∣_(CH_∥_(CH_2 ) ) −CH_∣_Δ −CH_∣_(Br) −CH_3 what is the iupac name?

$${CH}_{\mathrm{3}} −{C}\underset{\underset{{CH}_{\mathrm{3}} } {\mid}} {{H}}−{CH}=\overset{\overset{\overset{\mid{CH}_{\mathrm{3}} } {{C}H}_{\mathrm{2}} } {\mid}} {{C}}−{C}\underset{\underset{{C}\underset{\underset{{CH}_{\mathrm{2}} } {\parallel}} {{H}}} {\mid}} {{H}}−{C}\underset{\underset{\Delta} {\mid}} {{H}}−{C}\underset{\underset{{Br}} {\mid}} {{H}}−{CH}_{\mathrm{3}} \:\:\:\: \\ $$$${what}\:{is}\:{the}\:{iupac}\:{name}? \\ $$

Question Number 164496 Answers: 2 Comments: 0

{ ((x^2 + xy = 11)),((y^2 + xy = 24)) :} ⇒ ∣x+y∣=?

$$\begin{cases}{\mathrm{x}^{\mathrm{2}} \:+\:\mathrm{xy}\:=\:\mathrm{11}}\\{\mathrm{y}^{\mathrm{2}} \:+\:\mathrm{xy}\:=\:\mathrm{24}}\end{cases}\:\:\Rightarrow\:\mid\mathrm{x}+\mathrm{y}\mid=? \\ $$

Question Number 164376 Answers: 2 Comments: 0

Question Number 164374 Answers: 0 Comments: 2

Question Number 164372 Answers: 1 Comments: 1

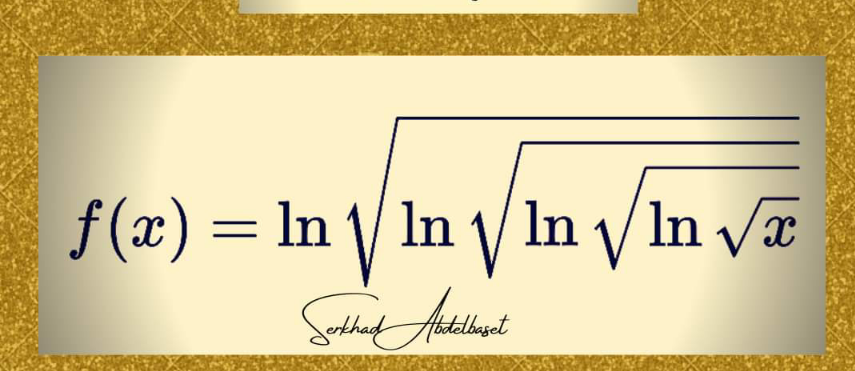
Question Number 164367 Answers: 1 Comments: 0

∫ e^(tan(x)) dx {Z.A}

$$\int\:\boldsymbol{{e}}^{\boldsymbol{{tan}}\left(\boldsymbol{{x}}\right)} \:\boldsymbol{{dx}} \\ $$$$\left\{\boldsymbol{{Z}}.\boldsymbol{{A}}\right\} \\ $$

Question Number 164366 Answers: 1 Comments: 1

(d/dx) (e^(tan(x)) ) {Z.A}

$$\frac{\boldsymbol{{d}}}{\boldsymbol{{dx}}}\:\left(\boldsymbol{{e}}^{\boldsymbol{{tan}}\left(\boldsymbol{{x}}\right)} \right) \\ $$$$\left\{\boldsymbol{{Z}}.\boldsymbol{\mathrm{A}}\right\} \\ $$

Question Number 164364 Answers: 0 Comments: 0

Lim_(x→∞) (e^(tan(x)) ) {Z.A}

$$\boldsymbol{{Lim}}_{\boldsymbol{{x}}\rightarrow\infty} \:\left(\boldsymbol{{e}}^{\boldsymbol{{tan}}\left(\boldsymbol{{x}}\right)} \right) \\ $$$$\left\{\boldsymbol{{Z}}.\boldsymbol{\mathrm{A}}\right\} \\ $$

Question Number 164347 Answers: 0 Comments: 4

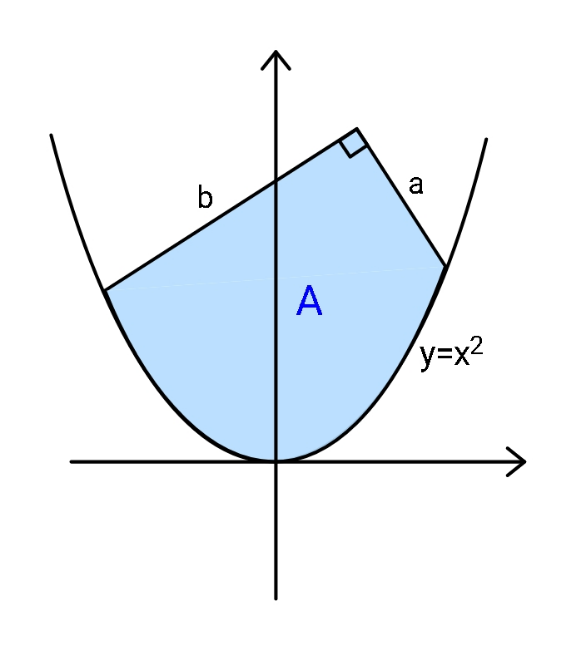
Pg 530 Pg 531 Pg 532 Pg 533 Pg 534 Pg 535 Pg 536 Pg 537 Pg 538 Pg 539

Contact: info@tinkutara.com