
AllQuestion and Answers: Page 533
Question Number 164600 Answers: 3 Comments: 0

Question Number 164599 Answers: 0 Comments: 1
Question Number 164598 Answers: 0 Comments: 0
Question Number 164591 Answers: 1 Comments: 0
Question Number 164590 Answers: 1 Comments: 0
Question Number 164589 Answers: 0 Comments: 0
Question Number 164588 Answers: 0 Comments: 0
Question Number 164585 Answers: 2 Comments: 0
Question Number 164576 Answers: 1 Comments: 0

Question Number 164568 Answers: 2 Comments: 0
$$\:\:\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}−{x}}}\:>\:\frac{\mathrm{1}}{{x}−\mathrm{1}} \\ $$
Question Number 164564 Answers: 0 Comments: 0
Question Number 164560 Answers: 2 Comments: 1
Question Number 164555 Answers: 2 Comments: 0
Question Number 164553 Answers: 3 Comments: 1

Question Number 164549 Answers: 2 Comments: 0

Question Number 164547 Answers: 1 Comments: 0
Question Number 164546 Answers: 1 Comments: 4

Question Number 164544 Answers: 2 Comments: 0
Question Number 164543 Answers: 1 Comments: 0
Question Number 164538 Answers: 0 Comments: 0
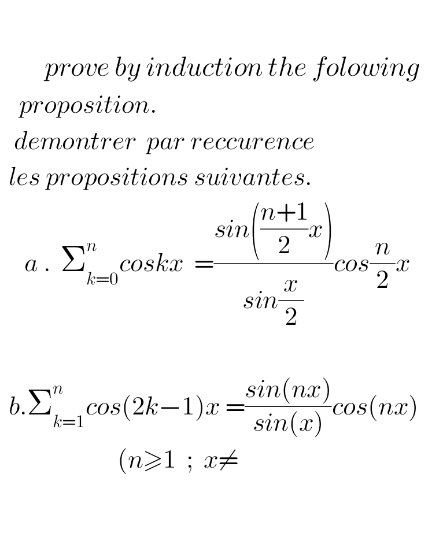
Question Number 164533 Answers: 2 Comments: 0
Question Number 164530 Answers: 1 Comments: 1

Question Number 164524 Answers: 1 Comments: 0

Question Number 164520 Answers: 0 Comments: 0
Question Number 164542 Answers: 0 Comments: 0
Question Number 164515 Answers: 0 Comments: 3
Pg 528 Pg 529 Pg 530 Pg 531 Pg 532 Pg 533 Pg 534 Pg 535 Pg 536 Pg 537
