Question and Answers Forum

AllQuestion and Answers: Page 509

Question Number 161644 Answers: 0 Comments: 2

there is no single greater than symbol the closest to such a symbol is ≪ or ≫ when is tinku tara going to add the single arrow its a very common symbol and should be on this keyboard

$$\: \\ $$$$\:\:\mathrm{there}\:\mathrm{is}\:\mathrm{no}\:\mathrm{single}\:\mathrm{greater}\:\mathrm{than}\:\mathrm{symbol}\:\: \\ $$$$\:\mathrm{the}\:\mathrm{closest}\:\mathrm{to}\:\mathrm{such}\:\mathrm{a}\:\mathrm{symbol}\:\mathrm{is}\:\ll\:\mathrm{or}\:\gg\:\: \\ $$$$\:\mathrm{when}\:\mathrm{is}\:\mathrm{tinku}\:\mathrm{tara}\:\mathrm{going}\:\mathrm{to}\:\mathrm{add}\:\mathrm{the}\:\mathrm{single}\:\mathrm{arrow}\:\: \\ $$$$\:\mathrm{its}\:\mathrm{a}\:\mathrm{very}\:\mathrm{common}\:\mathrm{symbol}\:\mathrm{and}\:\mathrm{should}\:\mathrm{be}\:\mathrm{on}\:\mathrm{this}\:\mathrm{keyboard}\:\: \\ $$$$\: \\ $$$$\: \\ $$

Question Number 161629 Answers: 0 Comments: 0

Question Number 161626 Answers: 3 Comments: 1

Question Number 161668 Answers: 1 Comments: 0

Question Number 161623 Answers: 2 Comments: 0

Σ_(n=1) ^∞ (((−1)^(n+1) )/(n(n+2)))=?

$$\underset{\boldsymbol{{n}}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{\boldsymbol{{n}}+\mathrm{1}} }{\boldsymbol{{n}}\left(\boldsymbol{{n}}+\mathrm{2}\right)}=? \\ $$

Question Number 161622 Answers: 1 Comments: 0

Σ_(n=1) ^∞ (((−1)^(n+1) )/(n(2n+1)))=?

$$\underset{\boldsymbol{{n}}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{\boldsymbol{{n}}+\mathrm{1}} }{\boldsymbol{{n}}\left(\mathrm{2}\boldsymbol{{n}}+\mathrm{1}\right)}=? \\ $$

Question Number 161617 Answers: 2 Comments: 0

Question Number 161612 Answers: 2 Comments: 0

lim_(x→0^+ ) ((cos (√x)))^(1/x) =?

$$\:\:\underset{{x}\rightarrow\mathrm{0}^{+} } {\mathrm{lim}}\:\sqrt[{{x}}]{\mathrm{cos}\:\sqrt{{x}}}\:=? \\ $$

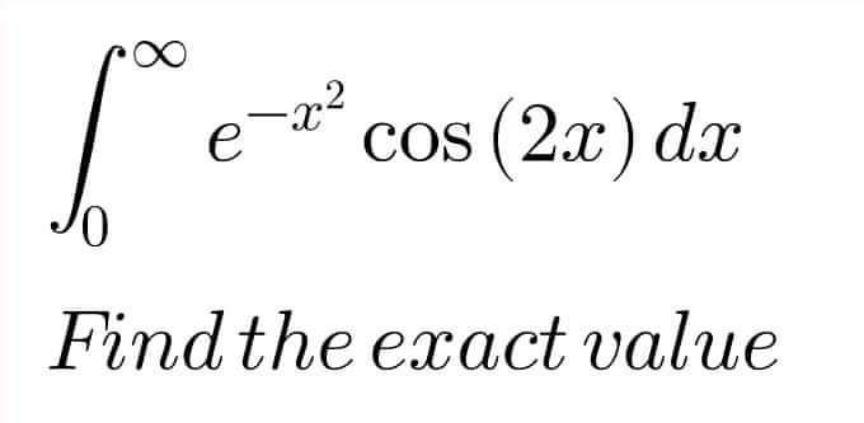
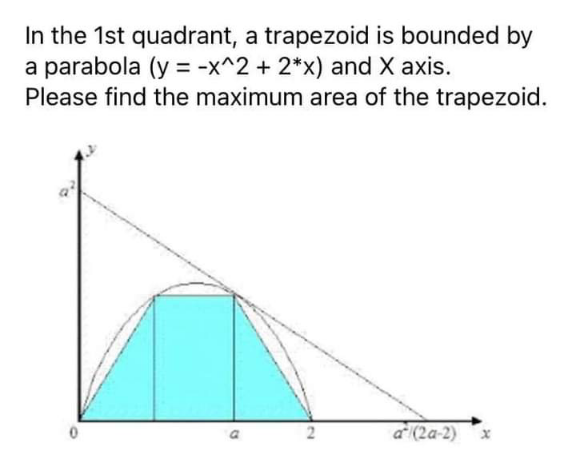
Question Number 161609 Answers: 0 Comments: 0

∫_0 ^1 ((xln(1+x^4 ))/(1+x^2 ))dx=?

$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\boldsymbol{{xln}}\left(\mathrm{1}+\boldsymbol{{x}}^{\mathrm{4}} \right)}{\mathrm{1}+\boldsymbol{{x}}^{\mathrm{2}} }\boldsymbol{{dx}}=? \\ $$$$ \\ $$

Question Number 161590 Answers: 2 Comments: 0

f(x)=(1/(1−(√x))) + (1/(1−(√(1−x)))) f(x)_(min) = ?

$${f}\left({x}\right)=\frac{\mathrm{1}}{\mathrm{1}−\sqrt{{x}}}\:+\:\frac{\mathrm{1}}{\mathrm{1}−\sqrt{\mathrm{1}−\boldsymbol{{x}}}} \\ $$$$\boldsymbol{{f}}\left(\boldsymbol{{x}}\right)_{\boldsymbol{{min}}} =\:? \\ $$

Question Number 161586 Answers: 2 Comments: 2

lim_(x→2^− ) ((2−2cos (√(x−2)))/((x−2)^2 )) =?

$$\:\:\underset{{x}\rightarrow\mathrm{2}^{−} } {\mathrm{lim}}\:\frac{\mathrm{2}−\mathrm{2cos}\:\sqrt{{x}−\mathrm{2}}}{\left({x}−\mathrm{2}\right)^{\mathrm{2}} }\:=? \\ $$

Question Number 161583 Answers: 3 Comments: 1

lim_(x→2) ((1−sin (π/x))/(x^2 −4x+4)) =? lim_(x→2) ((x^3 −8+sin πx)/(2−x))=? lim_(x→1) ((x^2 −1)/(cos ((π/(x+1)))))=?

$$\:\:\underset{{x}\rightarrow\mathrm{2}} {\mathrm{lim}}\:\frac{\mathrm{1}−\mathrm{sin}\:\frac{\pi}{{x}}}{{x}^{\mathrm{2}} −\mathrm{4}{x}+\mathrm{4}}\:=? \\ $$$$\:\underset{{x}\rightarrow\mathrm{2}} {\mathrm{lim}}\:\frac{{x}^{\mathrm{3}} −\mathrm{8}+\mathrm{sin}\:\pi{x}}{\mathrm{2}−{x}}=? \\ $$$$\:\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\:\frac{{x}^{\mathrm{2}} −\mathrm{1}}{\mathrm{cos}\:\left(\frac{\pi}{{x}+\mathrm{1}}\right)}=? \\ $$

Question Number 161578 Answers: 1 Comments: 1

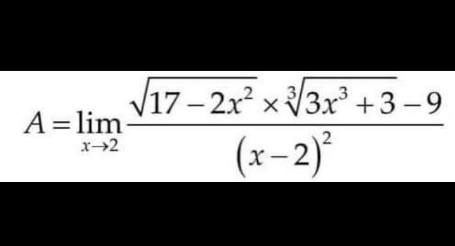
Question Number 161579 Answers: 1 Comments: 1

A gardener wishes to make a rectangular hen run of area 128m² against a wall which is to serve as one of the boundaries. Find the length of the wire netting required for the other three sides

$$ \\ $$A gardener wishes to make a rectangular hen run of area 128m² against a wall which is to serve as one of the boundaries. Find the length of the wire netting required for the other three sides

Question Number 161575 Answers: 1 Comments: 0

∫_0 ^9 ((√x)/(1−x))dx=?

$$\int_{\mathrm{0}} ^{\mathrm{9}} \frac{\sqrt{{x}}}{\mathrm{1}−{x}}{dx}=? \\ $$$$ \\ $$

Question Number 161764 Answers: 2 Comments: 5