Question and Answers Forum

AllQuestion and Answers: Page 505

Question Number 161353 Answers: 0 Comments: 0

Prove that: Σ_(n=0) ^∞ (((-1)^n )/(2n + 1)) ∫_( 0) ^( 1) ∫_( 0) ^( 1) ((dxdy)/((x^2 + y^2 )^n )) = (2/3)

$$\mathrm{Prove}\:\mathrm{that}: \\ $$$$\underset{\boldsymbol{\mathrm{n}}=\mathrm{0}} {\overset{\infty} {\sum}}\:\frac{\left(-\mathrm{1}\right)^{\boldsymbol{\mathrm{n}}} }{\mathrm{2n}\:+\:\mathrm{1}}\:\underset{\:\mathrm{0}} {\overset{\:\mathrm{1}} {\int}}\underset{\:\mathrm{0}} {\overset{\:\mathrm{1}} {\int}}\:\frac{\mathrm{dxdy}}{\left(\mathrm{x}^{\mathrm{2}} \:+\:\mathrm{y}^{\mathrm{2}} \right)^{\boldsymbol{\mathrm{n}}} }\:=\:\frac{\mathrm{2}}{\mathrm{3}} \\ $$

Question Number 161367 Answers: 1 Comments: 1

Question Number 161346 Answers: 0 Comments: 0

Question Number 161342 Answers: 2 Comments: 0

2log _x (3) log _(3x) (3)=log _(9(√x)) (3) x=?

$$\:\:\mathrm{2log}\:_{\mathrm{x}} \left(\mathrm{3}\right)\:\mathrm{log}\:_{\mathrm{3x}} \left(\mathrm{3}\right)=\mathrm{log}\:_{\mathrm{9}\sqrt{\mathrm{x}}} \left(\mathrm{3}\right) \\ $$$$\:\mathrm{x}=? \\ $$

Question Number 161338 Answers: 1 Comments: 1

Question Number 161337 Answers: 0 Comments: 1

(1) lim_(x→0) ((2cos (p+x)−cos (p+2x)−cos p)/x^2 ) ? (2) lim_(x→0) ((tan (2x+q)−2tan (x+q)+tan q)/x^2 ) ?

$$\:\left(\mathrm{1}\right)\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{2cos}\:\left(\mathrm{p}+\mathrm{x}\right)−\mathrm{cos}\:\left(\mathrm{p}+\mathrm{2x}\right)−\mathrm{cos}\:\mathrm{p}}{\mathrm{x}^{\mathrm{2}} }\:? \\ $$$$\:\left(\mathrm{2}\right)\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{tan}\:\left(\mathrm{2x}+\mathrm{q}\right)−\mathrm{2tan}\:\left(\mathrm{x}+\mathrm{q}\right)+\mathrm{tan}\:\mathrm{q}}{\mathrm{x}^{\mathrm{2}} }\:? \\ $$

Question Number 161335 Answers: 0 Comments: 1

Question Number 161331 Answers: 0 Comments: 0

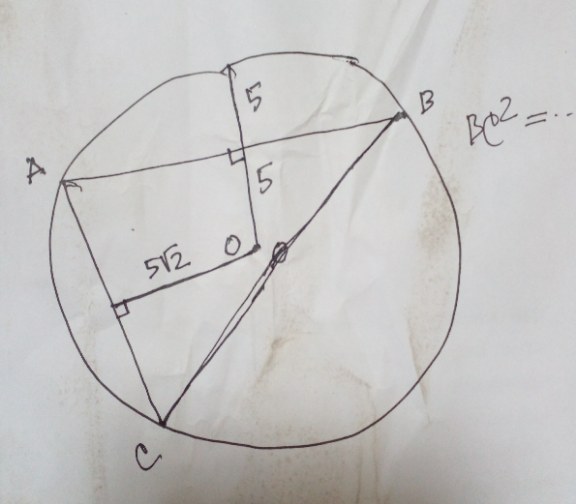
Question Number 161329 Answers: 0 Comments: 0

∫_1 ^( 2) ((tan^(−1) (x−1)log(x))/x)dx

$$\int_{\mathrm{1}} ^{\:\mathrm{2}} \frac{\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{x}−\mathrm{1}\right)\mathrm{log}\left(\mathrm{x}\right)}{\mathrm{x}}\mathrm{dx} \\ $$

Question Number 161326 Answers: 0 Comments: 0

Question Number 161323 Answers: 1 Comments: 3

prove ((( −n )),(( k)) ) =^? (−1)^( k) ((( n +k −1)),(( k)) ) example : ((( −5)),(( 4)) ) = ((( 8)),(( 4)) )

$$ \\ $$$$\:\:\:{prove} \\ $$$$ \\ $$$$\:\:\:\:\begin{pmatrix}{\:\:−{n}\:}\\{\:\:\:\:\:{k}}\end{pmatrix}\:\overset{?} {=}\:\left(−\mathrm{1}\right)^{\:{k}} \:\begin{pmatrix}{\:{n}\:+{k}\:−\mathrm{1}}\\{\:\:\:\:\:\:\:\:\:{k}}\end{pmatrix} \\ $$$$\:\:\:{example}\::\:\:\begin{pmatrix}{\:−\mathrm{5}}\\{\:\:\:\mathrm{4}}\end{pmatrix}\:=\:\begin{pmatrix}{\:\mathrm{8}}\\{\:\:\mathrm{4}}\end{pmatrix} \\ $$$$\:\:\:\:\:\:\: \\ $$

Question Number 161322 Answers: 1 Comments: 4

Question Number 161316 Answers: 1 Comments: 0

Three quarters of a number added to two and a half of that number gives 13. find the number

$$\mathrm{Three}\:\mathrm{quarters}\:\mathrm{of}\:\mathrm{a}\:\mathrm{number}\:\mathrm{added}\:\mathrm{to} \\ $$$$\mathrm{two}\:\mathrm{and}\:\mathrm{a}\:\mathrm{half}\:\mathrm{of}\:\mathrm{that}\:\mathrm{number}\:\mathrm{gives}\: \\ $$$$\mathrm{13}.\:\mathrm{find}\:\mathrm{the}\:\mathrm{number} \\ $$$$ \\ $$

Question Number 161311 Answers: 2 Comments: 0

Differentiate y=sin xy

$${Differentiate}\:{y}=\mathrm{sin}\:{xy} \\ $$

Question Number 161296 Answers: 1 Comments: 0

Question Number 161295 Answers: 0 Comments: 2

prove that:x^8 +x^6 −x^3 −x+1>0,x∈R

$${prove}\:{that}:{x}^{\mathrm{8}} +{x}^{\mathrm{6}} −{x}^{\mathrm{3}} −{x}+\mathrm{1}>\mathrm{0},{x}\in{R} \\ $$

Question Number 161294 Answers: 1 Comments: 0

∫_(−2) ^2 (x^3 cos((x/2))+(1/2))(√(4−x^2 ))dx

$$\int_{−\mathrm{2}} ^{\mathrm{2}} \left(\mathrm{x}^{\mathrm{3}} \mathrm{cos}\left(\frac{\mathrm{x}}{\mathrm{2}}\right)+\frac{\mathrm{1}}{\mathrm{2}}\right)\sqrt{\mathrm{4}−\mathrm{x}^{\mathrm{2}} }\mathrm{dx} \\ $$

Question Number 161285 Answers: 5 Comments: 0

(1) ∫ (dx/(1−2cos x)) (2) ∫ ((sin 2x)/(sin x−sin^2 2x)) dx (3) ∫ (dx/(cos 2x−sin x))

$$\left(\mathrm{1}\right)\:\int\:\frac{{dx}}{\mathrm{1}−\mathrm{2cos}\:{x}} \\ $$$$\left(\mathrm{2}\right)\:\int\:\frac{\mathrm{sin}\:\mathrm{2}{x}}{\mathrm{sin}\:{x}−\mathrm{sin}\:^{\mathrm{2}} \mathrm{2}{x}}\:{dx} \\ $$$$\left(\mathrm{3}\right)\:\int\:\frac{{dx}}{\mathrm{cos}\:\mathrm{2}{x}−\mathrm{sin}\:{x}} \\ $$

Question Number 161284 Answers: 0 Comments: 0

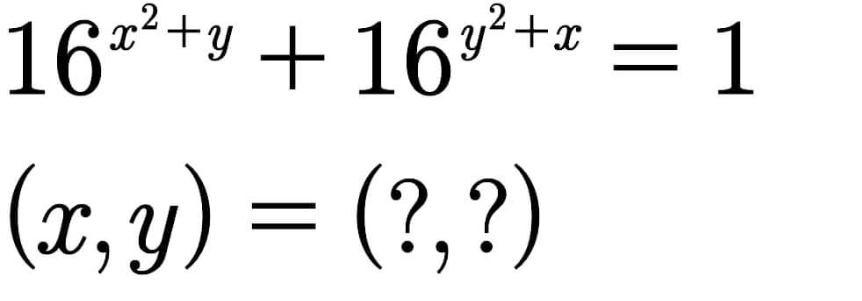
Question Number 161319 Answers: 1 Comments: 0

lim_(x→(π/2)) ((cos x)/( ((sin x+cos x))^(1/3) −sin x))=?

$$\underset{{x}\rightarrow\frac{\pi}{\mathrm{2}}} {\mathrm{lim}}\:\frac{\mathrm{cos}\:{x}}{\:\sqrt[{\mathrm{3}}]{\mathrm{sin}\:{x}+\mathrm{cos}\:{x}}−\mathrm{sin}\:{x}}=? \\ $$

Question Number 161282 Answers: 1 Comments: 0

Question Number 161281 Answers: 0 Comments: 0

Question Number 161280 Answers: 1 Comments: 0

if x;y;z>0 and (1/(1+x)) + (1/(1+y)) + (1/(1+z)) = 1 then prove that: x + y + z ≥ (3/4) xyz

$$\mathrm{if}\:\:\mathrm{x};\mathrm{y};\mathrm{z}>\mathrm{0}\:\:\mathrm{and}\:\:\frac{\mathrm{1}}{\mathrm{1}+\mathrm{x}}\:+\:\frac{\mathrm{1}}{\mathrm{1}+\mathrm{y}}\:+\:\frac{\mathrm{1}}{\mathrm{1}+\mathrm{z}}\:=\:\mathrm{1} \\ $$$$\mathrm{then}\:\mathrm{prove}\:\mathrm{that}: \\ $$$$\mathrm{x}\:+\:\mathrm{y}\:+\:\mathrm{z}\:\geqslant\:\frac{\mathrm{3}}{\mathrm{4}}\:\mathrm{xyz} \\ $$

Question Number 161272 Answers: 0 Comments: 0

Solve the differential systeme (Σ) below: (Σ) { ((x^. (t)=x(t)+2y(t)+t)),((y^. (t)=−4x(t)−3y(t))) :}

$$\mathrm{Solve}\:\mathrm{the}\:\mathrm{differential}\:\mathrm{systeme}\:\left(\Sigma\right)\:\mathrm{below}: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\Sigma\right)\begin{cases}{\overset{.} {{x}}\left({t}\right)={x}\left({t}\right)+\mathrm{2}{y}\left({t}\right)+{t}}\\{\overset{.} {{y}}\left({t}\right)=−\mathrm{4}{x}\left({t}\right)−\mathrm{3}{y}\left({t}\right)}\end{cases}\: \\ $$

Question Number 161265 Answers: 1 Comments: 2

Question Number 161257 Answers: 2 Comments: 1

Pg 500 Pg 501 Pg 502 Pg 503 Pg 504 Pg 505 Pg 506 Pg 507 Pg 508 Pg 509

Contact: info@tinkutara.com