
AllQuestion and Answers: Page 504
Question Number 167725 Answers: 1 Comments: 4
Question Number 167722 Answers: 1 Comments: 1
Question Number 167718 Answers: 0 Comments: 2
Question Number 167716 Answers: 1 Comments: 0

Question Number 167713 Answers: 1 Comments: 0
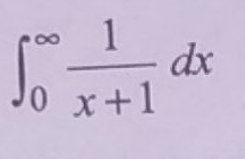
Question Number 167709 Answers: 0 Comments: 0

Question Number 167700 Answers: 1 Comments: 0
Question Number 167699 Answers: 4 Comments: 0

Question Number 167696 Answers: 1 Comments: 0
$${solv}:\:\: \\ $$$${e}^{{x}} +{x}+\mathrm{1}=\mathrm{0} \\ $$
Question Number 167690 Answers: 1 Comments: 0

Question Number 167682 Answers: 1 Comments: 1
$${solve}:\:\mathrm{2}^{{x}} +\mathrm{3}^{{x}} =\mathrm{5}^{{x}} \\ $$
Question Number 167662 Answers: 1 Comments: 0
Question Number 167666 Answers: 1 Comments: 1
Question Number 167664 Answers: 0 Comments: 0
Question Number 167663 Answers: 1 Comments: 2
Question Number 167650 Answers: 1 Comments: 4
Question Number 167648 Answers: 0 Comments: 6
Question Number 167647 Answers: 2 Comments: 0
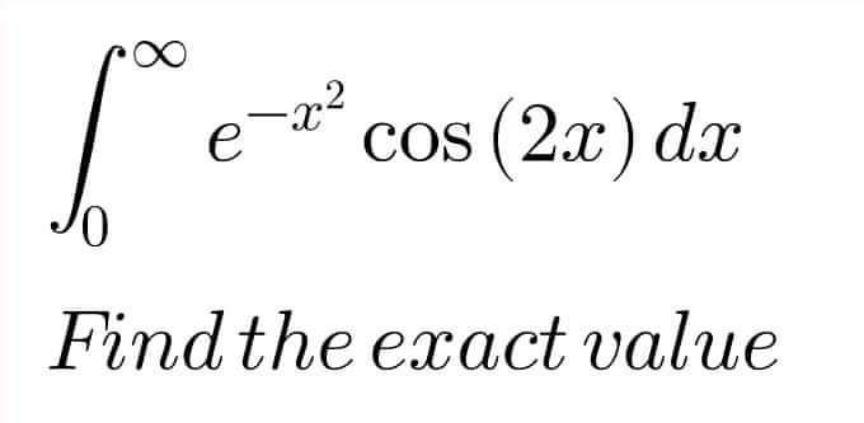
Question Number 167626 Answers: 0 Comments: 0
Question Number 167624 Answers: 2 Comments: 0
Question Number 167633 Answers: 1 Comments: 6

Question Number 167630 Answers: 0 Comments: 0
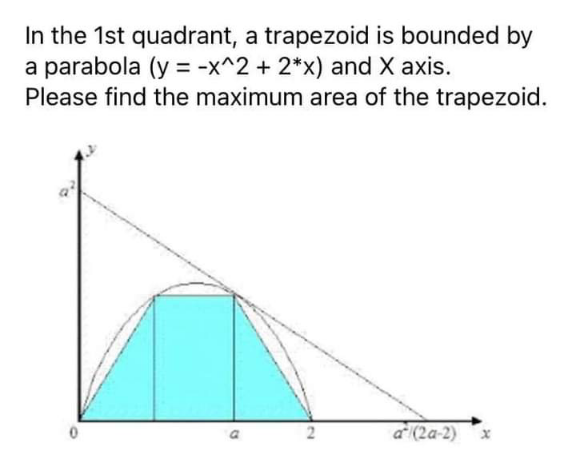
Question Number 167617 Answers: 3 Comments: 0
Question Number 167616 Answers: 0 Comments: 0
Question Number 167615 Answers: 0 Comments: 0
Question Number 167612 Answers: 0 Comments: 1

Pg 499 Pg 500 Pg 501 Pg 502 Pg 503 Pg 504 Pg 505 Pg 506 Pg 507 Pg 508
