Question and Answers Forum

AllQuestion and Answers: Page 503

Question Number 168147 Answers: 0 Comments: 0

calculate :: lim_(x→0) (((tan (1+tan x))/(tan (1+sin x))))^(1/x^3 ) =?

$$\mathrm{calculate}\:\:::\:\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\sqrt[{\mathrm{x}^{\mathrm{3}} }]{\frac{\mathrm{tan}\:\left(\mathrm{1}+\mathrm{tan}\:\mathrm{x}\right)}{\mathrm{tan}\:\left(\mathrm{1}+\mathrm{sin}\:\mathrm{x}\right)}}=? \\ $$

Question Number 168144 Answers: 1 Comments: 0

lim_(x→0) ((x^3 (√(1+x)) −sin^3 x −(1/2)x^3 tan x)/((((1+2x^3 ))^(1/3) −1)ln (1+x^2 )))=?

$$\:\:\:\:\:\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{x}^{\mathrm{3}} \sqrt{\mathrm{1}+{x}}\:−\mathrm{sin}\:^{\mathrm{3}} {x}\:−\frac{\mathrm{1}}{\mathrm{2}}{x}^{\mathrm{3}} \:\mathrm{tan}\:{x}}{\left(\sqrt[{\mathrm{3}}]{\mathrm{1}+\mathrm{2}{x}^{\mathrm{3}} }−\mathrm{1}\right)\mathrm{ln}\:\left(\mathrm{1}+{x}^{\mathrm{2}} \right)}=?\:\:\:\:\:\:\:\: \\ $$

Question Number 168143 Answers: 2 Comments: 0

lim_(x→0) ((1−cos (1−cos x))/x^4 ) =?

$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{1}−\mathrm{cos}\:\left(\mathrm{1}−\mathrm{cos}\:{x}\right)}{{x}^{\mathrm{4}} }\:=?\:\:\:\:\:\: \\ $$

Question Number 168138 Answers: 2 Comments: 0

Question Number 168131 Answers: 2 Comments: 1

Question Number 168121 Answers: 1 Comments: 0

Question Number 168119 Answers: 1 Comments: 0

Question Number 168118 Answers: 1 Comments: 0

If tan(θ+iφ)=cosα+isinα, prove that : θ=((nΠ)/2)+(Π/4) and φ=(1/2)log tan((Π/4)+(α/2))

$${If}\:{tan}\left(\theta+{i}\phi\right)={cos}\alpha+{isin}\alpha,\: \\ $$$${prove}\:{that}\::\:\theta=\frac{{n}\Pi}{\mathrm{2}}+\frac{\Pi}{\mathrm{4}}\:{and}\:\phi=\frac{\mathrm{1}}{\mathrm{2}}{log}\:{tan}\left(\frac{\Pi}{\mathrm{4}}+\frac{\alpha}{\mathrm{2}}\right) \\ $$

Question Number 168115 Answers: 0 Comments: 2

Question Number 168114 Answers: 2 Comments: 0

Question Number 168094 Answers: 1 Comments: 0

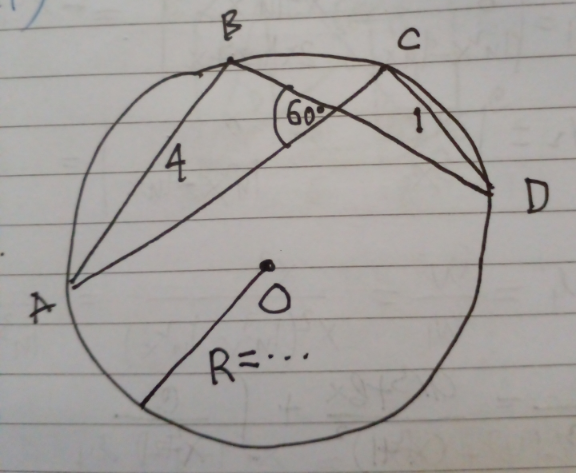
Question Number 168093 Answers: 1 Comments: 0

Question Number 168092 Answers: 0 Comments: 0

Question Number 168091 Answers: 0 Comments: 0

Question Number 168090 Answers: 0 Comments: 0

Question Number 168086 Answers: 1 Comments: 0

Question Number 168085 Answers: 0 Comments: 1

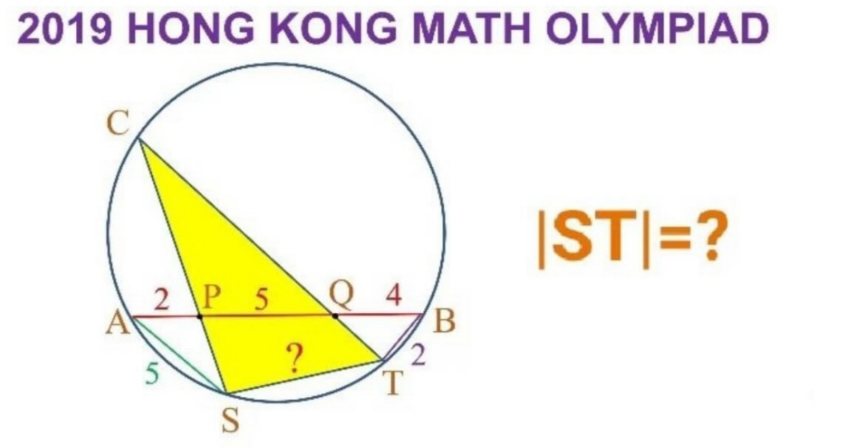
Question Number 168084 Answers: 3 Comments: 3

solve in R arcsin(x)+arcsin((√(1−x^2 )))=(π/2)

$${solve}\:{in}\:\mathbb{R} \\ $$$${arcsin}\left({x}\right)+{arcsin}\left(\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }\right)=\frac{\pi}{\mathrm{2}} \\ $$

Question Number 168081 Answers: 0 Comments: 0

A cook puts 9.00 g of water in a 2.00L pressure cooker that is then warmed to 500 degree C. What is the pressure inside the container A. 1.89 times 106Pa B. 1.89times 105Pa C. 1.99 times 104Pa D. 1.89 times 103P a E. 1.99 times 109Pa

$$ \\ $$$$\mathrm{A}\:\mathrm{cook}\:\mathrm{puts}\:\mathrm{9}.\mathrm{00}\:\mathrm{g}\:\mathrm{of}\:\mathrm{water}\:\mathrm{in}\:\mathrm{a}\:\mathrm{2}.\mathrm{00L}\:\mathrm{pressure}\:\mathrm{cooker}\: \\ $$$$\mathrm{th}{a}\mathrm{t}\:\mathrm{is}\:\mathrm{then}\:\mathrm{warmed}\:\mathrm{to}\:\mathrm{500}\:\mathrm{degree}\:\mathrm{C}.\:\mathrm{What}\:\mathrm{is}\:\mathrm{the} \\ $$$$\mathrm{pressur}{e}\:\mathrm{inside}\:\mathrm{the}\:\mathrm{container}\:\mathrm{A}.\:\mathrm{1}.\mathrm{89}\:\mathrm{times}\:\mathrm{106Pa}\:\mathrm{B}.\: \\ $$$$\mathrm{1}.\mathrm{89times}\:\mathrm{105Pa}\:\mathrm{C}.\:\mathrm{1}.\mathrm{99}\:\mathrm{times}\:\mathrm{104Pa}\:\mathrm{D}.\:\mathrm{1}.\mathrm{89}\:\mathrm{times}\:\mathrm{103P} \\ $$$$\mathrm{a}\:\mathrm{E}.\:\mathrm{1}.\mathrm{99}\:\mathrm{times}\:\mathrm{109Pa} \\ $$

Question Number 168102 Answers: 0 Comments: 0

solve the differential equation sin^3 x y′′+sinxcosx y′+4y=0

$${solve}\:{the}\:{differential}\:{equation} \\ $$$${sin}^{\mathrm{3}} {x}\:{y}''+{sinxcosx}\:{y}'+\mathrm{4}{y}=\mathrm{0} \\ $$

Question Number 168103 Answers: 2 Comments: 4

Question Number 168073 Answers: 1 Comments: 0

The unit digit of a two degit number is 1 less than the tens degit. if the number is increased by 8 and then divided by the sum of the digits, the result is 8. Find the number.

$$\mathrm{The}\:\mathrm{unit}\:\mathrm{digit}\:\mathrm{of}\:\mathrm{a}\:\mathrm{two}\:\mathrm{degit}\:\mathrm{number}\:\mathrm{is}\:\mathrm{1}\:\mathrm{less} \\ $$$$\mathrm{than}\:\mathrm{the}\:\mathrm{tens}\:\mathrm{degit}.\:\mathrm{if}\:\mathrm{the}\:\mathrm{number}\:\mathrm{is}\:\mathrm{increased} \\ $$$$\mathrm{by}\:\mathrm{8}\:\mathrm{and}\:\mathrm{then}\:\mathrm{divided}\:\mathrm{by}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{digits},\:\mathrm{the}\:\mathrm{result}\:\mathrm{is}\:\mathrm{8}.\:\mathrm{Find}\:\mathrm{the}\:\mathrm{number}. \\ $$

Question Number 168072 Answers: 2 Comments: 0

The ratio of cars to motorcycles sold in a garage is 5:7. If a dealer sold 142 more motorcycles than cars in a particular month, find the number of each type of vehicle sold.

$$\mathrm{The}\:\mathrm{ratio}\:\mathrm{of}\:\mathrm{cars}\:\mathrm{to}\:\mathrm{motorcycles}\:\mathrm{sold}\:\mathrm{in}\:\mathrm{a} \\ $$$$\mathrm{garage}\:\mathrm{is}\:\mathrm{5}:\mathrm{7}.\:\mathrm{If}\:\mathrm{a}\:\mathrm{dealer}\:\mathrm{sold}\:\mathrm{142}\:\mathrm{more}\: \\ $$$$\mathrm{motorcycles}\:\mathrm{than}\:\mathrm{cars}\:\mathrm{in}\:\mathrm{a}\:\mathrm{particular}\:\mathrm{month}, \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{each}\:\mathrm{type}\:\mathrm{of}\:\mathrm{vehicle} \\ $$$$\mathrm{sold}. \\ $$

Question Number 168071 Answers: 0 Comments: 0

A clean tube of diameter 2.5 mm is imersed in a liquidi wth a coefficient of surface tension 0.4 nm. The anglef o contact of the liquid with the glass can be assumed as1 35^0 . The density of liquid 13600 kgm3. What would bethe level of the liquid in the tube relative to the freeu srface of the liuid inside the tube Answer should be inm m

$$ \\ $$$$\mathrm{A}\:\mathrm{clean}\:\mathrm{tube}\:\mathrm{of}\:\mathrm{diameter}\:\mathrm{2}.\mathrm{5}\:\mathrm{mm}\:\mathrm{is}\:\mathrm{imersed}\:\mathrm{in}\:\mathrm{a}\:\mathrm{liquidi} \\ $$$$\mathrm{wth}\:\mathrm{a}\:\mathrm{coefficient}\:\mathrm{of}\:\mathrm{surface}\:\mathrm{tension}\:\:\mathrm{0}.\mathrm{4}\:\mathrm{nm}.\:\mathrm{The}\:\mathrm{anglef} \\ $$$$\mathrm{o}\:\mathrm{contact}\:\mathrm{of}\:\mathrm{the}\:\mathrm{liquid}\:\mathrm{with}\:\mathrm{the}\:\mathrm{glass}\:\mathrm{can}\:\mathrm{be}\:\mathrm{assumed}\:\mathrm{as1} \\ $$$$\mathrm{35}^{\mathrm{0}} .\:\mathrm{The}\:\mathrm{density}\:\mathrm{of}\:\mathrm{liquid}\:\mathrm{13600}\:\mathrm{kgm3}.\:\mathrm{What}\:\mathrm{would}\: \\ $$$$\mathrm{bethe}\:\mathrm{level}\:\mathrm{of}\:\mathrm{the}\:\mathrm{liquid}\:\mathrm{in}\:\mathrm{the}\:\mathrm{tube}\:\mathrm{relative}\:\mathrm{to}\:\mathrm{the}\:\mathrm{freeu} \\ $$$$\mathrm{srface}\:\mathrm{of}\:\mathrm{the}\:\mathrm{liuid}\:\mathrm{inside}\:\mathrm{the}\:\mathrm{tube}\:\mathrm{Answer}\:\mathrm{should}\:\mathrm{be}\:\mathrm{inm} \\ $$$$\mathrm{m} \\ $$

Question Number 168064 Answers: 0 Comments: 5

q=(m/( (√p)))+(p^2 /m) make p the subject

$$\:{q}=\frac{{m}}{\:\sqrt{{p}}}+\frac{{p}^{\mathrm{2}} }{{m}} \\ $$$$\:{make}\:\:{p}\:\:{the}\:{subject} \\ $$

Question Number 168055 Answers: 2 Comments: 0

Calculate :: lim_(x→0) (((1+x)^(−(1/x^3 )) )/x)=?

$$\mathrm{Calculate}\:::\:\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\left(\mathrm{1}+\mathrm{x}\right)^{−\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{3}} }} }{\mathrm{x}}=? \\ $$

Pg 498 Pg 499 Pg 500 Pg 501 Pg 502 Pg 503 Pg 504 Pg 505 Pg 506 Pg 507

Contact: info@tinkutara.com