
AllQuestion and Answers: Page 502
Question Number 168341 Answers: 1 Comments: 0
$$\int\left(\mathrm{1}+{x}^{\mathrm{2}} \right)^{\mathrm{3}} {dx}=? \\ $$
Question Number 168339 Answers: 0 Comments: 0
Question Number 168338 Answers: 1 Comments: 0
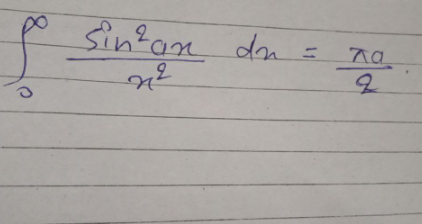
Question Number 168335 Answers: 2 Comments: 0
$$\frac{{x}}{\mathrm{2}}+\mathrm{4}\sqrt{{x}\:}+\mathrm{6}+\mathrm{3}+\mathrm{1}={x} \\ $$
Question Number 168325 Answers: 0 Comments: 0
Question Number 168324 Answers: 1 Comments: 0

Question Number 168320 Answers: 0 Comments: 0
Question Number 168311 Answers: 0 Comments: 5
Question Number 168303 Answers: 2 Comments: 0
$${calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} {x}\sqrt{\mathrm{1}−{x}^{\mathrm{6}} }{dx} \\ $$
Question Number 168297 Answers: 1 Comments: 0
Question Number 168296 Answers: 2 Comments: 0
$$\int\left(\mathrm{5}{x}+\mathrm{2}\right){cos}\left(\mathrm{2}{x}\right){dx}=? \\ $$
Question Number 168291 Answers: 2 Comments: 0
$$\int{t}^{\mathrm{7}} \mathrm{sin}\left({t}^{\mathrm{7}} \right){dt} \\ $$
Question Number 168280 Answers: 1 Comments: 0
Question Number 168278 Answers: 1 Comments: 2
Question Number 168277 Answers: 0 Comments: 1
Question Number 168276 Answers: 3 Comments: 2
$$\:\:\:\:{y}\:=\:\sqrt{{x}+\sqrt{{x}+\sqrt{{x}}}} \\ $$$$\:\:\:\:{y}'\:=\: \\ $$$$\:\:\:\:\: \\ $$
Question Number 168274 Answers: 1 Comments: 0

Question Number 168267 Answers: 1 Comments: 0
Question Number 168264 Answers: 1 Comments: 0

Question Number 168259 Answers: 1 Comments: 0
Question Number 168256 Answers: 1 Comments: 0
$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\frac{{n}!}{{n}^{{n}} }=? \\ $$
Question Number 168248 Answers: 0 Comments: 1
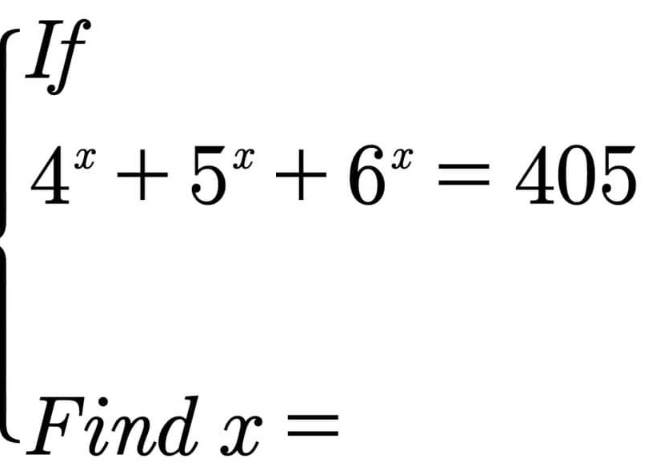
Question Number 168247 Answers: 2 Comments: 0
Question Number 168244 Answers: 3 Comments: 0

Question Number 168233 Answers: 1 Comments: 0
Question Number 168231 Answers: 0 Comments: 4
$$\int\:\:{x}\:\sqrt{\mathrm{1}−{x}^{\mathrm{6}} }\:\:{dx}\:\:=\:\:? \\ $$
Pg 497 Pg 498 Pg 499 Pg 500 Pg 501 Pg 502 Pg 503 Pg 504 Pg 505 Pg 506
