Question and Answers Forum

AllQuestion and Answers: Page 501

Question Number 167985 Answers: 2 Comments: 0

If af(x)+bf((1/x))=(1/x) where a≠b and x≠0 show that f(x)=((1/(a^2 −b^2 )))((a/x)−bx)

$$\mathrm{If}\:\:\mathrm{af}\left(\mathrm{x}\right)+\mathrm{bf}\left(\frac{\mathrm{1}}{\mathrm{x}}\right)=\frac{\mathrm{1}}{\mathrm{x}}\:\mathrm{where}\: \\ $$$$\mathrm{a}\neq\mathrm{b}\:\mathrm{and}\:\:\mathrm{x}\neq\mathrm{0}\:\mathrm{show}\:\mathrm{that} \\ $$$$\boldsymbol{\mathrm{f}}\left(\boldsymbol{\mathrm{x}}\right)=\left(\frac{\mathrm{1}}{\boldsymbol{\mathrm{a}}^{\mathrm{2}} −\boldsymbol{\mathrm{b}}^{\mathrm{2}} }\right)\left(\frac{\boldsymbol{\mathrm{a}}}{\boldsymbol{\mathrm{x}}}−\boldsymbol{\mathrm{bx}}\right) \\ $$

Question Number 167983 Answers: 0 Comments: 0

lim_(x→2^x ) (((x+2)/(2−x)))=?

$$\underset{{x}\rightarrow\mathrm{2}^{{x}} } {\mathrm{lim}}\left(\frac{{x}+\mathrm{2}}{\mathrm{2}−{x}}\right)=? \\ $$

Question Number 167982 Answers: 0 Comments: 3

lim_(x→∞) (((√(4x^2 −4x+1))+3x)/( (√(x^2 +x−5))+x))=?

$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\frac{\sqrt{\mathrm{4}{x}^{\mathrm{2}} −\mathrm{4}{x}+\mathrm{1}}+\mathrm{3}{x}}{\:\sqrt{{x}^{\mathrm{2}} +{x}−\mathrm{5}}+{x}}=? \\ $$

Question Number 167981 Answers: 1 Comments: 0

lim_(x→0) ((4^x −2^x )/(8^x −4^x ))=?

$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{4}^{{x}} −\mathrm{2}^{{x}} }{\mathrm{8}^{{x}} −\mathrm{4}^{{x}} }=? \\ $$

Question Number 167980 Answers: 1 Comments: 3

lim_(x→∞) (√(4x^2 −16x+1))−2x+3=?

$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\sqrt{\mathrm{4}{x}^{\mathrm{2}} −\mathrm{16}{x}+\mathrm{1}}−\mathrm{2}{x}+\mathrm{3}=? \\ $$

Question Number 167979 Answers: 1 Comments: 0

3 men and 4 women are to sit on a table. Calculate the number of possible sitting arrangements if (a) they sit in a row such that the men must not sit next to each other. (b) they sit in circular pattern and the clockwise and anticlockwise orders are considered the same.

$$\mathrm{3}\:\mathrm{men}\:\mathrm{and}\:\mathrm{4}\:\mathrm{women}\:\mathrm{are}\:\mathrm{to}\:\mathrm{sit}\:\mathrm{on}\:\mathrm{a} \\ $$$$\mathrm{table}.\:\mathrm{Calculate}\:\mathrm{the}\:\mathrm{number}\:\mathrm{of} \\ $$$$\mathrm{possible}\:\mathrm{sitting}\:\mathrm{arrangements}\:\mathrm{if} \\ $$$$\:\left({a}\right)\:\mathrm{they}\:\mathrm{sit}\:\mathrm{in}\:\mathrm{a}\:\mathrm{row}\:\mathrm{such}\:\mathrm{that}\:\mathrm{the} \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{men}\:\mathrm{must}\:\mathrm{not}\:\mathrm{sit}\:\mathrm{next}\:\mathrm{to}\:\mathrm{each} \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{other}. \\ $$$$\:\left({b}\right)\:\mathrm{they}\:\mathrm{sit}\:\mathrm{in}\:\mathrm{circular}\:\mathrm{pattern}\:\mathrm{and} \\ $$$$\:\:\:\:\:\:\:\:\mathrm{the}\:\mathrm{clockwise}\:\mathrm{and}\:\mathrm{anticlockwise} \\ $$$$\:\:\:\:\:\:\:\:\mathrm{orders}\:\mathrm{are}\:\mathrm{considered}\:\mathrm{the}\:\mathrm{same}. \\ $$

Question Number 167978 Answers: 0 Comments: 0

(((3 2 )),((4 5)) ) [A] = determinant (((3 2)),((4 5)))

$$\begin{pmatrix}{\mathrm{3}\:\:\:\:\:\mathrm{2}\:}\\{\mathrm{4}\:\:\:\:\:\:\mathrm{5}}\end{pmatrix}\:\:\left[{A}\right]\:=\begin{vmatrix}{\mathrm{3}\:\:\:\:\:\:\mathrm{2}}\\{\mathrm{4}\:\:\:\:\:\:\mathrm{5}}\end{vmatrix} \\ $$

Question Number 167977 Answers: 1 Comments: 0

Prove that I_n =(1/2^(n+1) )∫_π ^(4nπ) xcos (x/2)dx=((2−π)/2^(np) )

$${Prove}\:{that} \\ $$$${I}_{{n}} =\frac{\mathrm{1}}{\mathrm{2}^{{n}+\mathrm{1}} }\int_{\pi} ^{\mathrm{4}{n}\pi} {x}\mathrm{cos}\:\frac{{x}}{\mathrm{2}}{dx}=\frac{\mathrm{2}−\pi}{\mathrm{2}^{{np}} } \\ $$

Question Number 167976 Answers: 0 Comments: 0

Question Number 167974 Answers: 1 Comments: 0

Question Number 167970 Answers: 0 Comments: 0

Calculate: lim_(n→∞) Σ_(k=1) ^n (k^2 /n^3 )∙(k)^(1/k) =?

$$\mathrm{Calculate}:\:\:\:\underset{\mathrm{n}\rightarrow\infty} {\mathrm{lim}}\underset{\mathrm{k}=\mathrm{1}} {\overset{\mathrm{n}} {\sum}}\frac{\mathrm{k}^{\mathrm{2}} }{\mathrm{n}^{\mathrm{3}} }\centerdot\sqrt[{\mathrm{k}}]{\mathrm{k}}=? \\ $$

Question Number 167966 Answers: 1 Comments: 0

Question Number 167965 Answers: 1 Comments: 0

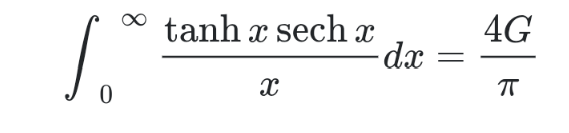
Question Number 167964 Answers: 1 Comments: 1

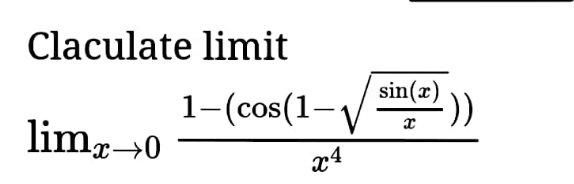
Question Number 167963 Answers: 0 Comments: 0

show that_β_(1 =( nΣxy−ΣxΣy)/(nΣx^2 −(Σx)^2 )=Σxy/Σ(xy)^(2 ) where x=(x−x^− ) and y=(y−y^− ) )

$$\:{show}\:{that}_{\beta_{\mathrm{1}\:=\left(\:{n}\Sigma{xy}−\Sigma{x}\Sigma{y}\right)/\left({n}\Sigma{x}^{\mathrm{2}} −\left(\Sigma{x}\right)^{\mathrm{2}} \right)=\Sigma{xy}/\Sigma\left({xy}\right)^{\mathrm{2}\:} \:\:\:\:\:\:\:{where}\:{x}=\left({x}−\overset{−} {{x}}\right)\:{and}\:{y}=\left({y}−\overset{−} {{y}}\right)\:\:} } \: \\ $$$$ \\ $$

Question Number 167956 Answers: 0 Comments: 0

Question Number 167955 Answers: 2 Comments: 0

Question Number 167943 Answers: 2 Comments: 3

lim_(x→3) ((e^x −e^3 )/(x−3))=? wiht out H,pital ruls

$$\underset{{x}\rightarrow\mathrm{3}} {\mathrm{lim}}\frac{{e}^{{x}} −{e}^{\mathrm{3}} }{{x}−\mathrm{3}}=? \\ $$$${wiht}\:{out}\:{H},{pital}\:{ruls} \\ $$

Question Number 167942 Answers: 1 Comments: 0

(x−1)(x+4)(x−2)^2 =10x^2 x=???

$$\left({x}−\mathrm{1}\right)\left({x}+\mathrm{4}\right)\left({x}−\mathrm{2}\right)^{\mathrm{2}} =\mathrm{10}{x}^{\mathrm{2}} \\ $$$${x}=??? \\ $$

Question Number 167939 Answers: 0 Comments: 0

find the root of f(x)=x^3 −5^x +3 using newton raphson iteration method

$${find}\:{the}\:{root}\:{of}\: \\ $$$${f}\left({x}\right)={x}^{\mathrm{3}} −\mathrm{5}^{{x}} +\mathrm{3} \\ $$$${using}\:{newton}\:{raphson}\:{iteration}\:{method} \\ $$

Question Number 190928 Answers: 0 Comments: 6

Solve the equation : 2+x^2 (x^4 +1)=(((√2)x^3 (x^4 −1))/( (√(x^4 +1))))

$${Solve}\:{the}\:{equation}\:: \\ $$$$\mathrm{2}+{x}^{\mathrm{2}} \left({x}^{\mathrm{4}} +\mathrm{1}\right)=\frac{\sqrt{\mathrm{2}}{x}^{\mathrm{3}} \left({x}^{\mathrm{4}} −\mathrm{1}\right)}{\:\sqrt{{x}^{\mathrm{4}} +\mathrm{1}}} \\ $$$$ \\ $$

Question Number 167930 Answers: 1 Comments: 1

y^(′′) − y^′ − 6y = xe^x sinx

$$\boldsymbol{{y}}\:^{''} \:−\:\boldsymbol{{y}}^{'} \:−\:\mathrm{6}\boldsymbol{{y}}\:=\:\boldsymbol{{xe}}^{\boldsymbol{{x}}} \:\boldsymbol{{sinx}} \\ $$

Question Number 167929 Answers: 1 Comments: 0

prove: (((a^2 +b^2 )sinαcosα−ab)/((a^2 +b^2 )cos^2 α−b^2 ))=((asinα−bcosα)/(acosα+bsinα))

$${prove}:\: \\ $$$$\:\:\:\:\:\:\:\frac{\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)\mathrm{sin}\alpha\mathrm{cos}\alpha−{ab}}{\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} \right)\mathrm{cos}^{\mathrm{2}} \alpha−{b}^{\mathrm{2}} }=\frac{{a}\mathrm{sin}\alpha−{b}\mathrm{cos}\alpha}{{a}\mathrm{cos}\alpha+{b}\mathrm{sin}\alpha} \\ $$

Question Number 167928 Answers: 1 Comments: 0

Show that ∣1−i∣^x =2^x has no nonzero integral solution

$${Show}\:{that}\:\mid\mathrm{1}−{i}\mid^{{x}} =\mathrm{2}^{{x}} \:{has}\:{no}\:{nonzero}\:{integral}\:{solution}\: \\ $$

Question Number 167925 Answers: 1 Comments: 0

Given f(x) = ax^5 + bx^4 + cx^3 + dx^2 + ex + f . f(1) = 1 , f(2) = (1/4) , f(3) = (1/9) , f(4) = (1/(16)) , f(5) = (1/(25)) , and f(6) = (1/(36)) . Value of f(8) = ?

$$\mathrm{Given}\:\:{f}\left({x}\right)\:=\:{ax}^{\mathrm{5}} \:+\:{bx}^{\mathrm{4}} \:+\:{cx}^{\mathrm{3}} \:+\:{dx}^{\mathrm{2}} \:+\:{ex}\:+\:{f}\:. \\ $$$${f}\left(\mathrm{1}\right)\:=\:\mathrm{1}\:,\:{f}\left(\mathrm{2}\right)\:=\:\frac{\mathrm{1}}{\mathrm{4}}\:\:,\:\:{f}\left(\mathrm{3}\right)\:=\:\frac{\mathrm{1}}{\mathrm{9}}\:\:,\:\:{f}\left(\mathrm{4}\right)\:=\:\frac{\mathrm{1}}{\mathrm{16}}\:\:, \\ $$$${f}\left(\mathrm{5}\right)\:=\:\frac{\mathrm{1}}{\mathrm{25}}\:\:,\:\:{and}\:\:{f}\left(\mathrm{6}\right)\:=\:\frac{\mathrm{1}}{\mathrm{36}}\:. \\ $$$${Value}\:\:{of}\:\:{f}\left(\mathrm{8}\right)\:=\:? \\ $$

Question Number 167922 Answers: 4 Comments: 2

Find the value of Q if ∫_0 ^Q (√(cosec θ−1)) dθ=ln (((3+2(√2))/2))

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\:\mathrm{Q}\:\:\mathrm{if} \\ $$$$\int_{\mathrm{0}} ^{\mathrm{Q}} \sqrt{\mathrm{cosec}\:\theta−\mathrm{1}}\:\mathrm{d}\theta=\mathrm{ln}\:\left(\frac{\mathrm{3}+\mathrm{2}\sqrt{\mathrm{2}}}{\mathrm{2}}\right) \\ $$$$ \\ $$

Pg 496 Pg 497 Pg 498 Pg 499 Pg 500 Pg 501 Pg 502 Pg 503 Pg 504 Pg 505

Contact: info@tinkutara.com