
AllQuestion and Answers: Page 500
Question Number 168480 Answers: 1 Comments: 4
Question Number 168473 Answers: 1 Comments: 0
Question Number 168471 Answers: 1 Comments: 1

Question Number 168464 Answers: 1 Comments: 1
Question Number 168463 Answers: 0 Comments: 0
Question Number 168459 Answers: 2 Comments: 0

Question Number 168458 Answers: 0 Comments: 1
Question Number 168461 Answers: 0 Comments: 1
Question Number 168443 Answers: 0 Comments: 0

Question Number 168442 Answers: 0 Comments: 0

Question Number 168441 Answers: 1 Comments: 0
Question Number 168438 Answers: 0 Comments: 0
Question Number 168436 Answers: 0 Comments: 0
Question Number 168435 Answers: 0 Comments: 0
Question Number 168434 Answers: 3 Comments: 0
$$\mathrm{2}^{{x}} ×\mathrm{3}^{\frac{\mathrm{4}}{{x}}} =\mathrm{48} \\ $$$${x}=? \\ $$
Question Number 168429 Answers: 2 Comments: 0
Question Number 168446 Answers: 1 Comments: 0
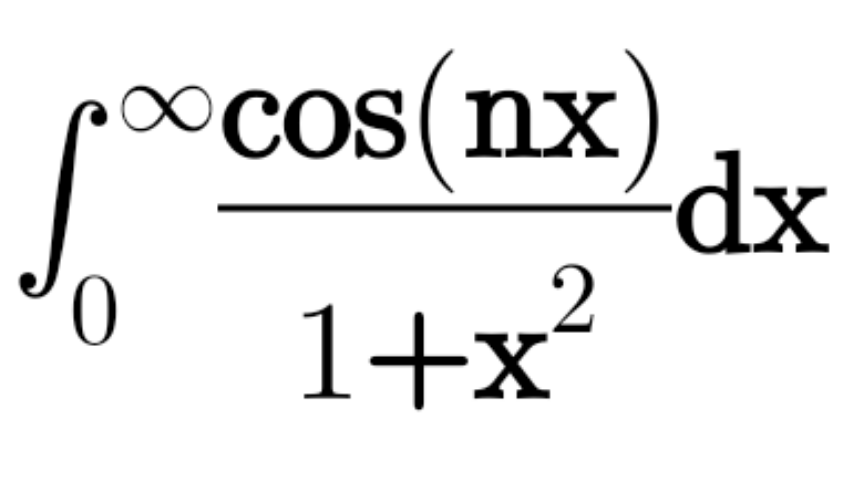
Question Number 168428 Answers: 1 Comments: 0
Question Number 168427 Answers: 2 Comments: 2
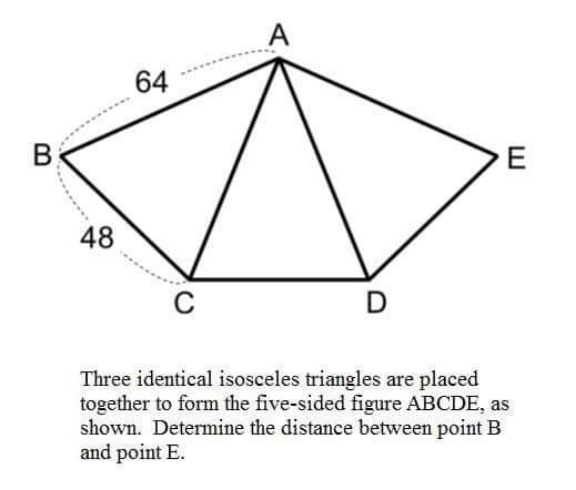
Question Number 168421 Answers: 0 Comments: 0
Question Number 168411 Answers: 0 Comments: 0

Question Number 168424 Answers: 0 Comments: 0
Question Number 168405 Answers: 1 Comments: 2
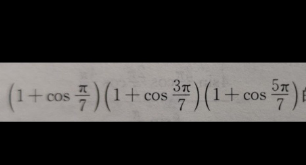
Question Number 168402 Answers: 0 Comments: 1
Question Number 168400 Answers: 0 Comments: 1

Question Number 168399 Answers: 1 Comments: 0
Pg 495 Pg 496 Pg 497 Pg 498 Pg 499 Pg 500 Pg 501 Pg 502 Pg 503 Pg 504
