
AllQuestion and Answers: Page 440
Question Number 174356 Answers: 2 Comments: 0
Question Number 174351 Answers: 1 Comments: 0

Question Number 174350 Answers: 0 Comments: 1
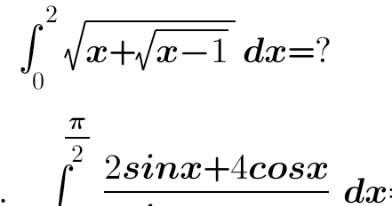
Question Number 174346 Answers: 1 Comments: 0
Question Number 174341 Answers: 3 Comments: 1
Question Number 174340 Answers: 0 Comments: 0
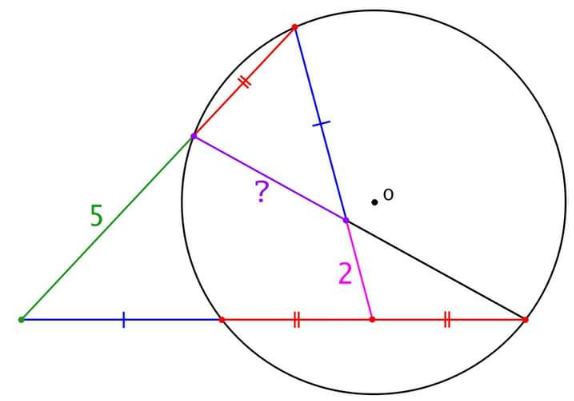
Question Number 174331 Answers: 0 Comments: 1

Question Number 174327 Answers: 1 Comments: 0
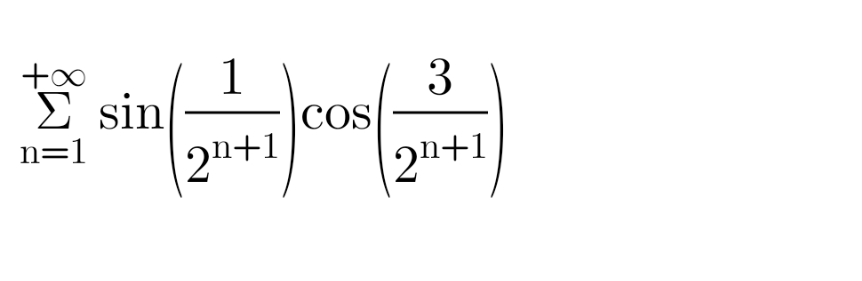
Question Number 174324 Answers: 2 Comments: 5
Question Number 176895 Answers: 2 Comments: 0
Question Number 176894 Answers: 1 Comments: 0
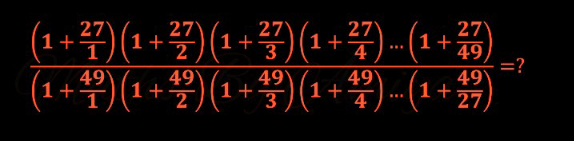
Question Number 176893 Answers: 1 Comments: 0
Question Number 176891 Answers: 1 Comments: 0

Question Number 174367 Answers: 0 Comments: 2
Question Number 174315 Answers: 1 Comments: 0
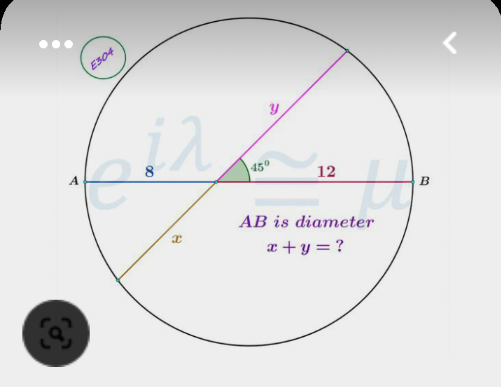
Question Number 174304 Answers: 1 Comments: 0
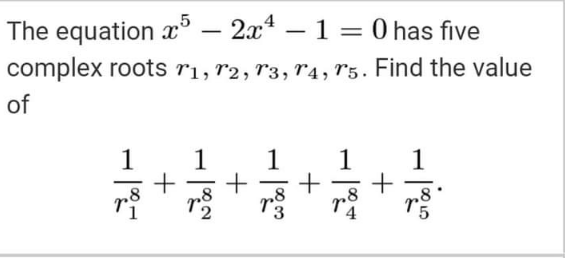
Question Number 174303 Answers: 1 Comments: 0

Question Number 174292 Answers: 1 Comments: 0
Question Number 174291 Answers: 1 Comments: 0
Question Number 174288 Answers: 1 Comments: 2

Question Number 174287 Answers: 2 Comments: 0

Question Number 174283 Answers: 1 Comments: 1
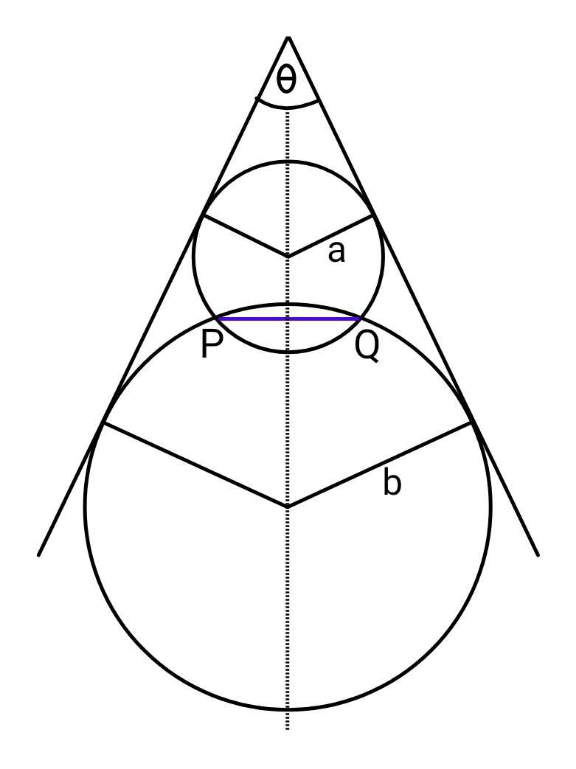
Question Number 174276 Answers: 2 Comments: 0

Question Number 174271 Answers: 0 Comments: 2
Question Number 174269 Answers: 2 Comments: 0
$${solve}\:\left({x}^{\mathrm{2}} −\mathrm{3}\right)^{\mathrm{2}} ={x}+\mathrm{3} \\ $$
Question Number 174267 Answers: 0 Comments: 7
Pg 435 Pg 436 Pg 437 Pg 438 Pg 439 Pg 440 Pg 441 Pg 442 Pg 443 Pg 444
