
AllQuestion and Answers: Page 380
Question Number 181318 Answers: 2 Comments: 1
Question Number 181313 Answers: 2 Comments: 0
Question Number 181296 Answers: 1 Comments: 0

Question Number 181312 Answers: 0 Comments: 0

Question Number 181280 Answers: 1 Comments: 0
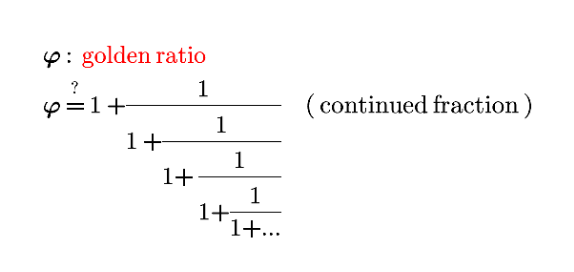
Question Number 181279 Answers: 3 Comments: 0
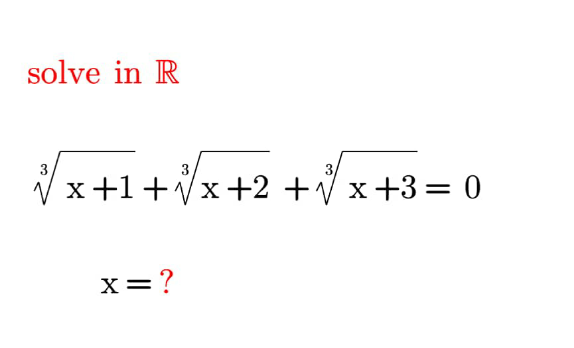
Question Number 181275 Answers: 1 Comments: 7
$${what}\:{is}\:{the}\:{sum}\:{of}\:{all} \\ $$$${even}\:{factors}\:{of}\:\mathrm{1000}? \\ $$
Question Number 181260 Answers: 2 Comments: 0
Question Number 181256 Answers: 0 Comments: 7
Question Number 181253 Answers: 0 Comments: 5
$${define}\:{microscopic}\:{and}\:{macroscopic} \\ $$$${with}\:{one}\:{one}\:{example}. \\ $$
Question Number 181243 Answers: 1 Comments: 0
Question Number 181238 Answers: 1 Comments: 0
Question Number 181232 Answers: 3 Comments: 1

Question Number 181221 Answers: 1 Comments: 0
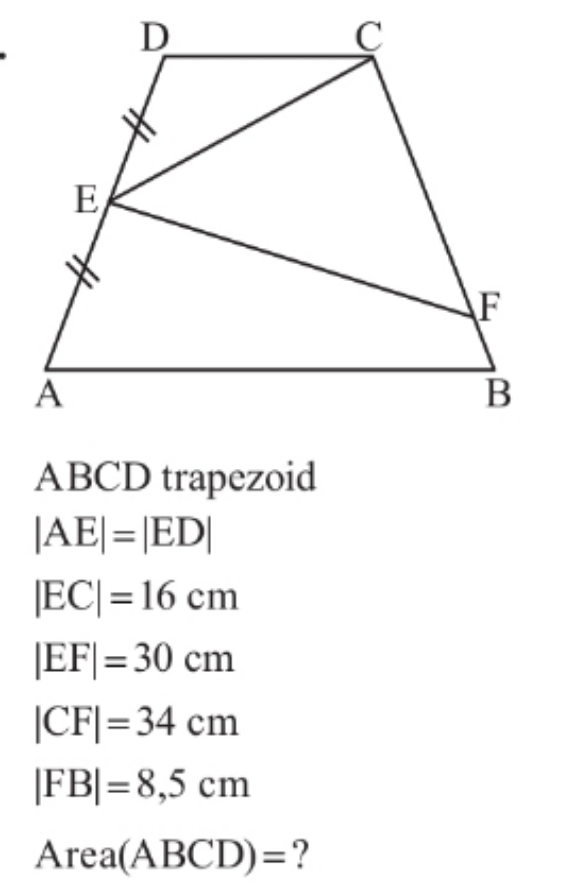
Question Number 181219 Answers: 1 Comments: 0
Question Number 181218 Answers: 1 Comments: 0
Question Number 181217 Answers: 1 Comments: 0

Question Number 181207 Answers: 1 Comments: 0
Question Number 181201 Answers: 3 Comments: 0

Question Number 181183 Answers: 1 Comments: 0
Question Number 181182 Answers: 1 Comments: 0
Question Number 181173 Answers: 0 Comments: 1

Question Number 181172 Answers: 0 Comments: 2
Question Number 181171 Answers: 0 Comments: 1
Question Number 181152 Answers: 0 Comments: 0
Question Number 181151 Answers: 4 Comments: 0

Pg 375 Pg 376 Pg 377 Pg 378 Pg 379 Pg 380 Pg 381 Pg 382 Pg 383 Pg 384
