
AllQuestion and Answers: Page 354
Question Number 185104 Answers: 2 Comments: 0

Question Number 185101 Answers: 2 Comments: 0

Question Number 185090 Answers: 1 Comments: 2
Question Number 185087 Answers: 2 Comments: 0
Question Number 185086 Answers: 1 Comments: 0
Question Number 185085 Answers: 2 Comments: 0
Question Number 185077 Answers: 1 Comments: 0
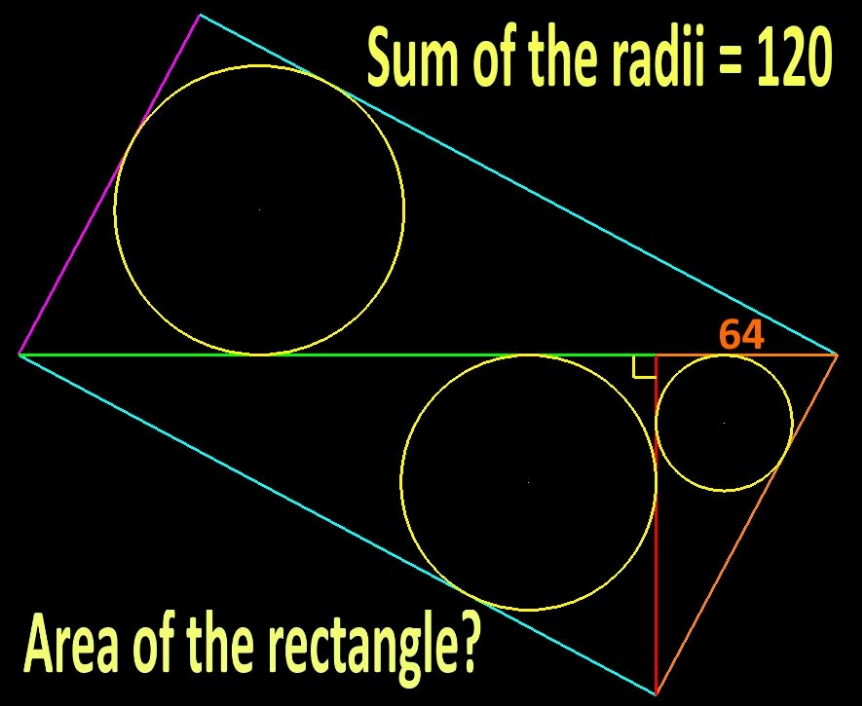
Question Number 185076 Answers: 2 Comments: 0
$${x}^{\mathrm{2}{x}^{\mathrm{6}} } =\mathrm{3} \\ $$$${x}=? \\ $$
Question Number 185075 Answers: 0 Comments: 5
Question Number 185073 Answers: 1 Comments: 0

Question Number 185058 Answers: 1 Comments: 0

Question Number 185054 Answers: 1 Comments: 0
Question Number 185053 Answers: 1 Comments: 0

Question Number 185052 Answers: 0 Comments: 3

Question Number 185042 Answers: 0 Comments: 0
Question Number 185038 Answers: 1 Comments: 0
Question Number 185036 Answers: 1 Comments: 0
Question Number 185030 Answers: 1 Comments: 1
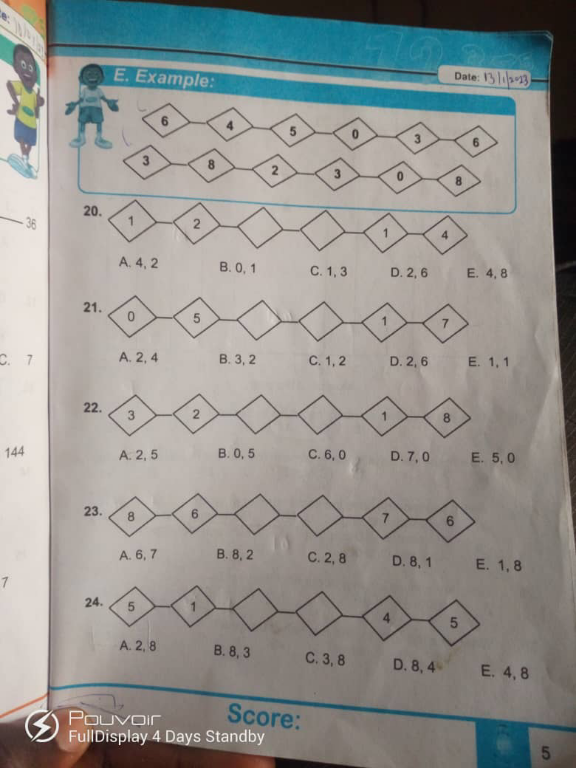
Question Number 185024 Answers: 1 Comments: 0
Question Number 185023 Answers: 1 Comments: 0
Question Number 185014 Answers: 1 Comments: 1
Question Number 185013 Answers: 4 Comments: 3

Question Number 185012 Answers: 0 Comments: 2
Question Number 185011 Answers: 0 Comments: 0

Question Number 185010 Answers: 0 Comments: 0

Question Number 185009 Answers: 0 Comments: 0

Pg 349 Pg 350 Pg 351 Pg 352 Pg 353 Pg 354 Pg 355 Pg 356 Pg 357 Pg 358
