
AllQuestion and Answers: Page 336
Question Number 186327 Answers: 0 Comments: 0
Question Number 186323 Answers: 1 Comments: 1
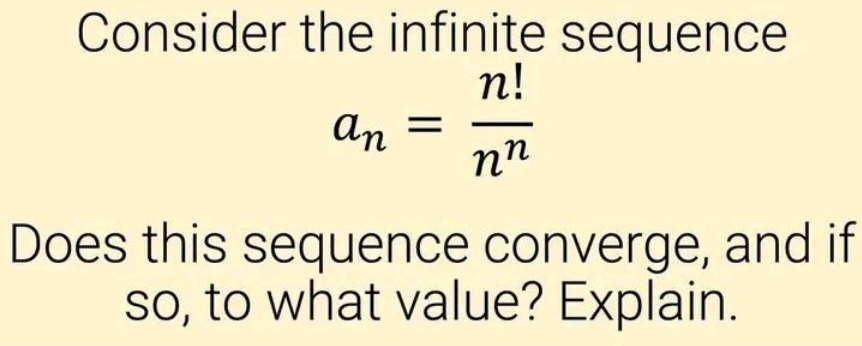
Question Number 186322 Answers: 1 Comments: 0

Question Number 186321 Answers: 3 Comments: 0

Question Number 186320 Answers: 1 Comments: 0

Question Number 186310 Answers: 1 Comments: 0
Question Number 186306 Answers: 0 Comments: 2
Question Number 186305 Answers: 0 Comments: 3
Question Number 186302 Answers: 1 Comments: 3

Question Number 186301 Answers: 1 Comments: 0
Question Number 186300 Answers: 1 Comments: 0
Question Number 186298 Answers: 1 Comments: 0
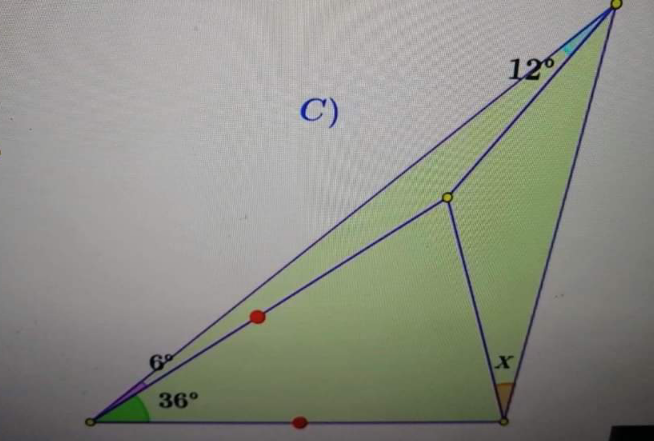
Question Number 186597 Answers: 1 Comments: 0

Question Number 186594 Answers: 5 Comments: 1
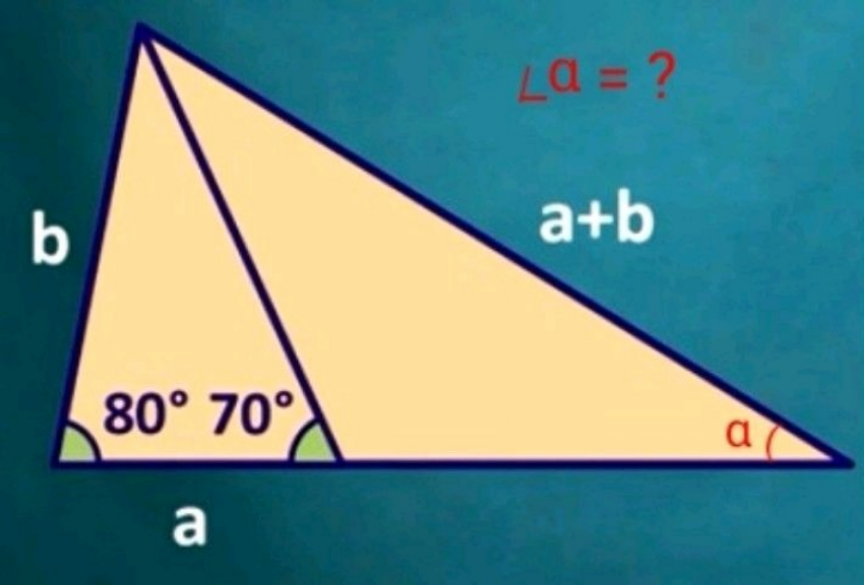
Question Number 186293 Answers: 0 Comments: 4

Question Number 186292 Answers: 0 Comments: 0
Question Number 186285 Answers: 0 Comments: 2
Question Number 186283 Answers: 0 Comments: 0
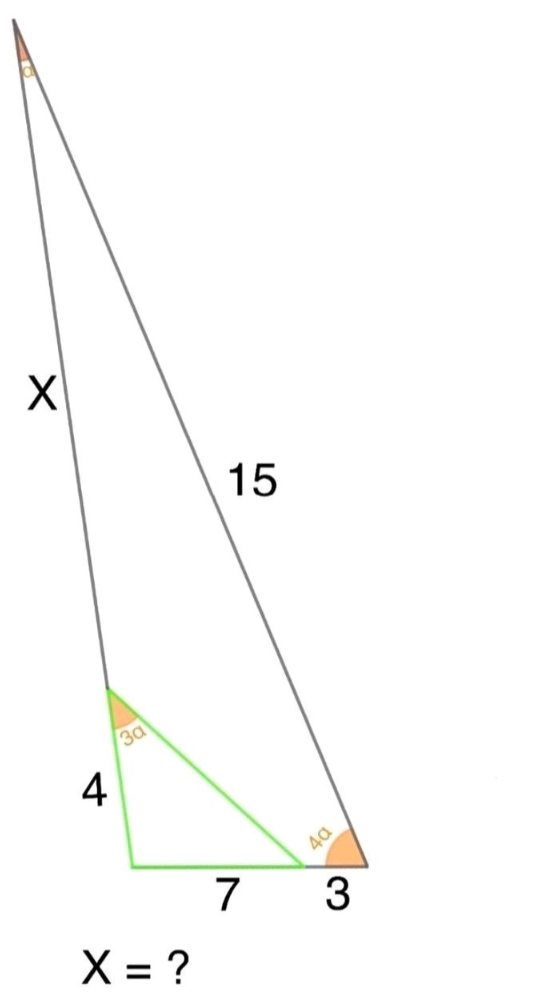
Question Number 186282 Answers: 1 Comments: 0
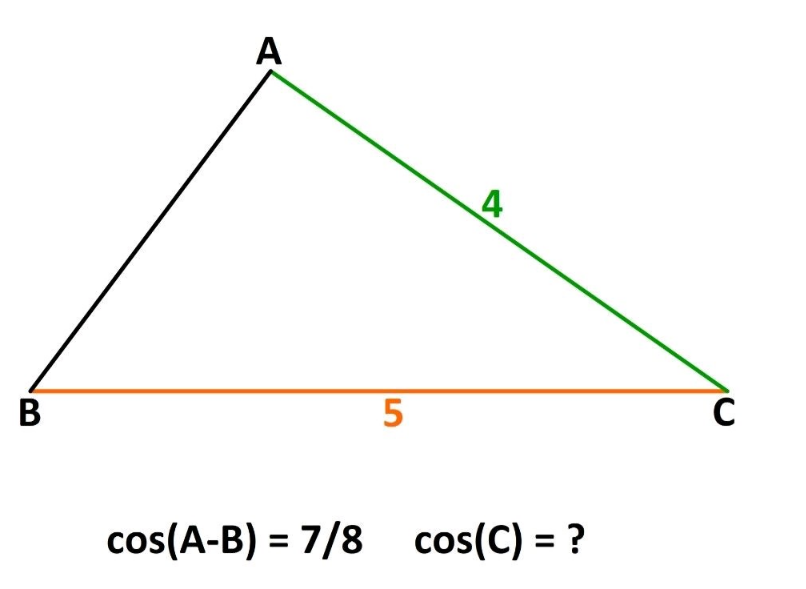
Question Number 186271 Answers: 2 Comments: 0

Question Number 186267 Answers: 1 Comments: 1
Question Number 186265 Answers: 1 Comments: 0
Question Number 186263 Answers: 2 Comments: 0
Question Number 186256 Answers: 0 Comments: 1
Question Number 186253 Answers: 1 Comments: 1
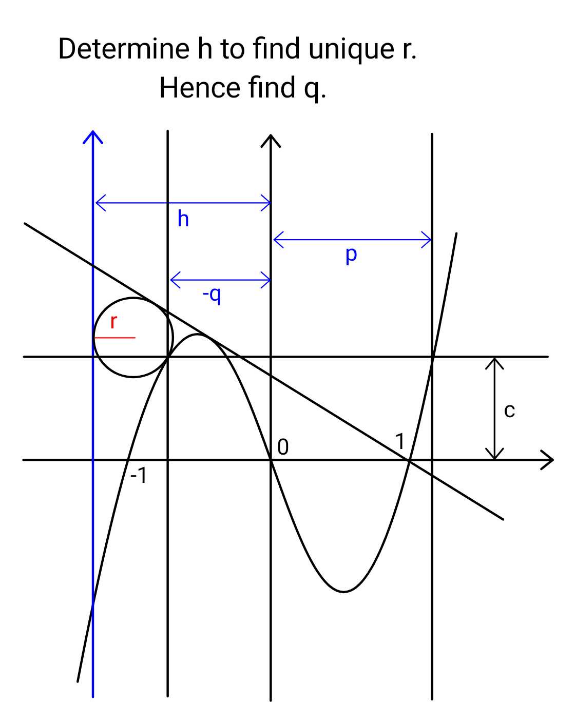
Question Number 186249 Answers: 0 Comments: 0

Pg 331 Pg 332 Pg 333 Pg 334 Pg 335 Pg 336 Pg 337 Pg 338 Pg 339 Pg 340
