Question and Answers Forum

AllQuestion and Answers: Page 332

Question Number 186764 Answers: 0 Comments: 3

Question Number 186762 Answers: 3 Comments: 4

Question Number 186758 Answers: 2 Comments: 0

If [t] denotes the integral part of t, then lim_(x→1) [x sin πx] (A) equals 1 (B) equals −1 (C) equals 0 (D) does not exist

$$\mathrm{If}\:\left[{t}\right]\:\mathrm{denotes}\:\mathrm{the}\:\mathrm{integral}\:\mathrm{part}\:\mathrm{of}\:{t},\:\mathrm{then}\:\underset{{x}\rightarrow\mathrm{1}} {\mathrm{lim}}\left[{x}\:\mathrm{sin}\:\pi{x}\right] \\ $$$$\left(\mathrm{A}\right)\:\:\mathrm{equals}\:\mathrm{1}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{B}\right)\:\:\mathrm{equals}\:−\mathrm{1} \\ $$$$\left(\mathrm{C}\right)\:\:\mathrm{equals}\:\mathrm{0}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{D}\right)\:\mathrm{does}\:\mathrm{not}\:\mathrm{exist} \\ $$

Question Number 186752 Answers: 1 Comments: 0

lim_(x→+∞) (((√(x^3 −3x^2 +7))+((x^4 +3))^(1/3) )/( ((x^6 +2x^5 +1))^(1/4) −((x^7 +2x^3 +3))^(1/5) )) Please show work.

$$\underset{{x}\rightarrow+\infty} {\mathrm{lim}}\:\frac{\sqrt{{x}^{\mathrm{3}} −\mathrm{3}{x}^{\mathrm{2}} +\mathrm{7}}+\sqrt[{\mathrm{3}}]{{x}^{\mathrm{4}} +\mathrm{3}}}{\:\sqrt[{\mathrm{4}}]{{x}^{\mathrm{6}} +\mathrm{2}{x}^{\mathrm{5}} +\mathrm{1}}−\sqrt[{\mathrm{5}}]{{x}^{\mathrm{7}} +\mathrm{2}{x}^{\mathrm{3}} +\mathrm{3}}} \\ $$$${Please}\:{show}\:{work}. \\ $$

Question Number 186751 Answers: 3 Comments: 0

Question Number 186750 Answers: 1 Comments: 0

Question Number 186748 Answers: 0 Comments: 0

Let f:R^+ →R^+ be a function satisfying the relation f(x.f(y))=f(xy)+x for all x, y ∈R^+ . Then lim_(x→0) ((((f(x))^(1/3) −1)/((f(x))^(1/2) −1)))= (A) 1 (B) (1/2) (C) (2/3) (D) (3/2)

Question Number 186741 Answers: 1 Comments: 0

cos ((π/(18))).cos (((3π)/(18))).cos (((5π)/(18))).cos (((7π)/(18)))=?

$$\:\mathrm{cos}\:\left(\frac{\pi}{\mathrm{18}}\right).\mathrm{cos}\:\left(\frac{\mathrm{3}\pi}{\mathrm{18}}\right).\mathrm{cos}\:\left(\frac{\mathrm{5}\pi}{\mathrm{18}}\right).\mathrm{cos}\:\left(\frac{\mathrm{7}\pi}{\mathrm{18}}\right)=? \\ $$

Question Number 186739 Answers: 1 Comments: 0

((5+((5+((5+...))^(1/3) ))^(1/3) ))^(1/3) =?

$$\sqrt[{\mathrm{3}}]{\mathrm{5}+\sqrt[{\mathrm{3}}]{\mathrm{5}+\sqrt[{\mathrm{3}}]{\mathrm{5}+...}}}=? \\ $$

Question Number 186737 Answers: 1 Comments: 1

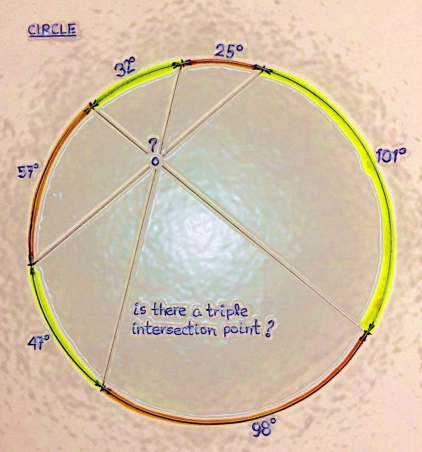
Question Number 186736 Answers: 1 Comments: 0

Question Number 186735 Answers: 1 Comments: 0

Question Number 186726 Answers: 2 Comments: 0

Question Number 186721 Answers: 1 Comments: 1

Question Number 186705 Answers: 1 Comments: 1

a,b>0 , a+b=2 Prove that a^(2b) +b^(2a) +(((a−b)/2))^2 ≤2

$$\mathrm{a},\mathrm{b}>\mathrm{0}\:,\:\mathrm{a}+\mathrm{b}=\mathrm{2} \\ $$$$\mathrm{Prove}\:\mathrm{that}\:\mathrm{a}^{\mathrm{2b}} +\mathrm{b}^{\mathrm{2a}} +\left(\frac{\mathrm{a}−\mathrm{b}}{\mathrm{2}}\right)^{\mathrm{2}} \leqslant\mathrm{2} \\ $$

Question Number 186701 Answers: 2 Comments: 2

Question Number 186698 Answers: 0 Comments: 0

Question Number 186697 Answers: 2 Comments: 0

Question Number 186692 Answers: 1 Comments: 1

Question Number 186691 Answers: 1 Comments: 0

Question Number 186690 Answers: 1 Comments: 0

Question Number 186689 Answers: 1 Comments: 1

Σ_(n=o) ^(+oo) (x^(3n) /((2n)!)) = ?

$$\underset{{n}={o}} {\overset{+{oo}} {\sum}}\:\frac{{x}^{\mathrm{3}{n}} }{\left(\mathrm{2}{n}\right)!}\:\:=\:\:\:? \\ $$

Question Number 186688 Answers: 0 Comments: 1

a_1 =0 a_2 =1 a_(n+2) =a_(n+1) −a_n a_(885) =?

$${a}_{\mathrm{1}} =\mathrm{0} \\ $$$${a}_{\mathrm{2}} =\mathrm{1} \\ $$$${a}_{{n}+\mathrm{2}} ={a}_{{n}+\mathrm{1}} −{a}_{{n}} \\ $$$${a}_{\mathrm{885}} =? \\ $$

Question Number 186685 Answers: 1 Comments: 0

(sinx)^2 −(1/2)sin2x−2(cosx)^2 ≥0 x∈[0;2π]

$$\left(\mathrm{sin}{x}\right)^{\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{2}}\mathrm{sin2}{x}−\mathrm{2}\left(\mathrm{cos}{x}\right)^{\mathrm{2}} \geq\mathrm{0} \\ $$$${x}\in\left[\mathrm{0};\mathrm{2}\pi\right] \\ $$

Question Number 186682 Answers: 0 Comments: 0

Prove the following set identities 1) A∪(B∪C)=(A∪B)∪C 2) A∪∅=A 3) A∩(B∪C)=(A∩B)∪(A∩C)

$$\mathrm{Prove}\:\mathrm{the}\:\mathrm{following}\:\mathrm{set}\:\mathrm{identities} \\ $$$$\left.\mathrm{1}\right)\:\mathrm{A}\cup\left(\mathrm{B}\cup\mathrm{C}\right)=\left(\mathrm{A}\cup\mathrm{B}\right)\cup\mathrm{C} \\ $$$$\left.\mathrm{2}\right)\:\mathrm{A}\cup\varnothing=\mathrm{A} \\ $$$$\left.\mathrm{3}\right)\:\mathrm{A}\cap\left(\mathrm{B}\cup\mathrm{C}\right)=\left(\mathrm{A}\cap\mathrm{B}\right)\cup\left(\mathrm{A}\cap\mathrm{C}\right) \\ $$

Question Number 186681 Answers: 0 Comments: 0

Etudier la convergence uniforme Σ_(n=0) ^(+∞) (−)^n (e^(−nx^2 ) /((1+n)^3 )) ; n ∈ N.

$${Etudier}\:{la}\:{convergence}\:{uniforme} \\ $$$$\underset{{n}=\mathrm{0}} {\overset{+\infty} {\sum}}\left(−\right)^{{n}} \frac{{e}^{−{nx}^{\mathrm{2}} } }{\left(\mathrm{1}+{n}\right)^{\mathrm{3}} }\:;\:{n}\:\in\:\mathbb{N}. \\ $$

Pg 327 Pg 328 Pg 329 Pg 330 Pg 331 Pg 332 Pg 333 Pg 334 Pg 335 Pg 336

Contact: info@tinkutara.com