Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 331

Question Number 187227 Answers: 0 Comments: 0

Question Number 187223 Answers: 0 Comments: 0

Question Number 187274 Answers: 0 Comments: 1

Question Number 187216 Answers: 1 Comments: 1

Question Number 187215 Answers: 1 Comments: 0

((√(n!))/n)=(√(20)) ; n=?

$$\frac{\sqrt{{n}!}}{{n}}=\sqrt{\mathrm{20}}\:;\:{n}=? \\ $$

Question Number 187205 Answers: 3 Comments: 0

if the sides of a triangle are (√(a^2 +b^2 )), (√(a^2 +4b^2 )), (√(4a^2 +b^2 )) respectively, what is its area?

$${if}\:{the}\:{sides}\:{of}\:{a}\:{triangle}\:{are} \\ $$$$\sqrt{{a}^{\mathrm{2}} +{b}^{\mathrm{2}} },\:\sqrt{{a}^{\mathrm{2}} +\mathrm{4}{b}^{\mathrm{2}} },\:\sqrt{\mathrm{4}{a}^{\mathrm{2}} +{b}^{\mathrm{2}} }\:{respectively}, \\ $$$${what}\:{is}\:{its}\:{area}? \\ $$

Question Number 187213 Answers: 0 Comments: 0

If a,b are co-prime natural numbers satisfying a^2 + b = (a−b)^3 and b+1 is a prime find all possible values of (a, b). Please help me solve this.

$$\mathrm{If}\:{a},{b}\:\mathrm{are}\:\mathrm{co}-\mathrm{prime}\:\mathrm{natural}\:\mathrm{numbers}\:\mathrm{satisfying} \\ $$$${a}^{\mathrm{2}} \:+\:{b}\:=\:\left({a}−{b}\right)^{\mathrm{3}} \:\mathrm{and}\:{b}+\mathrm{1}\:\mathrm{is}\:\mathrm{a}\:\mathrm{prime}\:\mathrm{find}\:\mathrm{all} \\ $$$$\mathrm{possible}\:\mathrm{values}\:\mathrm{of}\:\left({a},\:{b}\right). \\ $$$$\mathrm{Please}\:\mathrm{help}\:\mathrm{me}\:\mathrm{solve}\:\mathrm{this}. \\ $$$$ \\ $$

Question Number 187209 Answers: 0 Comments: 1

Question Number 187191 Answers: 2 Comments: 2

Question Number 187190 Answers: 2 Comments: 0

Question Number 187186 Answers: 2 Comments: 6

Question Number 187184 Answers: 0 Comments: 0

×/ { { {{×=

$$ ×/ \left\{ \left\{ \left\{\left\{×=\right.\right.\right.\right. \\ $$

Question Number 187177 Answers: 1 Comments: 0

2^y y^2 +(2y)^((2y)) =272

$$ \\ $$$$\mathrm{2}^{{y}} {y}^{\mathrm{2}} +\left(\mathrm{2}{y}\right)^{\left(\mathrm{2}{y}\right)} =\mathrm{272} \\ $$

Question Number 187176 Answers: 1 Comments: 0

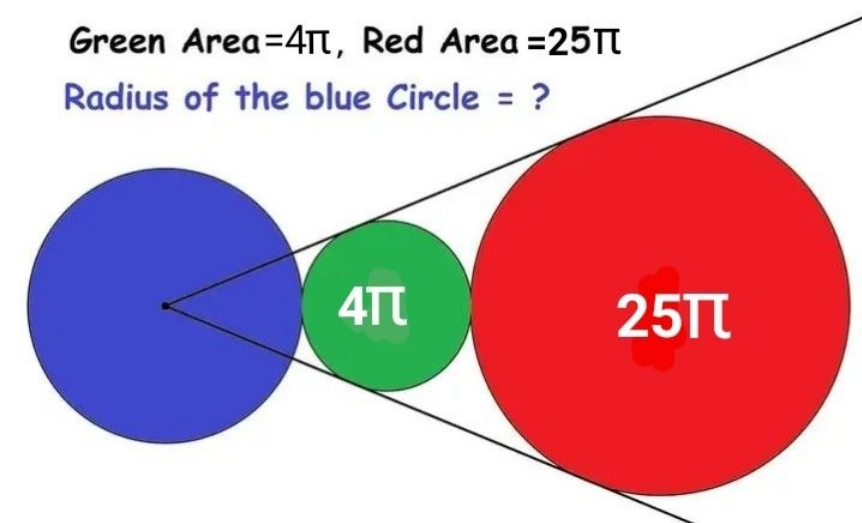
Question Number 187168 Answers: 0 Comments: 0

Question Number 187167 Answers: 3 Comments: 0

Question Number 187166 Answers: 0 Comments: 0

x^3 =x+c let x=((mt)/(1−t)) m^3 t^3 =mt(1−t)^2 +c(1−t)^3 ⇒ (m^3 −m−c)t^3 +(2m−3c)t^2 +(3c−m)t−c=0 t^3 +At^2 +Bt+C=0 let AB=C ⇒ (2m−3c)(m−3c)=c(m^3 −m−c) ⇒ m^3 −(2/c)m^2 −8m+10c=0 ...

$${x}^{\mathrm{3}} ={x}+{c} \\ $$$${let}\:\:{x}=\frac{{mt}}{\mathrm{1}−{t}} \\ $$$${m}^{\mathrm{3}} {t}^{\mathrm{3}} ={mt}\left(\mathrm{1}−{t}\right)^{\mathrm{2}} +{c}\left(\mathrm{1}−{t}\right)^{\mathrm{3}} \\ $$$$\Rightarrow \\ $$$$\left({m}^{\mathrm{3}} −{m}−{c}\right){t}^{\mathrm{3}} +\left(\mathrm{2}{m}−\mathrm{3}{c}\right){t}^{\mathrm{2}} \\ $$$$\:\:\:\:\:+\left(\mathrm{3}{c}−{m}\right){t}−{c}=\mathrm{0} \\ $$$${t}^{\mathrm{3}} +{At}^{\mathrm{2}} +{Bt}+{C}=\mathrm{0} \\ $$$${let}\:\:{AB}={C} \\ $$$$\Rightarrow\:\left(\mathrm{2}{m}−\mathrm{3}{c}\right)\left({m}−\mathrm{3}{c}\right)={c}\left({m}^{\mathrm{3}} −{m}−{c}\right) \\ $$$$\Rightarrow\:{m}^{\mathrm{3}} −\frac{\mathrm{2}}{{c}}{m}^{\mathrm{2}} −\mathrm{8}{m}+\mathrm{10}{c}=\mathrm{0} \\ $$$$... \\ $$

Question Number 187164 Answers: 1 Comments: 0

Question Number 187155 Answers: 2 Comments: 2

f(x)=x.e^(2x) =>f^((n)) (x)=

$${f}\left({x}\right)={x}.{e}^{\mathrm{2}{x}} \\ $$$$=>{f}^{\left({n}\right)} \left({x}\right)= \\ $$$$ \\ $$

Question Number 187146 Answers: 2 Comments: 0

Question Number 187144 Answers: 0 Comments: 1

determiner les elements demandes (fig ci−joint)

$${determiner}\:{les}\:{elements} \\ $$$${demandes}\:\left({fig}\:{ci}−{joint}\right) \\ $$

Question Number 187142 Answers: 1 Comments: 2

Montrer que: 4r^2 =a^2 +b^2 +c^2 +d^2

$$\:{Montrer}\:{que}: \\ $$$$\mathrm{4}{r}^{\mathrm{2}} ={a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} +{d}^{\mathrm{2}} \\ $$$$ \\ $$

Question Number 187135 Answers: 1 Comments: 0

∫_( 0) ^( π/4) ((tan^2 x)/(1+sin x)) dx =?

$$\:\underset{\:\:\mathrm{0}} {\overset{\:\:\pi/\mathrm{4}} {\int}}\:\frac{\mathrm{tan}\:^{\mathrm{2}} {x}}{\mathrm{1}+\mathrm{sin}\:{x}}\:{dx}\:=? \\ $$

Question Number 187132 Answers: 0 Comments: 0

Question Number 187124 Answers: 2 Comments: 0

(a/x)=(b/y)=(c/z)=(1/3) , a−2b+c=2 and −2y+z=1 x=? An altered form of q#187020 (In this case solveable)

$$\frac{{a}}{{x}}=\frac{{b}}{{y}}=\frac{{c}}{{z}}=\frac{\mathrm{1}}{\mathrm{3}}\:, \\ $$$${a}−\mathrm{2}{b}+{c}=\mathrm{2}\:\:{and}\:\:−\mathrm{2}{y}+{z}=\mathrm{1}\:\:\:\: \\ $$$${x}=? \\ $$$${An}\:{altered}\:{form}\:{of}\:\:{q}#\mathrm{187020} \\ $$$$\left({In}\:{this}\:{case}\:{solveable}\right) \\ $$

Question Number 187100 Answers: 1 Comments: 0

Pg 326 Pg 327 Pg 328 Pg 329 Pg 330 Pg 331 Pg 332 Pg 333 Pg 334 Pg 335

Contact: info@tinkutara.com