Question and Answers Forum

AllQuestion and Answers: Page 325

Question Number 187478 Answers: 1 Comments: 0

let a and b be positive integers such that ab + 1 divides a^2 + b^2 . show that ((a^2 + b^2 )/(ab + 1)) is the sequare of an integer.

$$\: \\ $$$$\:\:\:\boldsymbol{{let}}\:\boldsymbol{{a}}\:\boldsymbol{{and}}\:\boldsymbol{{b}}\:\boldsymbol{{be}}\:\boldsymbol{{positive}}\:\boldsymbol{{integers}}\:\:\:\:\: \\ $$$$\:\:\:\boldsymbol{{such}}\:\boldsymbol{{that}}\:\boldsymbol{{ab}}\:+\:\mathrm{1}\:\boldsymbol{{divides}}\:\boldsymbol{{a}}^{\mathrm{2}} \:+\:\boldsymbol{{b}}^{\mathrm{2}} .\:\:\:\:\: \\ $$$$\:\:\:\:\:\boldsymbol{{show}}\:\boldsymbol{{that}}\:\frac{\boldsymbol{{a}}^{\mathrm{2}} \:+\:\boldsymbol{{b}}^{\mathrm{2}} }{\boldsymbol{{ab}}\:+\:\mathrm{1}} \\ $$$$\:\:\:\:\:\:\boldsymbol{{is}}\:\boldsymbol{{the}}\:\boldsymbol{{sequare}}\:\boldsymbol{{of}}\:\boldsymbol{{an}}\:\boldsymbol{{integer}}. \\ $$$$ \\ $$

Question Number 187476 Answers: 1 Comments: 0

Question Number 187473 Answers: 1 Comments: 0

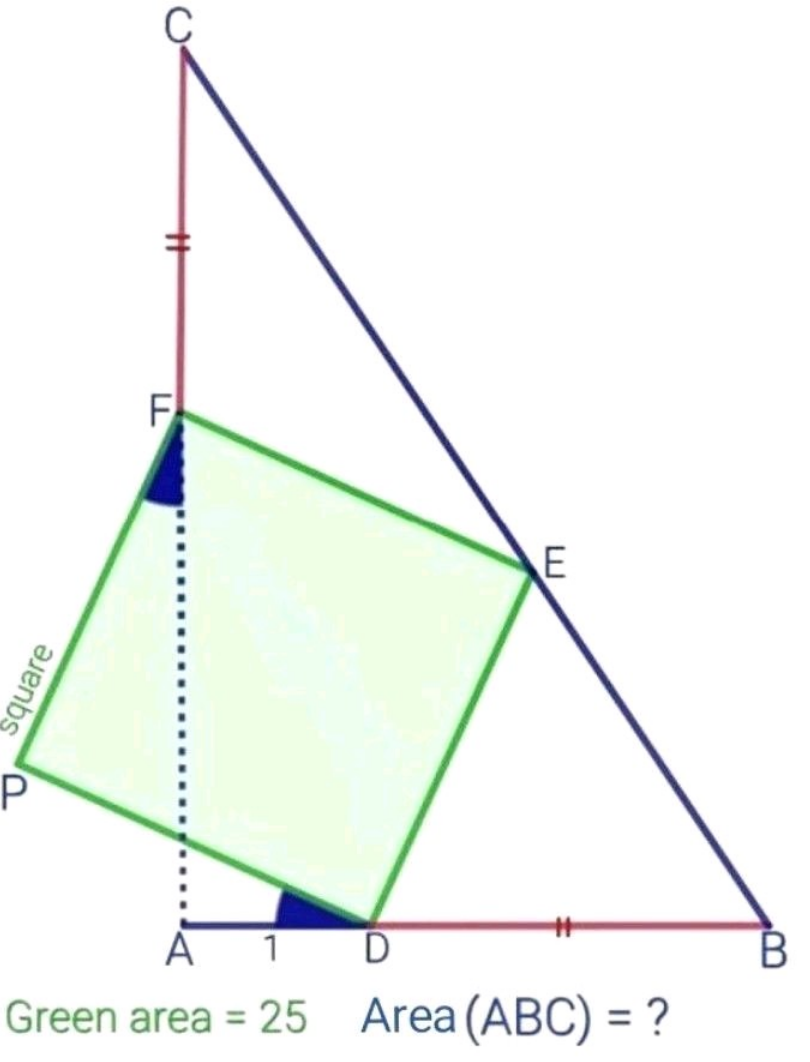
Question Number 187458 Answers: 2 Comments: 0

Ω= ∫_0 ^( (π/2)) (( 2sin(x) − cos(x))/(sin(x) + cos (x))) dx = ? −−−−

$$ \\ $$$$ \\ $$$$\:\:\Omega=\:\int_{\mathrm{0}} ^{\:\frac{\pi}{\mathrm{2}}} \frac{\:\:\mathrm{2}{sin}\left({x}\right)\:−\:{cos}\left({x}\right)}{{sin}\left({x}\right)\:+\:{cos}\:\left({x}\right)}\:{dx}\:=\:? \\ $$$$−−−− \\ $$

Question Number 187453 Answers: 2 Comments: 0

∫_0 ^1 (√(sinx))dx=?

$$\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\sqrt{\mathrm{sin}{x}}\mathrm{d}{x}=? \\ $$

Question Number 187452 Answers: 2 Comments: 0

Question Number 187451 Answers: 1 Comments: 0

Question Number 187449 Answers: 0 Comments: 0

Question Number 187444 Answers: 0 Comments: 1

2x+(3/8)=(x)^(1/4) x=?

$$\mathrm{2}{x}+\frac{\mathrm{3}}{\mathrm{8}}=\sqrt[{\mathrm{4}}]{{x}} \\ $$$${x}=? \\ $$

Question Number 187442 Answers: 1 Comments: 0

If (7^x /(1 + 7^x )) = (3/7) find ((2401^x )/(1 + 2401^x )) = ?

$$\mathrm{If}\:\:\:\:\:\frac{\mathrm{7}^{\boldsymbol{\mathrm{x}}} }{\mathrm{1}\:+\:\mathrm{7}^{\boldsymbol{\mathrm{x}}} }\:\:=\:\:\frac{\mathrm{3}}{\mathrm{7}}\:\:\:\:\:\mathrm{find}\:\:\:\:\frac{\mathrm{2401}^{\boldsymbol{\mathrm{x}}} }{\mathrm{1}\:+\:\mathrm{2401}^{\boldsymbol{\mathrm{x}}} }\:\:=\:\:? \\ $$

Question Number 187440 Answers: 0 Comments: 1

if y^(x−y) =(x^2 /y) then x−y=?

$${if}\:\:{y}^{{x}−{y}} =\frac{{x}^{\mathrm{2}} }{{y}}\:{then}\:{x}−{y}=? \\ $$

Question Number 187437 Answers: 1 Comments: 0

Question Number 187433 Answers: 1 Comments: 0

Prove that ▽×▽φ=0. If φ is assumed to be continous and having continous first and second order derivatives. M.m

$$\mathrm{Prove}\:\mathrm{that}\:\bigtriangledown×\bigtriangledown\phi=\mathrm{0}.\:\mathrm{If}\:\phi\:\mathrm{is} \\ $$$$\mathrm{assumed}\:\mathrm{to}\:\mathrm{be}\:\mathrm{continous}\:\mathrm{and} \\ $$$$\mathrm{having}\:\mathrm{continous}\:\mathrm{first}\:\mathrm{and}\:\mathrm{second} \\ $$$$\mathrm{order}\:\mathrm{derivatives}. \\ $$$$ \\ $$$$\mathrm{M}.\mathrm{m} \\ $$

Question Number 187408 Answers: 2 Comments: 0

∫((sec^2 x)/( (√(1−tan^2 x))))dx

$$\:\int\frac{\boldsymbol{\mathrm{sec}}^{\mathrm{2}} \boldsymbol{\mathrm{x}}}{\:\sqrt{\mathrm{1}−\boldsymbol{\mathrm{tan}}^{\mathrm{2}} \boldsymbol{\mathrm{x}}}}\boldsymbol{\mathrm{dx}} \\ $$

Question Number 187406 Answers: 1 Comments: 2

3^x .8^(x/(x+2)) =6ln0−log10

$$\mathrm{3}^{{x}} .\mathrm{8}^{\frac{{x}}{{x}+\mathrm{2}}} =\mathrm{6}{ln}\mathrm{0}−{log}\mathrm{10} \\ $$

Question Number 187401 Answers: 0 Comments: 0

a∈C determinant (((a 1 … 1)),((1 ⋱ ⋱ ⋮)),((⋮ ⋱ ⋱ 1)),((1 … 1 a)))=?

$$\mathrm{a}\in\mathbb{C} \\ $$$$\begin{vmatrix}{\mathrm{a}\:\:\:\mathrm{1}\:\:\:\:\ldots\:\:\:\mathrm{1}}\\{\mathrm{1}\:\:\:\ddots\:\:\ddots\:\:\vdots}\\{\vdots\:\:\ddots\:\:\ddots\:\:\mathrm{1}}\\{\mathrm{1}\:\:\:\:\ldots\:\:\:\:\mathrm{1}\:\:\:\mathrm{a}}\end{vmatrix}=? \\ $$

Question Number 187400 Answers: 1 Comments: 0

a,b∈C determinant (((a+b a 0 … 0)),(( b a+b ⋱ ⋱ ⋮)),(( 0 ⋱ ⋱ ⋱ 0)),(( ⋮ ⋱ ⋱ ⋱ a)),(( 0 … 0 b a+b)))=?

Question Number 187394 Answers: 1 Comments: 1

Question Number 187390 Answers: 2 Comments: 0

odd number (2n+1) The sum of twelve consecutive natural numbers is 288. Find the largest natural number

$${odd}\:{number}\:\left(\mathrm{2}{n}+\mathrm{1}\right) \\ $$The sum of twelve consecutive natural numbers is 288. Find the largest natural number

Question Number 187388 Answers: 2 Comments: 0

p^3 +q^3 =c , pq=(1/3) ∀ 0<c<(2/(3(√3))) Find p+q.

$${p}^{\mathrm{3}} +{q}^{\mathrm{3}} ={c}\:\:\:,\:\:{pq}=\frac{\mathrm{1}}{\mathrm{3}}\:\:\:\forall\:\:\:\mathrm{0}<{c}<\frac{\mathrm{2}}{\mathrm{3}\sqrt{\mathrm{3}}} \\ $$$${Find}\:{p}+{q}. \\ $$

Question Number 187381 Answers: 2 Comments: 0

3^x =5^y =225 Determiner: (1/x)+(1/y) ?

$$\mathrm{3}^{{x}} =\mathrm{5}^{{y}} =\mathrm{225} \\ $$$${Determiner}:\:\:\:\frac{\mathrm{1}}{{x}}+\frac{\mathrm{1}}{{y}}\:? \\ $$

Question Number 187379 Answers: 2 Comments: 0

if x and y are +ve integers x+xy+y=54 x+y=?

$${if}\:{x}\:{and}\:{y}\:{are}\:+{ve}\:{integers} \\ $$$${x}+{xy}+{y}=\mathrm{54} \\ $$$${x}+{y}=? \\ $$

Question Number 187377 Answers: 1 Comments: 1

Question Number 187376 Answers: 1 Comments: 0

Question Number 187375 Answers: 0 Comments: 2

Question Number 187374 Answers: 0 Comments: 2

∫(√((t+1)/(t(k−t))))dt=?

$$\int\sqrt{\frac{{t}+\mathrm{1}}{{t}\left({k}−{t}\right)}}{dt}=? \\ $$

Pg 320 Pg 321 Pg 322 Pg 323 Pg 324 Pg 325 Pg 326 Pg 327 Pg 328 Pg 329

Contact: info@tinkutara.com