Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 308

Question Number 189667 Answers: 0 Comments: 0

Question Number 189666 Answers: 0 Comments: 0

Question Number 189665 Answers: 1 Comments: 0

Question Number 189662 Answers: 0 Comments: 0

Question Number 189661 Answers: 0 Comments: 0

Question Number 189660 Answers: 0 Comments: 0

Question Number 189643 Answers: 1 Comments: 0

f(x)=((sinx+cosx)/3) R_(f(x)) =?

$$\mathrm{f}\left(\mathrm{x}\right)=\frac{\mathrm{sinx}+\mathrm{cosx}}{\mathrm{3}} \\ $$$$\mathrm{R}_{\mathrm{f}\left(\mathrm{x}\right)} =? \\ $$

Question Number 189641 Answers: 1 Comments: 3

A man has equal chances of travelling by air(A), bus(B) and train(T). the probability that when he travels by air, bus or train he will have an accident are (1/3), (3/5) and (1/(10)). find the probability that (a) he travelled and was involved in an accident (b) he was travelling by air given that he was involved in an accident. (c) he was travelling by bus or he arrived safely

$$\mathrm{A}\:\mathrm{man}\:\mathrm{has}\:\mathrm{equal}\:\mathrm{chances}\:\mathrm{of}\:\mathrm{travelling}\:\mathrm{by}\: \\ $$$$\mathrm{air}\left(\mathrm{A}\right),\:\mathrm{bus}\left(\mathrm{B}\right)\:\mathrm{and}\:\mathrm{train}\left(\mathrm{T}\right).\:\mathrm{the}\:\mathrm{probability} \\ $$$$\mathrm{that}\:\mathrm{when}\:\mathrm{he}\:\mathrm{travels}\:\mathrm{by}\:\mathrm{air},\:\mathrm{bus}\:\mathrm{or}\:\mathrm{train}\:\mathrm{he} \\ $$$$\mathrm{will}\:\mathrm{have}\:\mathrm{an}\:\mathrm{accident}\:\mathrm{are}\:\frac{\mathrm{1}}{\mathrm{3}},\:\frac{\mathrm{3}}{\mathrm{5}}\:\mathrm{and}\:\frac{\mathrm{1}}{\mathrm{10}}. \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{probability}\:\mathrm{that}\: \\ $$$$\left(\mathrm{a}\right)\:\mathrm{he}\:\mathrm{travelled}\:\mathrm{and}\:\mathrm{was}\:\mathrm{involved}\:\mathrm{in}\:\mathrm{an}\:\mathrm{accident} \\ $$$$\left(\mathrm{b}\right)\:\mathrm{he}\:\mathrm{was}\:\mathrm{travelling}\:\mathrm{by}\:\mathrm{air}\:\mathrm{given}\:\mathrm{that}\:\mathrm{he}\:\mathrm{was} \\ $$$$\mathrm{involved}\:\mathrm{in}\:\mathrm{an}\:\mathrm{accident}. \\ $$$$\left(\mathrm{c}\right)\:\mathrm{he}\:\mathrm{was}\:\mathrm{travelling}\:\mathrm{by}\:\mathrm{bus}\:\mathrm{or}\:\mathrm{he}\:\mathrm{arrived}\:\mathrm{safely} \\ $$

Question Number 189639 Answers: 1 Comments: 1

(((20)),(( 0)) ) (((10)),(( 1)) ) + (((20)),(( 1)) ) (((10)),(( 2)) ) +...+ ((( 20)),(( 9)) ) ((( 10)),(( 10)) ) =?

$$ \\ $$$$\:\:\begin{pmatrix}{\mathrm{20}}\\{\:\mathrm{0}}\end{pmatrix}\:\begin{pmatrix}{\mathrm{10}}\\{\:\mathrm{1}}\end{pmatrix}\:+\:\begin{pmatrix}{\mathrm{20}}\\{\:\mathrm{1}}\end{pmatrix}\:\begin{pmatrix}{\mathrm{10}}\\{\:\:\mathrm{2}}\end{pmatrix}\:+...+\:\begin{pmatrix}{\:\:\:\mathrm{20}}\\{\:\:\mathrm{9}}\end{pmatrix}\:\begin{pmatrix}{\:\:\mathrm{10}}\\{\:\mathrm{10}}\end{pmatrix}\:=? \\ $$$$ \\ $$

Question Number 189664 Answers: 1 Comments: 0

Question Number 189630 Answers: 2 Comments: 0

16^x +20^x =25^x x (∈ R) =?

$$\mathrm{16}^{{x}} +\mathrm{20}^{{x}} =\mathrm{25}^{{x}} \\ $$$${x}\:\left(\in\:\mathbb{R}\right)\:=? \\ $$

Question Number 189625 Answers: 2 Comments: 1

Question Number 189624 Answers: 1 Comments: 0

Question Number 189616 Answers: 1 Comments: 0

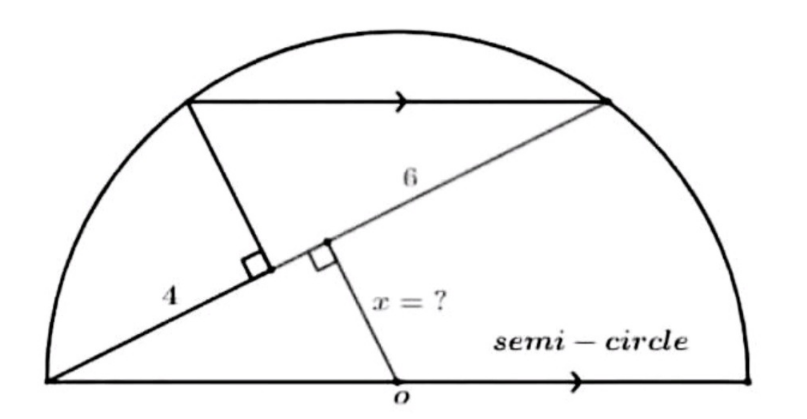
Question Number 189615 Answers: 1 Comments: 0

Question Number 189614 Answers: 1 Comments: 0

Question Number 189613 Answers: 1 Comments: 0

find nth term of (1,4,16,74,404,2578) ?

$${find}\:{nth}\:{term}\:{of}\:\left(\mathrm{1},\mathrm{4},\mathrm{16},\mathrm{74},\mathrm{404},\mathrm{2578}\right)\:? \\ $$

Question Number 189610 Answers: 1 Comments: 0

solve for x if log x=(x^2 /(25))

$${solve}\:{for}\:{x}\:{if}\:\:\: \\ $$$$\mathrm{log}\:{x}=\frac{{x}^{\mathrm{2}} }{\mathrm{25}} \\ $$

Question Number 189608 Answers: 2 Comments: 1

Question Number 189605 Answers: 0 Comments: 4

Question Number 189603 Answers: 2 Comments: 0

Question Number 189601 Answers: 0 Comments: 0

Question Number 189593 Answers: 0 Comments: 0

Question Number 189592 Answers: 0 Comments: 0

Question Number 189598 Answers: 0 Comments: 0

Question Number 189576 Answers: 1 Comments: 0

2^x + 2^x −4 + x = −(1/2) x = ???

$$ \\ $$$$\:\:\:\:\:\mathrm{2}^{\boldsymbol{{x}}} \:+\:\mathrm{2}^{\boldsymbol{{x}}} −\mathrm{4}\:+\:\boldsymbol{{x}}\:=\:−\frac{\mathrm{1}}{\mathrm{2}}\:\:\:\:\:\:\: \\ $$$$\:\:\:\:\:\:\boldsymbol{{x}}\:=\:??? \\ $$$$ \\ $$

Pg 303 Pg 304 Pg 305 Pg 306 Pg 307 Pg 308 Pg 309 Pg 310 Pg 311 Pg 312

Terms of Service

Contact: info@tinkutara.com