Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 269

Question Number 195107 Answers: 1 Comments: 0

Question Number 195101 Answers: 2 Comments: 0

(√(ln2)) >^? ln2

$$\sqrt{{ln}\mathrm{2}}\:\:\overset{?} {>}{ln}\mathrm{2} \\ $$

Question Number 195097 Answers: 1 Comments: 0

Question Number 195094 Answers: 1 Comments: 0

Question Number 195093 Answers: 2 Comments: 0

Question Number 195087 Answers: 2 Comments: 0

Question Number 195083 Answers: 1 Comments: 0

x=(√5)−2 x+(1/x)=?

$${x}=\sqrt{\mathrm{5}}−\mathrm{2}\:\:\:\:\: \\ $$$${x}+\frac{\mathrm{1}}{{x}}=? \\ $$

Question Number 195082 Answers: 0 Comments: 0

Question Number 195079 Answers: 1 Comments: 0

Question Number 195075 Answers: 1 Comments: 0

lim_(x→∞) ((sin^2 x−cos^3 x)/x)

$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\frac{{sin}^{\mathrm{2}} {x}−{cos}^{\mathrm{3}} {x}}{{x}} \\ $$

Question Number 195066 Answers: 0 Comments: 0

Question Number 195065 Answers: 2 Comments: 0

Question Number 195064 Answers: 0 Comments: 0

Question Number 195046 Answers: 2 Comments: 1

Question Number 195043 Answers: 2 Comments: 1

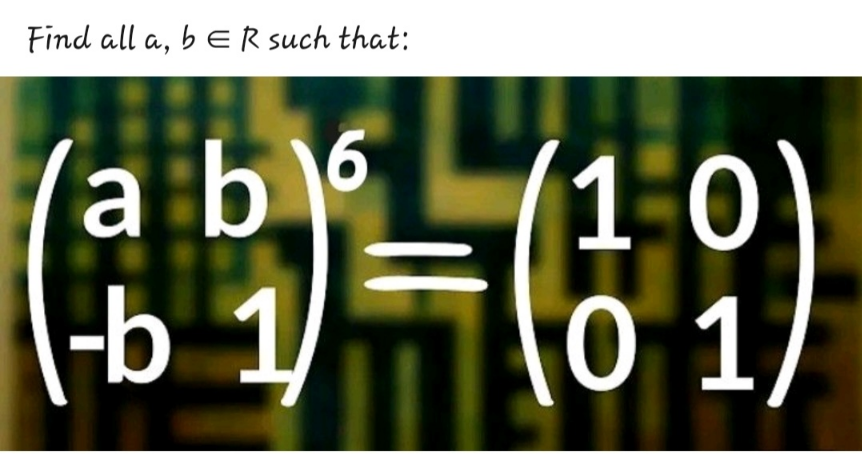
Question Number 195042 Answers: 1 Comments: 0

Question Number 195038 Answers: 0 Comments: 1

prove that x+y=(1/(x−y))

$${prove}\:{that}\:{x}+{y}=\frac{\mathrm{1}}{{x}−{y}} \\ $$

Question Number 195035 Answers: 2 Comments: 0

(√i)=?

$$\sqrt{{i}}=? \\ $$

Question Number 195033 Answers: 1 Comments: 0

any point is the function is not continous f(x)=(4x+8)^((ln45)/8) a) −8 b) −2 c) no one d) 5

$${any}\:{point}\:{is}\:{the}\:{function}\:{is} \\ $$$${not}\:{continous} \\ $$$${f}\left({x}\right)=\left(\mathrm{4}{x}+\mathrm{8}\right)^{\frac{{ln}\mathrm{45}}{\mathrm{8}}} \\ $$$$\left.{a}\left.\right)\:−\mathrm{8}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{b}\right)\:−\mathrm{2} \\ $$$$\left.{c}\left.\right)\:{no}\:{one}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{d}\right)\:\mathrm{5} \\ $$

Question Number 195029 Answers: 1 Comments: 0

lim_(x→0^+ ) ((((√(x+x^2 )) −(√x))/( (√(3x)) ln (1+x))) ).

$$\:\:\: \\ $$$$ \underset{{x}\rightarrow\mathrm{0}^{+} } {\mathrm{lim}}\:\left(\frac{\sqrt{{x}+{x}^{\mathrm{2}} }\:−\sqrt{{x}}}{\:\sqrt{\mathrm{3}{x}}\:\mathrm{ln}\:\left(\mathrm{1}+{x}\right)}\:\right).\: \\ $$

Question Number 195027 Answers: 1 Comments: 2

a_3 x^3 −x^2 +a_1 x−7=0 is a cubic polynomial in x whose Roots are α , β , γ positive real numbers satisfying ((225α^2 )/(α^2 +7))=((144β^2 )/(β^2 +7))=((100γ^2 )/(γ^2 +7)) find (a_1 )

$${a}_{\mathrm{3}} {x}^{\mathrm{3}} −{x}^{\mathrm{2}} +{a}_{\mathrm{1}} {x}−\mathrm{7}=\mathrm{0}\:{is}\:{a}\:{cubic}\:{polynomial}\:{in}\:{x} \\ $$$${whose}\:{Roots}\:{are}\:\alpha\:,\:\beta\:,\:\gamma\:{positive}\:{real}\:{numbers} \\ $$$${satisfying} \\ $$$$\frac{\mathrm{225}\alpha^{\mathrm{2}} }{\alpha^{\mathrm{2}} +\mathrm{7}}=\frac{\mathrm{144}\beta^{\mathrm{2}} }{\beta^{\mathrm{2}} +\mathrm{7}}=\frac{\mathrm{100}\gamma^{\mathrm{2}} }{\gamma^{\mathrm{2}} +\mathrm{7}} \\ $$$${find}\:\left({a}_{\mathrm{1}} \right) \\ $$

Question Number 195021 Answers: 1 Comments: 0

lim_(x→0) ((x−sin x)/x^n )=¿ (n∈N^∗ )

$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{x}−{sin}\:{x}}{{x}^{{n}} }=¿\:\left({n}\in{N}^{\ast} \right) \\ $$

Question Number 195020 Answers: 1 Comments: 0

y=x^x^(x...) => (dy/dx)=¿

$${y}={x}^{{x}^{{x}...} } \\ $$$$=>\:\frac{{dy}}{{dx}}=¿ \\ $$

Question Number 195017 Answers: 3 Comments: 0

(x+1)^3 =1 x=?

$$\left({x}+\mathrm{1}\right)^{\mathrm{3}} =\mathrm{1}\:\:\:\:\:\:\:\:\:\:{x}=? \\ $$

Question Number 195015 Answers: 1 Comments: 0

Question Number 195013 Answers: 1 Comments: 0

lim_(x→3) (((2((√6)−(√(2x)) +(√(6−2x))))/( (√(36−4x^2 )))) )

$$\:\:\:\:\:\:\underset{\mathrm{x}\rightarrow\mathrm{3}} {\mathrm{lim}}\:\left(\frac{\mathrm{2}\left(\sqrt{\mathrm{6}}−\sqrt{\mathrm{2x}}\:+\sqrt{\mathrm{6}−\mathrm{2x}}\right)}{\:\sqrt{\mathrm{36}−\mathrm{4x}^{\mathrm{2}} }}\:\right) \\ $$

Pg 264 Pg 265 Pg 266 Pg 267 Pg 268 Pg 269 Pg 270 Pg 271 Pg 272 Pg 273

Contact: info@tinkutara.com