Question and Answers Forum

AllQuestion and Answers: Page 240

Question Number 190953 Answers: 1 Comments: 3

Hello Tinku Tara When I copy one qustion or letter from another app I can not to paste in this Math Editor and copy to Latex do not paste in this Math Editor

$$\boldsymbol{\mathrm{Hello}}\:\boldsymbol{\mathrm{Tinku}}\:\boldsymbol{\mathrm{Tara}} \\ $$$$\mathrm{When}\:\mathrm{I}\:\mathrm{copy}\:\mathrm{one}\:\mathrm{qustion}\:\mathrm{or}\: \\ $$$$\mathrm{letter}\:\mathrm{from}\:\mathrm{another}\:\mathrm{app}\:\mathrm{I}\:\mathrm{can} \\ $$$$\mathrm{not}\:\mathrm{to}\:\mathrm{paste}\:\mathrm{in}\:\mathrm{this}\:\mathrm{Math}\:\mathrm{Editor} \\ $$$$\mathrm{and}\:\mathrm{copy}\:\mathrm{to}\:\mathrm{Latex}\:\mathrm{do}\:\mathrm{not}\:\mathrm{paste}\: \\ $$$$\mathrm{in}\:\mathrm{this}\:\mathrm{Math}\:\mathrm{Editor} \\ $$

Question Number 190947 Answers: 1 Comments: 0

Question Number 190942 Answers: 2 Comments: 0

(x+y)(x^2 −xy+y^2 )=2 ((x^3 y^3 )/(x^9 +y^9 −8))=?

$$\left({x}+{y}\right)\left({x}^{\mathrm{2}} −{xy}+{y}^{\mathrm{2}} \right)=\mathrm{2} \\ $$$$\frac{{x}^{\mathrm{3}} {y}^{\mathrm{3}} }{{x}^{\mathrm{9}} +{y}^{\mathrm{9}} −\mathrm{8}}=? \\ $$

Question Number 190940 Answers: 1 Comments: 0

The parametric equation of a curve are x=3t^2 and y=3t−t^2 . Find the volume generated when the plane bounded by the curve ,the x−axis and the ordinates corresponding to t=0 and t=2 rotates about the y−axis

$$\mathrm{The}\:\mathrm{parametric}\:\mathrm{equation}\:\mathrm{of}\:\mathrm{a}\:\mathrm{curve}\:\:\mathrm{are} \\ $$$$\mathrm{x}=\mathrm{3t}^{\mathrm{2}} \:\mathrm{and}\:\mathrm{y}=\mathrm{3t}−\mathrm{t}^{\mathrm{2}} . \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{volume}\:\mathrm{generated} \\ $$$$\mathrm{when}\:\mathrm{the}\:\mathrm{plane}\:\mathrm{bounded}\:\mathrm{by}\:\mathrm{the}\:\mathrm{curve} \\ $$$$,\mathrm{the}\:\mathrm{x}−\mathrm{axis}\:\mathrm{and}\:\mathrm{the}\:\mathrm{ordinates}\: \\ $$$$\mathrm{corresponding}\:\mathrm{to}\: \\ $$$$\mathrm{t}=\mathrm{0}\:\:\:\mathrm{and}\:\mathrm{t}=\mathrm{2}\:\:\mathrm{rotates}\:\mathrm{about}\:\mathrm{the}\:\mathrm{y}−\mathrm{axis} \\ $$

Question Number 190938 Answers: 1 Comments: 0

Question Number 190937 Answers: 1 Comments: 0

Show that ∫ ((sech (√x) tanh (√x))/( (√x)))=−(2/(cosh (√x)))

$$\mathrm{Show}\:\:\mathrm{that}\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\int\:\:\frac{\mathrm{sech}\:\sqrt{\mathrm{x}}\:\mathrm{tanh}\:\sqrt{\mathrm{x}}}{\:\sqrt{\mathrm{x}}}=−\frac{\mathrm{2}}{\mathrm{cosh}\:\sqrt{\mathrm{x}}} \\ $$

Question Number 190936 Answers: 1 Comments: 0

Determine the value of x such that e^(sinh^(−1) x) =1+e^(cosh^(−1) x)

$$\mathrm{Determine}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\mathrm{x}\:\mathrm{such}\:\mathrm{that} \\ $$$$\:\:\:\:\:\:\mathrm{e}^{\mathrm{sinh}\:^{−\mathrm{1}} \mathrm{x}} =\mathrm{1}+\mathrm{e}^{\mathrm{cosh}\:^{−\mathrm{1}} \mathrm{x}} \\ $$

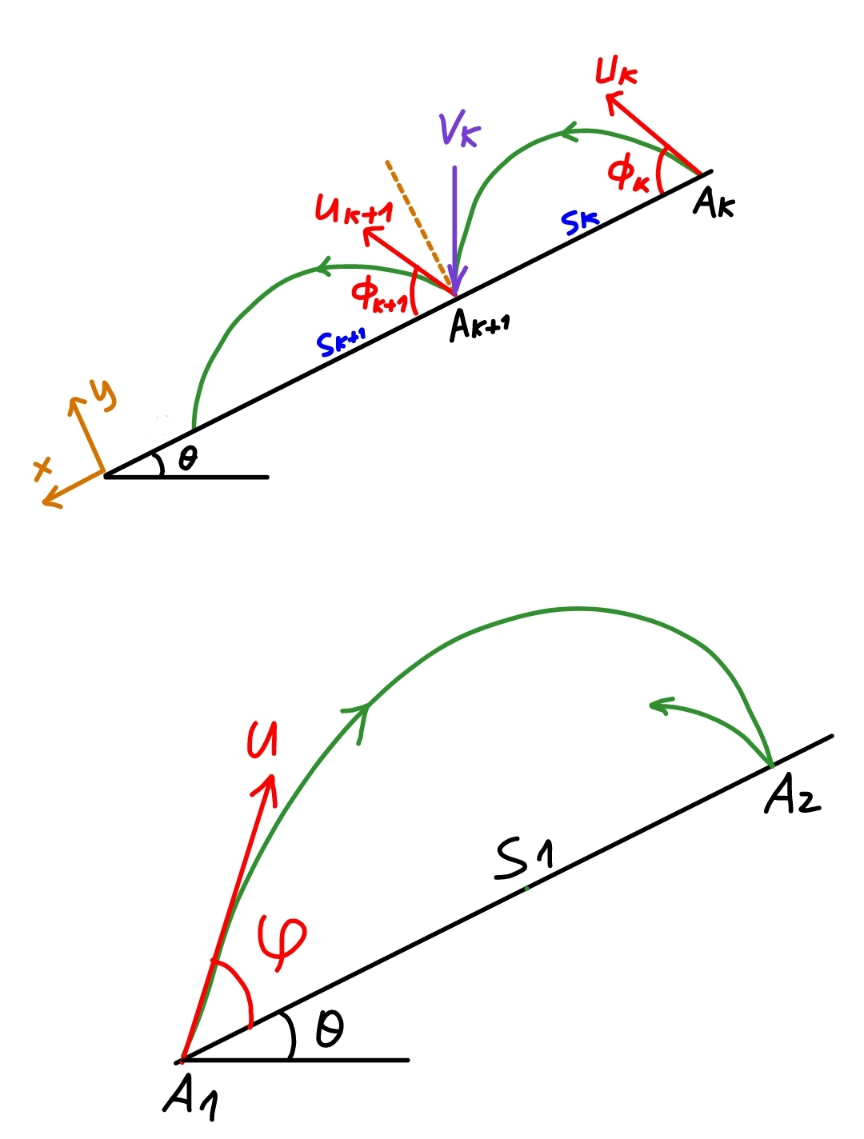
Question Number 190935 Answers: 1 Comments: 0

Given that sinh^(−1) y=sech^(−1) y show that y^2 =(((√5)−1)/2)

$$\mathrm{G}{iven}\:{that}\:\:\:\:\:\:\:\:\mathrm{sinh}\:^{−\mathrm{1}} {y}=\mathrm{sech}\:^{−\mathrm{1}} {y}\:\:\:\: \\ $$$${show}\:{that}\:\:\:\:\:\:\:\:\:{y}^{\mathrm{2}} =\frac{\sqrt{\mathrm{5}}−\mathrm{1}}{\mathrm{2}}\:\:\: \\ $$$$ \\ $$

Question Number 190934 Answers: 1 Comments: 0

Question Number 190927 Answers: 2 Comments: 0

Question Number 190925 Answers: 1 Comments: 1

Question Number 190920 Answers: 0 Comments: 1

solve graphically { ((x−3y<6)),((y≤((3x)/2)+5)) :}

$${solve}\:{graphically}\:\begin{cases}{{x}−\mathrm{3}{y}<\mathrm{6}}\\{{y}\leq\frac{\mathrm{3}{x}}{\mathrm{2}}+\mathrm{5}}\end{cases} \\ $$

Question Number 190916 Answers: 0 Comments: 1

Question Number 190907 Answers: 1 Comments: 0

how is explanotory solution 4^(x^2 +3x+2) +4^(x^2 +3x+7) =4^(2x^2 +6x+9) +1 x=?

$$\mathrm{how}\:\mathrm{is}\:\mathrm{explanotory}\:\mathrm{solution} \\ $$$$\mathrm{4}^{\mathrm{x}^{\mathrm{2}} +\mathrm{3x}+\mathrm{2}} +\mathrm{4}^{\mathrm{x}^{\mathrm{2}} +\mathrm{3x}+\mathrm{7}} =\mathrm{4}^{\mathrm{2x}^{\mathrm{2}} +\mathrm{6x}+\mathrm{9}} +\mathrm{1} \\ $$$$\mathrm{x}=? \\ $$

Question Number 190903 Answers: 1 Comments: 0

Question Number 190902 Answers: 1 Comments: 0

Question Number 190894 Answers: 2 Comments: 0

f(x)= mx + cos(x) , m>1 , f^( −1) (3)= 2f^( −1) (2 ) find : f ( (1/m) )= ?

$$ \\ $$$$\:\:\:\:\:{f}\left({x}\right)=\:{mx}\:+\:{cos}\left({x}\right)\:\:,\:\:{m}>\mathrm{1} \\ $$$$\:\:\:\:,\:\:\:{f}^{\:−\mathrm{1}} \left(\mathrm{3}\right)=\:\mathrm{2}{f}^{\:−\mathrm{1}} \left(\mathrm{2}\:\right) \\ $$$$\:\:\:\:\:{find}\::\:\:\:\:\:\:{f}\:\left(\:\frac{\mathrm{1}}{{m}}\:\right)=\:? \\ $$

Question Number 190893 Answers: 2 Comments: 0

if , ((sin(x)−cos(x))/(sin(x)+cos(x))) = (1/4) ⇒ find the value of F = sin^( 6) (x) + cos^( 6) (x)= ?

$$ \\ $$$$\:\:\:\:\:\:\:\:{if}\:,\:\:\frac{{sin}\left({x}\right)−{cos}\left({x}\right)}{{sin}\left({x}\right)+{cos}\left({x}\right)}\:=\:\frac{\mathrm{1}}{\mathrm{4}} \\ $$$$\:\:\:\:\:\Rightarrow\:{find}\:\:{the}\:{value}\:{of} \\ $$$$\:\:\:\:\:\:\:\:\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\mathrm{F}\:=\:{sin}^{\:\mathrm{6}} \left({x}\right)\:+\:{cos}^{\:\mathrm{6}} \left({x}\right)=\:? \\ $$$$ \\ $$

Question Number 190891 Answers: 2 Comments: 0

(a/b)=(2/3) a+b=?

$$\frac{\mathrm{a}}{\mathrm{b}}=\frac{\mathrm{2}}{\mathrm{3}} \\ $$$$\mathrm{a}+\mathrm{b}=? \\ $$

Question Number 190890 Answers: 0 Comments: 1

Question Number 190883 Answers: 2 Comments: 0

Elementary algebra If , x = ((1 +(√(17)))/2) ⇒ Find the value of : E = (( x^8 − x^( 7) + x^( 6) −....− x +1 )/(x^( 6) − x^( 3) + 1)) = ? @nice − mathematics

$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\mathrm{Elementary}\:\:\:\mathrm{algebra} \\ $$$$\:\:\: \\ $$$$\:\:\:\:\:\:\:\mathrm{If}\:\:,\:\:{x}\:=\:\frac{\mathrm{1}\:+\sqrt{\mathrm{17}}}{\mathrm{2}}\:\:\Rightarrow\:\:\mathrm{Find}\:\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\::\:\:\:\:\:\:\:\: \\ $$$$ \\ $$$$\:\:\:\:\:\:\mathrm{E}\:=\:\frac{\:{x}^{\mathrm{8}} −\:{x}^{\:\mathrm{7}} \:+\:{x}^{\:\mathrm{6}} −....−\:{x}\:+\mathrm{1}\:}{{x}^{\:\mathrm{6}} \:−\:{x}^{\:\mathrm{3}} \:+\:\mathrm{1}}\:=\:? \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:@\mathrm{nice}\:−\:\mathrm{mathematics}\:\:\: \\ $$$$ \\ $$

Question Number 190882 Answers: 1 Comments: 2

Question Number 190878 Answers: 1 Comments: 0

Question Number 190877 Answers: 0 Comments: 2

Question Number 195661 Answers: 1 Comments: 2

Question Number 195660 Answers: 1 Comments: 2

Pg 235 Pg 236 Pg 237 Pg 238 Pg 239 Pg 240 Pg 241 Pg 242 Pg 243 Pg 244

Contact: info@tinkutara.com