Question and Answers Forum

AllQuestion and Answers: Page 238

Question Number 197496 Answers: 2 Comments: 0

lim_(x→3) ((cos3−cosx)/(x−3))=?

$$\underset{{x}\rightarrow\mathrm{3}} {\mathrm{lim}}\frac{{cos}\mathrm{3}−{cosx}}{{x}−\mathrm{3}}=? \\ $$

Question Number 197483 Answers: 1 Comments: 0

lim_(x→−∞) ((tan^(−1) (x))/( (√(1−x)))) =?

$$\:\:\underset{{x}\rightarrow−\infty} {\mathrm{lim}}\:\frac{\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{x}\right)}{\:\sqrt{\mathrm{1}−\mathrm{x}}}\:=? \\ $$

Question Number 197482 Answers: 1 Comments: 0

lim_(x→∞) sin^(−1) (((x^2 (√3) +2)/(2x^2 −3x+1)) )=?

$$\:\:\:\:\:\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\mathrm{sin}^{−\mathrm{1}} \left(\frac{\mathrm{x}^{\mathrm{2}} \sqrt{\mathrm{3}}\:+\mathrm{2}}{\mathrm{2x}^{\mathrm{2}} −\mathrm{3x}+\mathrm{1}}\:\right)=?\: \\ $$

Question Number 197479 Answers: 2 Comments: 1

find: lim_(n→∞) U_n =((n^3 +2n^2 ))^(1/3) −((n^3 −3n^2 ))^(1/3)

$$\boldsymbol{{find}}: \\ $$$$ \\ $$$$\:\:\:\:\:\:\boldsymbol{{li}}\underset{\boldsymbol{{n}}\rightarrow\infty} {\boldsymbol{{m}}}\:\boldsymbol{{U}}_{\boldsymbol{{n}}} \:=\sqrt[{\mathrm{3}}]{\boldsymbol{{n}}^{\mathrm{3}} +\mathrm{2}\boldsymbol{{n}}^{\mathrm{2}} }−\sqrt[{\mathrm{3}}]{\boldsymbol{{n}}^{\mathrm{3}} −\mathrm{3}\boldsymbol{{n}}^{\mathrm{2}} }\: \\ $$

Question Number 197476 Answers: 1 Comments: 7

find the sum (1/(x+1))+(2/(x^2 +1))+(4/(x^4 +1))+.........+(2^n /(x^2^n +1)) = ??

$$ \\ $$$$\:\:\:\mathrm{find}\:\mathrm{the}\:\mathrm{sum} \\ $$$$\:\frac{\mathrm{1}}{{x}+\mathrm{1}}+\frac{\mathrm{2}}{{x}^{\mathrm{2}} +\mathrm{1}}+\frac{\mathrm{4}}{{x}^{\mathrm{4}} +\mathrm{1}}+.........+\frac{\mathrm{2}^{{n}} }{{x}^{\mathrm{2}^{{n}} } +\mathrm{1}}\:\:=\:?? \\ $$

Question Number 197475 Answers: 3 Comments: 0

x , y ∈ R , x^( 2) + xy = 12 y^( 2) + 2xy = 7 −−−−− x , y =?

$$ \\ $$$$\:\:\:\:\:\:{x}\:,\:{y}\:\in\:\mathbb{R}\:\:\:, \\ $$$$\:\:\:\:{x}^{\:\mathrm{2}} \:+\:{xy}\:=\:\mathrm{12} \\ $$$$\:\:\:\:\:{y}^{\:\mathrm{2}} \:+\:\mathrm{2}{xy}\:=\:\mathrm{7}\: \\ $$$$\:\:\:\:−−−−−\:\:\:{x}\:,\:{y}\:=? \\ $$

Question Number 197470 Answers: 2 Comments: 0

Solve the following equation x + 2y + 2z = 0 2x + y − 2z =0 3x + 4y − 6z =0 3x − 11y + 12z = 0

$${Solve}\:{the}\:{following}\:{equation} \\ $$$${x}\:+\:\mathrm{2}{y}\:+\:\mathrm{2}{z}\:=\:\mathrm{0} \\ $$$$\mathrm{2}{x}\:+\:{y}\:−\:\mathrm{2}{z}\:=\mathrm{0} \\ $$$$\mathrm{3}{x}\:+\:\mathrm{4}{y}\:−\:\mathrm{6}{z}\:=\mathrm{0} \\ $$$$\mathrm{3}{x}\:−\:\mathrm{11}{y}\:+\:\mathrm{12}{z}\:=\:\mathrm{0} \\ $$

Question Number 197469 Answers: 0 Comments: 0

solve limits for functions f(x)=cos(sgn(1/x)) f(x)=sgn(cos(1/x))

$$ \\ $$$${solve}\:{limits}\:{for}\:{functions} \\ $$$${f}\left({x}\right)={cos}\left({sgn}\left(\mathrm{1}/{x}\right)\right) \\ $$$${f}\left({x}\right)={sgn}\left({cos}\left(\mathrm{1}/{x}\right)\right) \\ $$$$ \\ $$$$ \\ $$$$ \\ $$

Question Number 197464 Answers: 1 Comments: 0

Question Number 197462 Answers: 0 Comments: 1

Question Number 197459 Answers: 0 Comments: 1

Is complex infinity big? ∞^∼ =∞∙(1+i) Their absolute value is big ∣∞^∼ ∣>∣∞∣

$$\mathrm{Is}\:\mathrm{complex}\:\mathrm{infinity}\:\mathrm{big}? \\ $$$$\overset{\sim} {\infty}=\infty\centerdot\left(\mathrm{1}+{i}\right) \\ $$$$\mathrm{Their}\:\mathrm{absolute}\:\mathrm{value}\:\mathrm{is}\:\mathrm{big} \\ $$$$\mid\overset{\sim} {\infty}\mid>\mid\infty\mid \\ $$

Question Number 197455 Answers: 1 Comments: 0

f(x)=((2x+6)/2)+6−x g(x)=(√(x^(99) +x^(98) +x^(97) +.....+x)) f(g(1))+f(g(2))+.........+f(g(100))=?

$${f}\left({x}\right)=\frac{\mathrm{2}{x}+\mathrm{6}}{\mathrm{2}}+\mathrm{6}−{x} \\ $$$${g}\left({x}\right)=\sqrt{{x}^{\mathrm{99}} +{x}^{\mathrm{98}} +{x}^{\mathrm{97}} +.....+{x}} \\ $$$${f}\left({g}\left(\mathrm{1}\right)\right)+{f}\left({g}\left(\mathrm{2}\right)\right)+.........+{f}\left({g}\left(\mathrm{100}\right)\right)=? \\ $$

Question Number 197452 Answers: 1 Comments: 0

Question Number 197445 Answers: 1 Comments: 0

xe^y y′=2(e^y +x^3 e^(2x) )

$${xe}^{{y}} {y}'=\mathrm{2}\left({e}^{{y}} +{x}^{\mathrm{3}} {e}^{\mathrm{2}{x}} \right) \\ $$

Question Number 197437 Answers: 4 Comments: 0

{ ((2sin (2x+y) sin y = cos 2x)),((sin 2x−sin 2y=(√2))) :} Find the solution

$$\:\:\:\:\begin{cases}{\mathrm{2sin}\:\left(\mathrm{2}{x}+{y}\right)\:\mathrm{sin}\:{y}\:=\:\mathrm{cos}\:\mathrm{2}{x}}\\{\mathrm{sin}\:\mathrm{2}{x}−\mathrm{sin}\:\mathrm{2}{y}=\sqrt{\mathrm{2}}}\end{cases} \\ $$$$\:\:{Find}\:{the}\:{solution} \\ $$

Question Number 197436 Answers: 1 Comments: 0

∫_0 ^(π/2) (dx/(3+tan x)) =?

$$\underset{\mathrm{0}} {\overset{\pi/\mathrm{2}} {\int}}\:\frac{\mathrm{dx}}{\mathrm{3}+\mathrm{tan}\:\mathrm{x}}\:=? \\ $$

Question Number 197431 Answers: 2 Comments: 0

I = ∫_0 ^( ∞) ∫_0 ^( ∞) ∫_0 ^( ∞) ( 1+ x^2 + y^( 2) +z^( 2) )^( −(5/2)) dxdydz=?

$$ \\ $$$$ \\ $$$$\mathrm{I}\:=\:\int_{\mathrm{0}} ^{\:\infty} \int_{\mathrm{0}} ^{\:\infty} \int_{\mathrm{0}} ^{\:\infty} \left(\:\mathrm{1}+\:{x}^{\mathrm{2}} \:+\:{y}^{\:\mathrm{2}} +{z}^{\:\mathrm{2}} \right)^{\:−\frac{\mathrm{5}}{\mathrm{2}}} {dxdydz}=? \\ $$$$ \\ $$

Question Number 197422 Answers: 2 Comments: 0

Question Number 197419 Answers: 1 Comments: 0

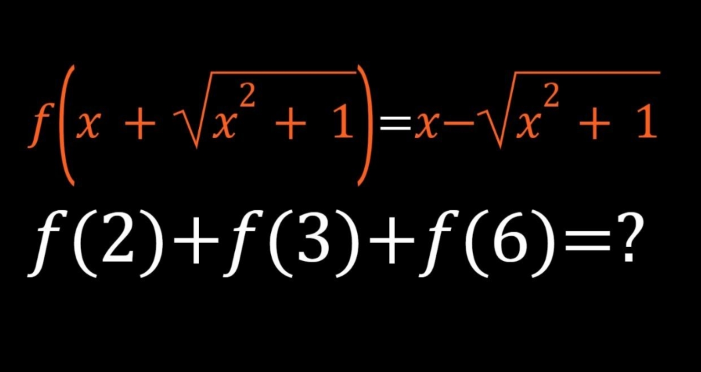
Question Number 197409 Answers: 1 Comments: 4

Question Number 197407 Answers: 2 Comments: 0

lim_(x→∞) ((√(x^2 +1))/(x+1))=?

$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\frac{\sqrt{{x}^{\mathrm{2}} +\mathrm{1}}}{{x}+\mathrm{1}}=? \\ $$

Question Number 197396 Answers: 1 Comments: 1

Question Number 197393 Answers: 1 Comments: 0

Question Number 197389 Answers: 0 Comments: 0

please check my answer (x−2y+5)dx+(2x−y+4)dy=0 X=x+a & Y=y+b (X−a−2Y+2b+5)dX+(2X−2a−Y+b+4)dY=0 -a+2b=-5 -2a+b=-4 a=1,b=-2 (X−2Y)dX+(2X−Y)dY=0 Y=XV⇒(dY/dX)=V+X(dV/dx) (X−2XV)+(2X−XV)(V+X(dV/dX))=0 (2X−XV)(V+X(dV/dX))=-X+2XV (V+X(dV/dX))=((-1+2V)/(2−V)) X(dV/dX)=((-1+2V)/(2−V))−V X(dV/dX)=((-1+2V−2V+V^( 2) )/(2−V)) X(dV/dX)=((V^( 2) −1)/(2−V)) ∫((2−V)/(V^( 2) −1))dV=∫(dX/X) ∫(1/(2(V−1)))+(3/(2(V+1)))dV=ln x+C (1/2)ln(V−1)+(3/2)ln(V+1) (1/2)ln(((y−2)/(x+1))−1)+(3/2)ln(((y−2)/(x+1))+1)=ln x+C

$$ \\ $$$${please}\:{check}\:{my}\:{answer} \\ $$$$\:\left({x}−\mathrm{2}{y}+\mathrm{5}\right){dx}+\left(\mathrm{2}{x}−{y}+\mathrm{4}\right){dy}=\mathrm{0} \\ $$$$\:{X}={x}+{a}\:\&\:{Y}={y}+{b} \\ $$$$ \\ $$$$\:\left({X}−{a}−\mathrm{2}{Y}+\mathrm{2}{b}+\mathrm{5}\right){dX}+\left(\mathrm{2}{X}−\mathrm{2}{a}−{Y}+{b}+\mathrm{4}\right){dY}=\mathrm{0} \\ $$$$\:-{a}+\mathrm{2}{b}=-\mathrm{5} \\ $$$$\:-\mathrm{2}{a}+{b}=-\mathrm{4} \\ $$$$\:{a}=\mathrm{1},{b}=-\mathrm{2} \\ $$$$\:\left({X}−\mathrm{2}{Y}\right){dX}+\left(\mathrm{2}{X}−{Y}\right){dY}=\mathrm{0} \\ $$$$\:{Y}={XV}\Rightarrow\frac{{dY}}{{dX}}={V}+{X}\frac{{dV}}{{dx}} \\ $$$$\:\left({X}−\mathrm{2}{XV}\right)+\left(\mathrm{2}{X}−{XV}\right)\left({V}+{X}\frac{{dV}}{{dX}}\right)=\mathrm{0} \\ $$$$\:\left(\mathrm{2}{X}−{XV}\right)\left({V}+{X}\frac{{dV}}{{dX}}\right)=-{X}+\mathrm{2}{XV} \\ $$$$\:\left({V}+{X}\frac{{dV}}{{dX}}\right)=\frac{-\mathrm{1}+\mathrm{2}{V}}{\mathrm{2}−{V}} \\ $$$$\:{X}\frac{{dV}}{{dX}}=\frac{-\mathrm{1}+\mathrm{2}{V}}{\mathrm{2}−{V}}−{V} \\ $$$$\:{X}\frac{{dV}}{{dX}}=\frac{-\mathrm{1}+\mathrm{2}{V}−\mathrm{2}{V}+{V}^{\:\mathrm{2}} }{\mathrm{2}−{V}} \\ $$$$\:{X}\frac{{dV}}{{dX}}=\frac{{V}^{\:\mathrm{2}} −\mathrm{1}}{\mathrm{2}−{V}} \\ $$$$\:\int\frac{\mathrm{2}−{V}}{{V}^{\:\mathrm{2}} −\mathrm{1}}{dV}=\int\frac{{dX}}{{X}} \\ $$$$\:\int\frac{\mathrm{1}}{\mathrm{2}\left({V}−\mathrm{1}\right)}+\frac{\mathrm{3}}{\mathrm{2}\left({V}+\mathrm{1}\right)}{dV}={ln}\:{x}+{C} \\ $$$$\:\frac{\mathrm{1}}{\mathrm{2}}{ln}\left({V}−\mathrm{1}\right)+\frac{\mathrm{3}}{\mathrm{2}}{ln}\left({V}+\mathrm{1}\right) \\ $$$$\:\frac{\mathrm{1}}{\mathrm{2}}{ln}\left(\frac{{y}−\mathrm{2}}{{x}+\mathrm{1}}−\mathrm{1}\right)+\frac{\mathrm{3}}{\mathrm{2}}{ln}\left(\frac{{y}−\mathrm{2}}{{x}+\mathrm{1}}+\mathrm{1}\right)={ln}\:{x}+{C} \\ $$$$ \\ $$$$ \\ $$

Question Number 197388 Answers: 4 Comments: 0

Question Number 197461 Answers: 1 Comments: 0

Prove that: •∫^( x) _( 0) ((lnt)/(t^2 −1))dt=∫^( (π/2)) _( 0) arctan(xtanθ)dθ • ∫^( x) _( (1/x)) ((lnt)/(t^2 −1))arctant dt=(π/8)∫^( π) _( 0) arctan((1/2)(x−(1/x))sint)dt

$$\mathrm{Prove}\:\mathrm{that}: \\ $$$$\bullet\underset{\:\mathrm{0}} {\int}^{\:\mathrm{x}} \frac{\mathrm{lnt}}{\mathrm{t}^{\mathrm{2}} −\mathrm{1}}\mathrm{dt}=\underset{\:\mathrm{0}} {\int}^{\:\frac{\pi}{\mathrm{2}}} \mathrm{arctan}\left(\mathrm{xtan}\theta\right)\mathrm{d}\theta \\ $$$$\bullet\:\:\underset{\:\frac{\mathrm{1}}{\mathrm{x}}} {\int}^{\:\mathrm{x}} \frac{\mathrm{lnt}}{\mathrm{t}^{\mathrm{2}} −\mathrm{1}}\mathrm{arctant}\:\mathrm{dt}=\frac{\pi}{\mathrm{8}}\underset{\:\mathrm{0}} {\int}^{\:\pi} \mathrm{arctan}\left(\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{x}−\frac{\mathrm{1}}{\mathrm{x}}\right)\mathrm{sint}\right)\mathrm{dt} \\ $$

Pg 233 Pg 234 Pg 235 Pg 236 Pg 237 Pg 238 Pg 239 Pg 240 Pg 241 Pg 242

Contact: info@tinkutara.com