
AllQuestion and Answers: Page 208
Question Number 200048 Answers: 0 Comments: 2
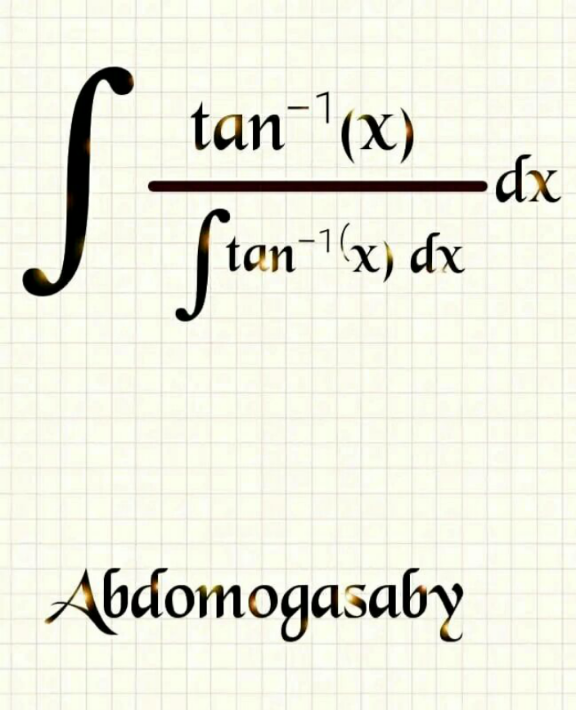
Question Number 200041 Answers: 3 Comments: 0
Question Number 200040 Answers: 2 Comments: 0
Question Number 200035 Answers: 1 Comments: 2

Question Number 200025 Answers: 3 Comments: 0

Question Number 200022 Answers: 0 Comments: 0
Question Number 200019 Answers: 1 Comments: 0

Question Number 200013 Answers: 0 Comments: 3

Question Number 200012 Answers: 0 Comments: 0

Question Number 200053 Answers: 0 Comments: 1

Question Number 200061 Answers: 1 Comments: 0
Question Number 200060 Answers: 2 Comments: 0

Question Number 200005 Answers: 3 Comments: 0

Question Number 200004 Answers: 1 Comments: 0

Question Number 199987 Answers: 1 Comments: 0
Question Number 199986 Answers: 2 Comments: 0
Question Number 199983 Answers: 1 Comments: 0
Question Number 199996 Answers: 0 Comments: 0
Question Number 199968 Answers: 1 Comments: 1
Question Number 199959 Answers: 0 Comments: 4
Question Number 199956 Answers: 1 Comments: 0

Question Number 199952 Answers: 1 Comments: 0

Question Number 199942 Answers: 1 Comments: 0

Question Number 199940 Answers: 2 Comments: 3

Question Number 199934 Answers: 2 Comments: 0
Question Number 199932 Answers: 2 Comments: 0
Pg 203 Pg 204 Pg 205 Pg 206 Pg 207 Pg 208 Pg 209 Pg 210 Pg 211 Pg 212
