Question and Answers Forum

AllQuestion and Answers: Page 207

Question Number 200336 Answers: 1 Comments: 0

Question Number 200330 Answers: 4 Comments: 0

2^x − 3^x = (√(6^x − 9^x )) find: x = ?

$$\mathrm{2}^{\boldsymbol{\mathrm{x}}} \:−\:\mathrm{3}^{\boldsymbol{\mathrm{x}}} \:=\:\sqrt{\mathrm{6}^{\boldsymbol{\mathrm{x}}} \:−\:\mathrm{9}^{\boldsymbol{\mathrm{x}}} } \\ $$$$\mathrm{find}:\:\:\:\mathrm{x}\:=\:? \\ $$

Question Number 200325 Answers: 1 Comments: 0

A point P is taken inside the rectangleC ABD. This point joins the four vertices ofh te rectangle. Knowing that PA is 15 cm. B P 24 cm and PC 20 cm find the distancef o point P from point D.

$$\mathrm{A}\:\mathrm{point}\:\mathrm{P}\:\mathrm{is}\:\mathrm{taken}\:\mathrm{inside}\:\mathrm{the}\:\mathrm{rectangleC} \\ $$$$\mathrm{ABD}.\:\mathrm{This}\:\mathrm{point}\:\mathrm{joins}\:\mathrm{the}\:\mathrm{four}\:\mathrm{vertices}\:\mathrm{ofh} \\ $$$$\mathrm{te}\:\mathrm{rectangle}.\:\:\mathrm{Knowing}\:\mathrm{that}\:\mathrm{PA}\:\mathrm{is}\:\mathrm{15}\:\mathrm{cm}.\:\mathrm{B} \\ $$$$\mathrm{P}\:\:\mathrm{24}\:\mathrm{cm}\:\mathrm{and}\:\mathrm{PC}\:\:\mathrm{20}\:\mathrm{cm}\:\mathrm{find}\:\mathrm{the}\:\mathrm{distancef} \\ $$$$\mathrm{o}\:\mathrm{point}\:\mathrm{P}\:\mathrm{from}\:\mathrm{point}\:\mathrm{D}. \\ $$

Question Number 200321 Answers: 5 Comments: 0

for x,y,z ∈N, if 38x+40y+41z=520 find x+y+z=?

$${for}\:{x},{y},{z}\:\in{N},\:{if} \\ $$$$\mathrm{38}{x}+\mathrm{40}{y}+\mathrm{41}{z}=\mathrm{520} \\ $$$${find}\:{x}+{y}+{z}=? \\ $$

Question Number 200319 Answers: 4 Comments: 0

Question Number 200318 Answers: 2 Comments: 0

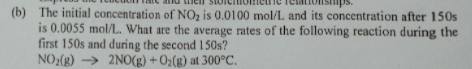
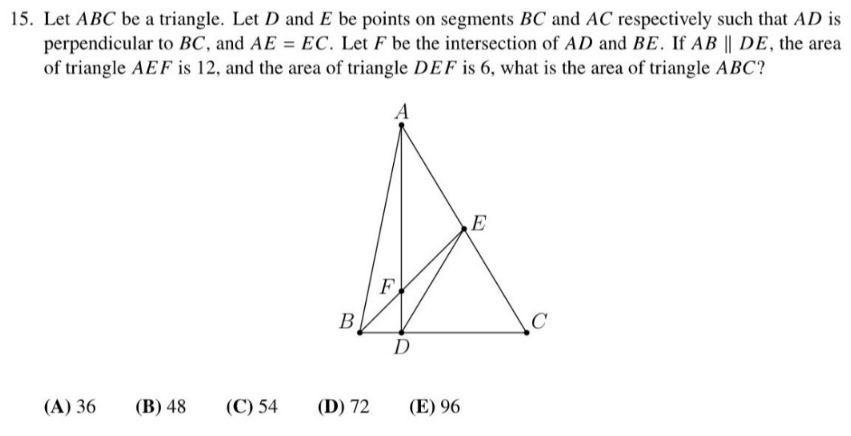
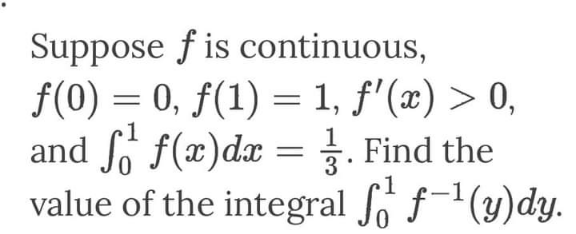
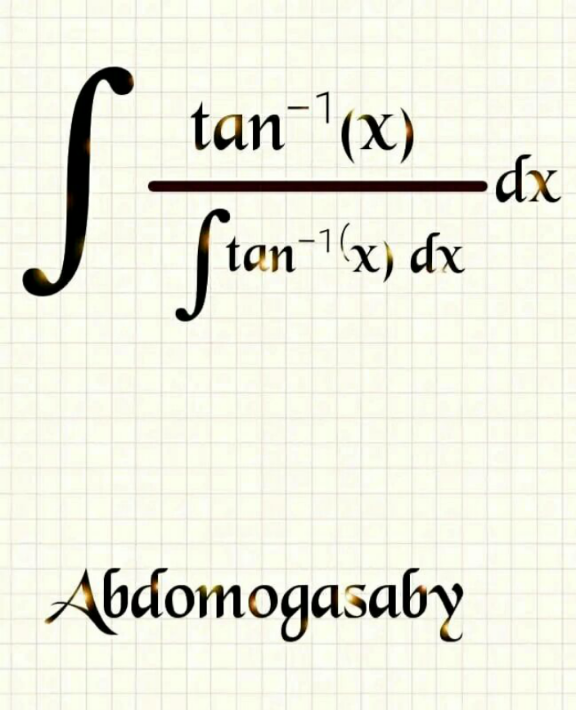
Question Number 200315 Answers: 0 Comments: 3

{ (( ab ^(−) ∙ b ^(−) + ba ^(−) ∙ a ^(−) = cde ^(−) )),(( ab ^(−) ∙ b ^(−) − ba ^(−) ∙ a ^(−) = f ^(−) )) :} a,b,c,d,e,f are all different and in some order consecutive also. Determine the remaining decimal digits.

$$\:\begin{cases}{\overline {\:{ab}\:}\centerdot\overline {\:{b}\:}+\overline {\:{ba}\:}\centerdot\overline {\:{a}\:}=\overline {\:{cde}\:}}\\{\overline {\:{ab}\:}\centerdot\overline {\:{b}\:}−\overline {\:{ba}\:}\centerdot\overline {\:{a}\:}=\overline {\:{f}\:}\:}\end{cases} \\ $$$${a},{b},{c},{d},{e},{f}\:{are}\:{all}\:{different}\:{and}\:{in} \\ $$$${some}\:{order}\:{consecutive}\:{also}. \\ $$$$\: \\ $$$$\mathcal{D}{etermine}\:{the}\:{remaining}\:{decimal} \\ $$$${digits}. \\ $$

Question Number 200275 Answers: 1 Comments: 0