
AllQuestion and Answers: Page 1961
Question Number 10621 Answers: 1 Comments: 0

Question Number 10620 Answers: 1 Comments: 1

Question Number 10612 Answers: 2 Comments: 1

Question Number 10607 Answers: 1 Comments: 0
Question Number 10597 Answers: 1 Comments: 0
Question Number 10583 Answers: 2 Comments: 3
Question Number 10577 Answers: 1 Comments: 0
$$\mathrm{5}^{\mathrm{71}} +\mathrm{5}^{\mathrm{72}} +\mathrm{5}^{\mathrm{73}} =? \\ $$
Question Number 10575 Answers: 1 Comments: 1
Question Number 10572 Answers: 1 Comments: 0
$$\mathrm{factorise} \\ $$$$\mathrm{x}^{\mathrm{3}} \:+\:\mathrm{1} \\ $$
Question Number 10571 Answers: 0 Comments: 0

Question Number 10570 Answers: 0 Comments: 0
Question Number 10566 Answers: 2 Comments: 0
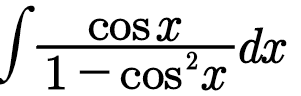
Question Number 10564 Answers: 1 Comments: 0
Question Number 10563 Answers: 0 Comments: 0
Question Number 10562 Answers: 0 Comments: 0
Question Number 10555 Answers: 0 Comments: 0
Question Number 10553 Answers: 0 Comments: 0
Question Number 11178 Answers: 0 Comments: 1
Question Number 11172 Answers: 1 Comments: 0
Question Number 11183 Answers: 1 Comments: 0

Question Number 10547 Answers: 1 Comments: 0
Question Number 11206 Answers: 3 Comments: 0

Question Number 11204 Answers: 0 Comments: 0

Question Number 11203 Answers: 1 Comments: 0
Question Number 11196 Answers: 0 Comments: 2
Question Number 10544 Answers: 0 Comments: 1
Pg 1956 Pg 1957 Pg 1958 Pg 1959 Pg 1960 Pg 1961 Pg 1962 Pg 1963 Pg 1964 Pg 1965
