
AllQuestion and Answers: Page 1950
Question Number 12093 Answers: 1 Comments: 0
Question Number 12092 Answers: 0 Comments: 0
Question Number 12087 Answers: 1 Comments: 0
Question Number 12085 Answers: 2 Comments: 0

Question Number 12082 Answers: 1 Comments: 0

Question Number 12080 Answers: 1 Comments: 0

Question Number 12078 Answers: 1 Comments: 0
Question Number 12077 Answers: 1 Comments: 0
Question Number 12074 Answers: 1 Comments: 0

Question Number 12071 Answers: 0 Comments: 0

Question Number 12064 Answers: 0 Comments: 0
Question Number 12063 Answers: 1 Comments: 0
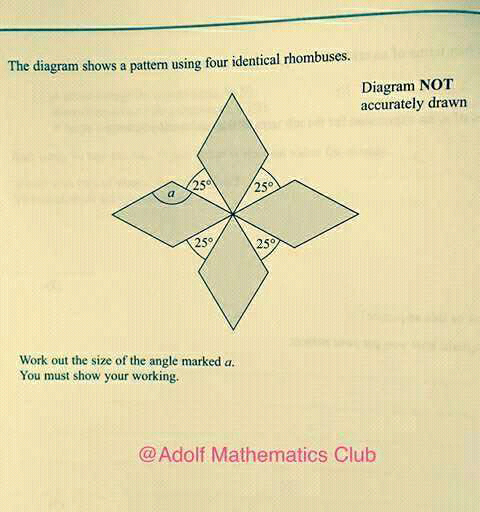
Question Number 12062 Answers: 2 Comments: 0
Question Number 12047 Answers: 1 Comments: 1
Question Number 12046 Answers: 1 Comments: 0
Question Number 12045 Answers: 1 Comments: 0
Question Number 12044 Answers: 1 Comments: 0
Question Number 12326 Answers: 1 Comments: 0
Question Number 12040 Answers: 2 Comments: 0
Question Number 12030 Answers: 1 Comments: 0

Question Number 12028 Answers: 1 Comments: 0
Question Number 12023 Answers: 3 Comments: 0
Question Number 12019 Answers: 1 Comments: 0

Question Number 12018 Answers: 0 Comments: 0
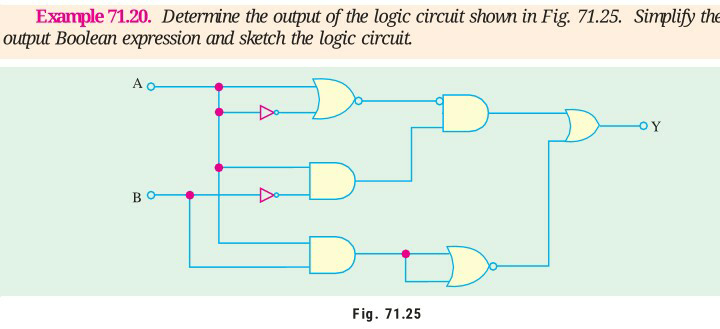
Question Number 12017 Answers: 0 Comments: 0
Question Number 12015 Answers: 1 Comments: 0
$${prove}\:{that}\:{for}\:{all}\:{x}\:\in{R}, \\ $$$${e}^{{x}} \geqslant{x}^{{e}} \\ $$
Pg 1945 Pg 1946 Pg 1947 Pg 1948 Pg 1949 Pg 1950 Pg 1951 Pg 1952 Pg 1953 Pg 1954
