Question and Answers Forum

AllQuestion and Answers: Page 1939

Question Number 12830 Answers: 1 Comments: 0

the LCM and HCF of 30 and a certain number are 150 and 5 respectively. find the number please help

$$\mathrm{the}\:\mathrm{LCM}\:\mathrm{and}\:\mathrm{HCF}\:\mathrm{of}\:\mathrm{30}\:\mathrm{and}\:\mathrm{a}\: \\ $$$$\mathrm{certain}\:\mathrm{number}\:\mathrm{are}\:\mathrm{150}\:\mathrm{and}\:\mathrm{5}\: \\ $$$$\mathrm{respectively}.\:\mathrm{find}\:\mathrm{the}\:\mathrm{number} \\ $$$$ \\ $$$$ \\ $$$$\mathrm{please}\:\mathrm{help} \\ $$

Question Number 12829 Answers: 2 Comments: 0

Question Number 12828 Answers: 1 Comments: 0

The value of the infinite product (√3) ∙ (9)^(1/4) ∙ ((27))^(1/8) ∙ ((81))^(1/(16)) ...to ∞ is equal to ____.

$$\mathrm{The}\:\mathrm{value}\:\mathrm{of}\:\mathrm{the}\:\mathrm{infinite}\:\mathrm{product} \\ $$$$\sqrt{\mathrm{3}}\:\centerdot\:\sqrt[{\mathrm{4}}]{\mathrm{9}}\:\centerdot\:\sqrt[{\mathrm{8}}]{\mathrm{27}}\:\centerdot\:\sqrt[{\mathrm{16}}]{\mathrm{81}}\:...\mathrm{to}\:\infty\:\:\mathrm{is}\:\mathrm{equal}\:\mathrm{to}\:\_\_\_\_. \\ $$

Question Number 12827 Answers: 2 Comments: 0

Sum of three numbers in GP be 14. If one is added to first and second and 1 is subtracted from the third, the new numbers are in AP. The smallest of them is

$$\mathrm{Sum}\:\mathrm{of}\:\mathrm{three}\:\mathrm{numbers}\:\mathrm{in}\:\mathrm{GP}\:\mathrm{be}\:\mathrm{14}.\:\mathrm{If}\:\mathrm{one}\:\mathrm{is} \\ $$$$\mathrm{added}\:\mathrm{to}\:\mathrm{first}\:\mathrm{and}\:\mathrm{second}\:\mathrm{and}\:\mathrm{1}\:\mathrm{is}\:\mathrm{subtracted} \\ $$$$\mathrm{from}\:\mathrm{the}\:\mathrm{third},\:\mathrm{the}\:\mathrm{new}\:\mathrm{numbers}\:\mathrm{are}\:\mathrm{in}\:\mathrm{AP}. \\ $$$$\mathrm{The}\:\mathrm{smallest}\:\mathrm{of}\:\mathrm{them}\:\mathrm{is} \\ $$

Question Number 12826 Answers: 0 Comments: 0

prove that 1: 0<∫_0 ^(π/4) x(√(tan x)) dx< (π^2 /(32)) 2: (1/2)<∫_(π/4) ^(π/2) ((sin x)/x) dx <((√2)/2) 3: 0<∫_(100π) ^(200π) ((cos x)/x) dx <(1/(100π))

$$\mathrm{prove}\:\mathrm{that} \\ $$$$\mathrm{1}:\:\:\mathrm{0}<\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\mathrm{x}\sqrt{\mathrm{tan}\:\mathrm{x}}\:\mathrm{dx}<\:\frac{\pi^{\mathrm{2}} }{\mathrm{32}} \\ $$$$\mathrm{2}:\:\frac{\mathrm{1}}{\mathrm{2}}<\int_{\frac{\pi}{\mathrm{4}}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{\mathrm{sin}\:\mathrm{x}}{\mathrm{x}}\:\mathrm{dx}\:<\frac{\sqrt{\mathrm{2}}}{\mathrm{2}} \\ $$$$\mathrm{3}:\:\mathrm{0}<\int_{\mathrm{100}\pi} ^{\mathrm{200}\pi} \:\frac{\mathrm{cos}\:\mathrm{x}}{\mathrm{x}}\:\mathrm{dx}\:<\frac{\mathrm{1}}{\mathrm{100}\pi} \\ $$

Question Number 12822 Answers: 1 Comments: 0

prove by contradiction 9+13(√(3 )) is irrational

$${prove}\:{by}\:{contradiction}\:\mathrm{9}+\mathrm{13}\sqrt{\mathrm{3}\:} \\ $$$${is}\:{irrational} \\ $$

Question Number 12814 Answers: 1 Comments: 2

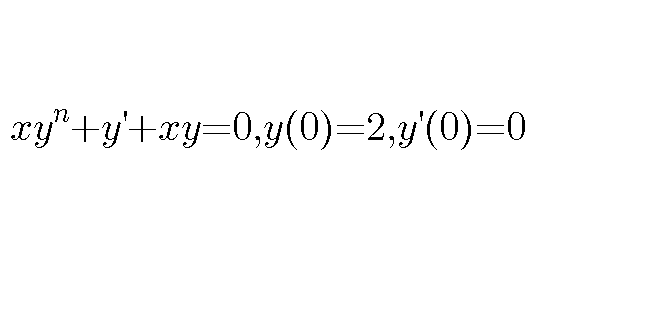
Question Number 12812 Answers: 1 Comments: 0

Question Number 12804 Answers: 1 Comments: 3

Question Number 12801 Answers: 1 Comments: 0

Question Number 12797 Answers: 1 Comments: 2

1 + x + x^2 + ... x^(49) = (1/2)(x^(49) − (1/x)) Find the value of x

$$\mathrm{1}\:+\:\mathrm{x}\:+\:\mathrm{x}^{\mathrm{2}} \:+\:...\:\mathrm{x}^{\mathrm{49}} \:=\:\frac{\mathrm{1}}{\mathrm{2}}\left(\mathrm{x}^{\mathrm{49}} \:−\:\frac{\mathrm{1}}{\mathrm{x}}\right) \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\mathrm{x} \\ $$

Question Number 12796 Answers: 1 Comments: 0

The sum of two positive numbers is 20. find the numbers (i) If their product is maximum (ii) If the sum of their square is maximum (iii) If the product of the square of one and the cube of the other is maximum

$$\mathrm{The}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{two}\:\mathrm{positive}\:\:\mathrm{numbers}\:\mathrm{is}\:\mathrm{20}.\:\mathrm{find}\:\mathrm{the}\:\mathrm{numbers} \\ $$$$\left(\mathrm{i}\right)\:\:\mathrm{If}\:\mathrm{their}\:\mathrm{product}\:\mathrm{is}\:\mathrm{maximum} \\ $$$$\left(\mathrm{ii}\right)\:\:\mathrm{If}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{their}\:\mathrm{square}\:\mathrm{is}\:\mathrm{maximum} \\ $$$$\left(\mathrm{iii}\right)\:\mathrm{If}\:\mathrm{the}\:\mathrm{product}\:\mathrm{of}\:\mathrm{the}\:\mathrm{square}\:\mathrm{of}\:\mathrm{one}\:\mathrm{and}\:\mathrm{the}\:\mathrm{cube}\:\mathrm{of}\:\mathrm{the}\:\mathrm{other}\:\mathrm{is}\:\mathrm{maximum} \\ $$

Question Number 12794 Answers: 0 Comments: 0

Let R be a cummutative ring with 1, and a,b∈R. suppose a is ivertible and b is nilpotent. Show that a + b is ivertible.

$$\mathrm{Let}\:\mathrm{R}\:\mathrm{be}\:\mathrm{a}\:\mathrm{cummutative}\:\mathrm{ring}\:\mathrm{with}\:\mathrm{1},\:\mathrm{and}\:\:\mathrm{a},\mathrm{b}\in\mathrm{R}.\:\mathrm{suppose}\:\mathrm{a}\:\mathrm{is}\:\mathrm{ivertible}\:\mathrm{and} \\ $$$$\mathrm{b}\:\mathrm{is}\:\mathrm{nilpotent}.\:\mathrm{Show}\:\mathrm{that}\:\:\mathrm{a}\:+\:\mathrm{b}\:\:\mathrm{is}\:\mathrm{ivertible}. \\ $$

Question Number 12773 Answers: 1 Comments: 0

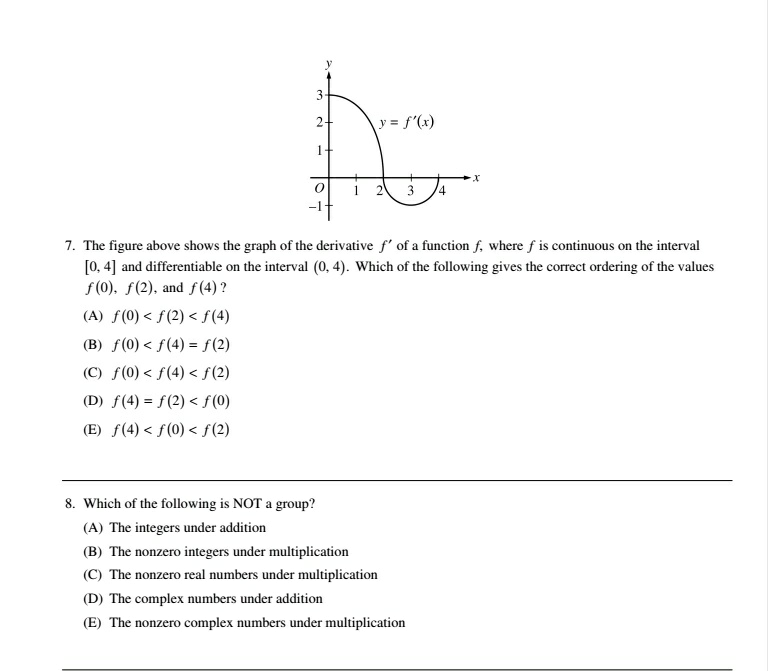
Question Number 12768 Answers: 1 Comments: 0

∫ ((sec x)/(tan^2 x)) dx

$$\int\:\frac{\mathrm{sec}\:{x}}{\mathrm{tan}^{\mathrm{2}} \:{x}}\:{dx} \\ $$

Question Number 12766 Answers: 1 Comments: 0

∫ (dx/(1 + tan x))

$$\int\:\frac{{dx}}{\mathrm{1}\:+\:\mathrm{tan}\:{x}} \\ $$

Question Number 12763 Answers: 0 Comments: 0

Solve simultaneously 2x + y − z = 8 ........... equation (i) x^2 − y^2 + 2z^2 = 14 .......... equation (ii) 3x^3 + 4y^3 + z^3 = 195 ........... equation (iii)

$$\mathrm{Solve}\:\mathrm{simultaneously} \\ $$$$\mathrm{2x}\:+\:\mathrm{y}\:−\:\mathrm{z}\:=\:\mathrm{8}\:\:\:\:\:\:\:\:...........\:\mathrm{equation}\:\left(\mathrm{i}\right) \\ $$$$\mathrm{x}^{\mathrm{2}} \:−\:\mathrm{y}^{\mathrm{2}} \:+\:\mathrm{2z}^{\mathrm{2}} \:=\:\mathrm{14}\:\:\:\:\:\:\:..........\:\mathrm{equation}\:\left(\mathrm{ii}\right) \\ $$$$\mathrm{3x}^{\mathrm{3}} \:+\:\mathrm{4y}^{\mathrm{3}} \:+\:\mathrm{z}^{\mathrm{3}} \:=\:\mathrm{195}\:\:\:\:\:\:\:\:\:...........\:\mathrm{equation}\:\left(\mathrm{iii}\right) \\ $$

Question Number 12760 Answers: 2 Comments: 0

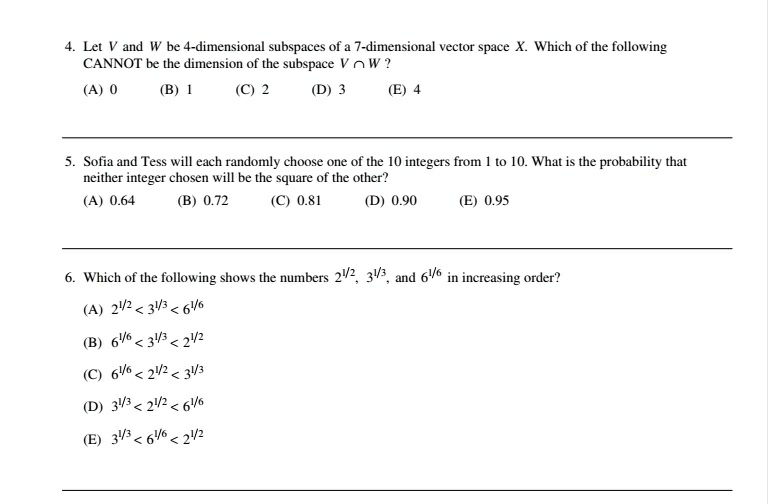
Question Number 12753 Answers: 1 Comments: 0

evaluate ∫(√((sin x)))dx

$${evaluate}\:\int\sqrt{\left(\mathrm{sin}\:{x}\right)}{dx} \\ $$

Question Number 12752 Answers: 0 Comments: 0

Let V and W be 4 dimensional subspaces of a 7 dimensional vector space X. Which of the following CANNOT be the dimension of the subspace V∩W. (A) 0 (B) 1 (C) 2 (D) 3 (E) 4

$$\mathrm{Let}\:\mathrm{V}\:\mathrm{and}\:\mathrm{W}\:\mathrm{be}\:\mathrm{4}\:\mathrm{dimensional}\:\mathrm{subspaces}\:\mathrm{of}\:\mathrm{a}\:\mathrm{7}\:\mathrm{dimensional}\:\mathrm{vector}\:\mathrm{space}\:\mathrm{X}. \\ $$$$\mathrm{Which}\:\mathrm{of}\:\mathrm{the}\:\mathrm{following}\:\mathrm{CANNOT}\:\mathrm{be}\:\mathrm{the}\:\mathrm{dimension}\:\mathrm{of}\:\mathrm{the}\:\mathrm{subspace}\:\mathrm{V}\cap\mathrm{W}. \\ $$$$\left(\mathrm{A}\right)\:\mathrm{0}\:\left(\mathrm{B}\right)\:\mathrm{1}\:\left(\mathrm{C}\right)\:\mathrm{2}\:\left(\mathrm{D}\right)\:\mathrm{3}\:\left(\mathrm{E}\right)\:\mathrm{4} \\ $$

Question Number 12744 Answers: 1 Comments: 0

∫_( e^(−3) ) ^( e^(−2) ) (1/((x)log(x))) dx = ?

$$\int_{\:\mathrm{e}^{−\mathrm{3}} } ^{\:\mathrm{e}^{−\mathrm{2}} } \:\:\frac{\mathrm{1}}{\left(\mathrm{x}\right)\mathrm{log}\left(\mathrm{x}\right)}\:\mathrm{dx}\:\:=\:\:? \\ $$

Question Number 12743 Answers: 2 Comments: 0

What is the area of eqilateral triangle whose inscribed circle has a radius 2

$$\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{area}\:\mathrm{of}\:\mathrm{eqilateral}\:\mathrm{triangle}\:\mathrm{whose}\:\mathrm{inscribed}\:\mathrm{circle}\:\mathrm{has}\:\mathrm{a}\:\mathrm{radius}\:\mathrm{2} \\ $$

Question Number 12742 Answers: 1 Comments: 0

Prove that ∫ (dx/((x +1)^2 (√(x^2 + 2x +2)))) = ((−(√(x^2 + 2x + 2)))/(x + 1)) + C

$$\mathrm{Prove}\:\mathrm{that} \\ $$$$\int\:\frac{{dx}}{\left({x}\:+\mathrm{1}\right)^{\mathrm{2}} \:\sqrt{{x}^{\mathrm{2}} \:+\:\mathrm{2}{x}\:+\mathrm{2}}}\:=\:\frac{−\sqrt{{x}^{\mathrm{2}} \:+\:\mathrm{2}{x}\:+\:\mathrm{2}}}{{x}\:+\:\mathrm{1}}\:+\:{C} \\ $$

Question Number 12740 Answers: 1 Comments: 0

∫∣x∣ dx