
AllQuestion and Answers: Page 1936
Question Number 13727 Answers: 1 Comments: 1
Question Number 13725 Answers: 0 Comments: 2
Question Number 13724 Answers: 2 Comments: 3
Question Number 13721 Answers: 0 Comments: 0
Question Number 13706 Answers: 1 Comments: 0
Question Number 13705 Answers: 1 Comments: 3
Question Number 13695 Answers: 1 Comments: 2
Question Number 13688 Answers: 1 Comments: 0

Question Number 13687 Answers: 0 Comments: 4
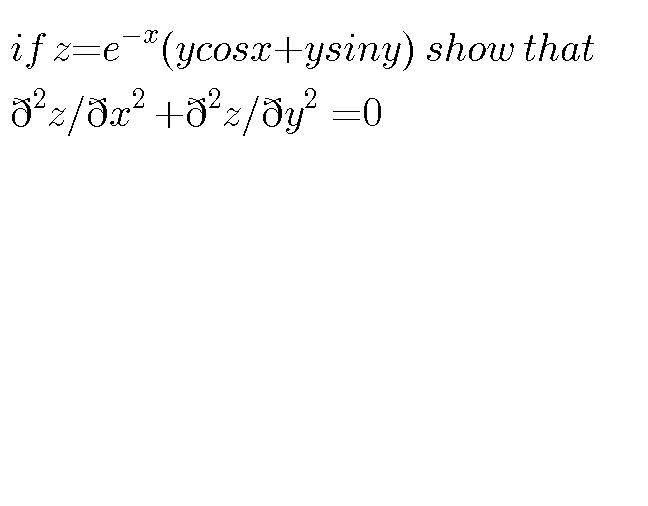
Question Number 13681 Answers: 1 Comments: 3

Question Number 13658 Answers: 1 Comments: 0
Question Number 13654 Answers: 1 Comments: 0
Question Number 13649 Answers: 1 Comments: 0
$$\mathrm{2}{xyy}'+\left({x}−\mathrm{1}\right){y}^{\mathrm{2}} ={x}^{\mathrm{2}} {e}^{{x}} \\ $$
Question Number 13647 Answers: 0 Comments: 6
Question Number 13645 Answers: 0 Comments: 0
Question Number 13636 Answers: 1 Comments: 0
Question Number 13627 Answers: 1 Comments: 0

Question Number 13626 Answers: 1 Comments: 0
Question Number 13609 Answers: 1 Comments: 0

Question Number 13608 Answers: 0 Comments: 2
Question Number 13606 Answers: 1 Comments: 0
Question Number 13732 Answers: 1 Comments: 3
Question Number 13601 Answers: 1 Comments: 0
Question Number 13622 Answers: 1 Comments: 0
Question Number 13623 Answers: 1 Comments: 0
Question Number 13598 Answers: 0 Comments: 1
Pg 1931 Pg 1932 Pg 1933 Pg 1934 Pg 1935 Pg 1936 Pg 1937 Pg 1938 Pg 1939 Pg 1940
