
AllQuestion and Answers: Page 1935
Question Number 13977 Answers: 2 Comments: 1

Question Number 13976 Answers: 1 Comments: 0
Question Number 13965 Answers: 1 Comments: 0
Question Number 13960 Answers: 1 Comments: 0
Question Number 13955 Answers: 0 Comments: 2
Question Number 13942 Answers: 1 Comments: 0
Question Number 13934 Answers: 0 Comments: 0

Question Number 13929 Answers: 1 Comments: 0
Question Number 13909 Answers: 4 Comments: 5
Question Number 13904 Answers: 2 Comments: 0
Question Number 13903 Answers: 2 Comments: 1
Question Number 13893 Answers: 0 Comments: 3
Question Number 13891 Answers: 0 Comments: 1
Question Number 13875 Answers: 0 Comments: 0

Question Number 13872 Answers: 1 Comments: 0
Question Number 13871 Answers: 0 Comments: 0
$${why}\:{the}\:{function},{sin}\left({x}\right)\:{is}\:{a}\:{power} \\ $$$${series}?? \\ $$
Question Number 13842 Answers: 3 Comments: 0
Question Number 13840 Answers: 1 Comments: 0
Question Number 13849 Answers: 0 Comments: 2
Question Number 13835 Answers: 1 Comments: 1

Question Number 13830 Answers: 1 Comments: 0
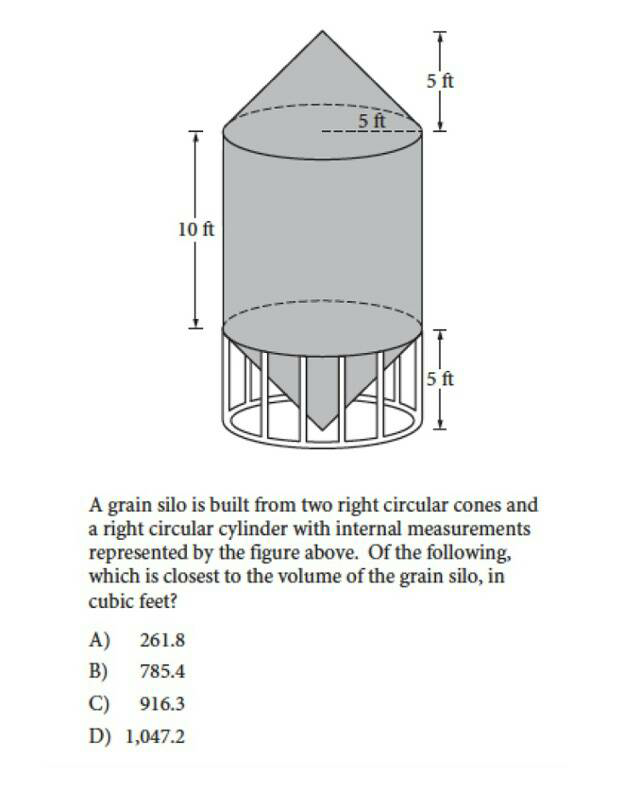
Question Number 13831 Answers: 1 Comments: 0

Question Number 13832 Answers: 1 Comments: 0

Question Number 13812 Answers: 1 Comments: 0
Question Number 13811 Answers: 1 Comments: 1
Question Number 13808 Answers: 1 Comments: 0
Pg 1930 Pg 1931 Pg 1932 Pg 1933 Pg 1934 Pg 1935 Pg 1936 Pg 1937 Pg 1938 Pg 1939
