Question and Answers Forum

AllQuestion and Answers: Page 1927

Question Number 15384 Answers: 1 Comments: 0

If a flagstaff subtends equal angles at 4 points A, B, C and D on the horizontal plane through the foot of the flagstaff, then A, B, C and D must be the vertices of (1) Square (2) Cyclic quadrilateral (3) Rectangle (4) Parallelogram

$$\mathrm{If}\:\mathrm{a}\:\mathrm{flagstaff}\:\mathrm{subtends}\:\mathrm{equal}\:\mathrm{angles}\:\mathrm{at}\:\mathrm{4} \\ $$$$\mathrm{points}\:{A},\:{B},\:{C}\:\mathrm{and}\:{D}\:\mathrm{on}\:\mathrm{the}\:\mathrm{horizontal} \\ $$$$\mathrm{plane}\:\mathrm{through}\:\mathrm{the}\:\mathrm{foot}\:\mathrm{of}\:\mathrm{the}\:\mathrm{flagstaff}, \\ $$$$\mathrm{then}\:{A},\:{B},\:{C}\:\mathrm{and}\:{D}\:\mathrm{must}\:\mathrm{be}\:\mathrm{the} \\ $$$$\mathrm{vertices}\:\mathrm{of} \\ $$$$\left(\mathrm{1}\right)\:\mathrm{Square} \\ $$$$\left(\mathrm{2}\right)\:\mathrm{Cyclic}\:\mathrm{quadrilateral} \\ $$$$\left(\mathrm{3}\right)\:\mathrm{Rectangle} \\ $$$$\left(\mathrm{4}\right)\:\mathrm{Parallelogram} \\ $$

Question Number 15418 Answers: 2 Comments: 0

A grasshopper can jump a maximum horizontal distance of 40 cm. If it spends negligible time on the ground then in this case its speed along the horizontal road will be?

$$\mathrm{A}\:\mathrm{grasshopper}\:\mathrm{can}\:\mathrm{jump}\:\mathrm{a}\:\mathrm{maximum} \\ $$$$\mathrm{horizontal}\:\mathrm{distance}\:\mathrm{of}\:\mathrm{40}\:\mathrm{cm}.\:\mathrm{If}\:\mathrm{it} \\ $$$$\mathrm{spends}\:\mathrm{negligible}\:\mathrm{time}\:\mathrm{on}\:\mathrm{the}\:\mathrm{ground} \\ $$$$\mathrm{then}\:\mathrm{in}\:\mathrm{this}\:\mathrm{case}\:\mathrm{its}\:\mathrm{speed}\:\mathrm{along}\:\mathrm{the} \\ $$$$\mathrm{horizontal}\:\mathrm{road}\:\mathrm{will}\:\mathrm{be}? \\ $$

Question Number 15358 Answers: 1 Comments: 0

If a^→ , b^→ , c^→ are mutually perpendicular vectors of equal magnitudes, show that the vector a^→ + b^→ + c^→ is equally inclined to a^→ , b^→ and c^→ .

Question Number 15352 Answers: 0 Comments: 4

Let a^→ = i^∧ + 4j^∧ + 2k^∧ , b^→ = 3i^∧ − 2j^∧ + 7k^∧ and c^→ = 2i^∧ − j^∧ + 4k^∧ . Find a vector d^→ which is perpendicular to both a^→ and b^→ , and c^→ ∙ d^→ = 15.

Question Number 15348 Answers: 2 Comments: 0

Solve the equation z^2 + 2(1 + j)z + 2 = 0, Givin each result in form a + jb with a and b correct to 2dp.

$$\mathrm{Solve}\:\mathrm{the}\:\mathrm{equation}\:\:\mathrm{z}^{\mathrm{2}} \:+\:\mathrm{2}\left(\mathrm{1}\:+\:\mathrm{j}\right)\mathrm{z}\:+\:\mathrm{2}\:=\:\mathrm{0},\:\mathrm{Givin}\:\mathrm{each}\:\mathrm{result}\:\mathrm{in}\:\mathrm{form}\:\:\mathrm{a}\:+\:\mathrm{jb} \\ $$$$\mathrm{with}\:\:\mathrm{a}\:\:\mathrm{and}\:\:\mathrm{b}\:\:\mathrm{correct}\:\mathrm{to}\:\:\mathrm{2dp}. \\ $$

Question Number 15343 Answers: 0 Comments: 0

Question Number 15344 Answers: 0 Comments: 0

Question Number 15328 Answers: 1 Comments: 0

Prove that. sec^4 (x) − cosec^4 (x) = ((sin^2 (x) − cos^2 (x))/(sec^4 (x)))

$$\mathrm{Prove}\:\mathrm{that}. \\ $$$$\mathrm{sec}^{\mathrm{4}} \left(\mathrm{x}\right)\:−\:\mathrm{cosec}^{\mathrm{4}} \left(\mathrm{x}\right)\:=\:\frac{\mathrm{sin}^{\mathrm{2}} \left(\mathrm{x}\right)\:−\:\mathrm{cos}^{\mathrm{2}} \left(\mathrm{x}\right)}{\mathrm{sec}^{\mathrm{4}} \left(\mathrm{x}\right)} \\ $$

Question Number 15322 Answers: 0 Comments: 6

Question Number 15318 Answers: 2 Comments: 6

Question Number 15311 Answers: 0 Comments: 0

In a toll-booth at a bridge , some cars can pass by paying a tax of Rs. 10 and some special vehicles are exempted from paying tax. The booth has to track number of vehicles and total tax collected. Define a class ′tollbooth′. It should contain two data items of type int to hold the number of cars and total tax connected. A constructor initalizes these two variable to zero. A memberfunction freecar() only increments the car total. Finally another memberfunction show() displays the two totals. Write a C++ program such that the user has to press the key ′T′ for printing number of taxable cars and total tax, ′F′ for printing number of free cars and ′Esc′ to exit.

$$\mathrm{In}\:\mathrm{a}\:\mathrm{toll}-\mathrm{booth}\:\mathrm{at}\:\mathrm{a}\:\mathrm{bridge}\:,\:\mathrm{some}\:\mathrm{cars}\:\mathrm{can}\:\mathrm{pass}\:\mathrm{by}\:\mathrm{paying}\:\mathrm{a}\:\mathrm{tax}\:\mathrm{of}\:\mathrm{Rs}.\:\mathrm{10}\:\mathrm{and} \\ $$$$\mathrm{some}\:\mathrm{special}\:\mathrm{vehicles}\:\mathrm{are}\:\mathrm{exempted}\:\mathrm{from}\:\mathrm{paying}\:\mathrm{tax}.\:\mathrm{The}\:\mathrm{booth}\:\mathrm{has}\:\mathrm{to}\:\mathrm{track}\: \\ $$$$\mathrm{number}\:\mathrm{of}\:\mathrm{vehicles}\:\mathrm{and}\:\mathrm{total}\:\mathrm{tax}\:\mathrm{collected}.\:\mathrm{Define}\:\mathrm{a}\:\mathrm{class}\:'\mathrm{tollbooth}'.\:\mathrm{It}\:\mathrm{should}\:\mathrm{contain} \\ $$$$\mathrm{two}\:\mathrm{data}\:\mathrm{items}\:\mathrm{of}\:\mathrm{type}\:\mathrm{int}\:\mathrm{to}\:\mathrm{hold}\:\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{cars}\:\mathrm{and}\:\mathrm{total}\:\mathrm{tax}\:\mathrm{connected}. \\ $$$$\mathrm{A}\:\mathrm{constructor}\:\mathrm{initalizes}\:\mathrm{these}\:\mathrm{two}\:\mathrm{variable}\:\mathrm{to}\:\mathrm{zero}.\:\mathrm{A}\:\mathrm{memberfunction}\:\mathrm{freecar}\left(\right)\:\mathrm{only} \\ $$$$\mathrm{increments}\:\mathrm{the}\:\mathrm{car}\:\mathrm{total}.\:\mathrm{Finally}\:\mathrm{another}\:\mathrm{memberfunction}\:\mathrm{show}\left(\right)\:\mathrm{displays}\:\mathrm{the} \\ $$$$\mathrm{two}\:\mathrm{totals}. \\ $$$$\:\:\:\mathrm{Write}\:\mathrm{a}\:\mathrm{C}++\:\mathrm{program}\:\mathrm{such}\:\mathrm{that}\:\mathrm{the}\:\mathrm{user}\:\mathrm{has}\:\mathrm{to}\:\mathrm{press}\:\mathrm{the}\:\mathrm{key}\:'\mathrm{T}'\:\mathrm{for} \\ $$$$\mathrm{printing}\:\mathrm{number}\:\mathrm{of}\:\mathrm{taxable}\:\mathrm{cars}\:\mathrm{and}\:\mathrm{total}\:\mathrm{tax},\:'\mathrm{F}'\:\mathrm{for}\:\mathrm{printing}\:\mathrm{number}\:\mathrm{of}\:\:\mathrm{free} \\ $$$$\mathrm{cars}\:\mathrm{and}\:'\mathrm{Esc}'\:\mathrm{to}\:\mathrm{exit}. \\ $$

Question Number 15302 Answers: 0 Comments: 0

Prove that number of commutative binary operations on a set having n elements is n^((n(n − 1))/2) .

$$\mathrm{Prove}\:\mathrm{that}\:\mathrm{number}\:\mathrm{of}\:\mathrm{commutative} \\ $$$$\mathrm{binary}\:\mathrm{operations}\:\mathrm{on}\:\mathrm{a}\:\mathrm{set}\:\mathrm{having}\:{n} \\ $$$$\mathrm{elements}\:\mathrm{is}\:{n}^{\frac{{n}\left({n}\:−\:\mathrm{1}\right)}{\mathrm{2}}} \:. \\ $$

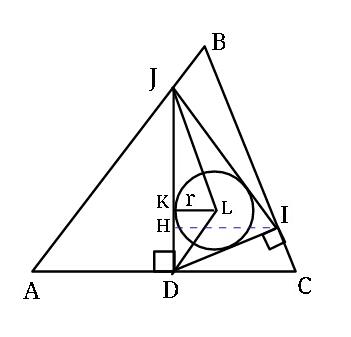
Question Number 15301 Answers: 1 Comments: 0

With the help of graph, find the solution set of inequation tan x > −(√3) .

$$\mathrm{With}\:\mathrm{the}\:\mathrm{help}\:\mathrm{of}\:\mathrm{graph},\:\mathrm{find}\:\mathrm{the} \\ $$$$\mathrm{solution}\:\mathrm{set}\:\mathrm{of}\:\mathrm{inequation}\:\mathrm{tan}\:{x}\:>\:−\sqrt{\mathrm{3}}\:. \\ $$

Question Number 15300 Answers: 1 Comments: 3

Find the values of α and β, 0 < α, β < (π/2) satisfying the following equation cos α cos β cos (α + β) = −(1/8) .

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{values}\:\mathrm{of}\:\alpha\:\mathrm{and}\:\beta,\:\mathrm{0}\:<\:\alpha,\:\beta\:<\:\frac{\pi}{\mathrm{2}} \\ $$$$\mathrm{satisfying}\:\mathrm{the}\:\mathrm{following}\:\mathrm{equation} \\ $$$$\mathrm{cos}\:\alpha\:\mathrm{cos}\:\beta\:\mathrm{cos}\:\left(\alpha\:+\:\beta\right)\:=\:−\frac{\mathrm{1}}{\mathrm{8}}\:. \\ $$

Question Number 15298 Answers: 1 Comments: 4

Solve for x and y x^2 + 2x sin(xy) + 1 = 0

$$\mathrm{Solve}\:\mathrm{for}\:{x}\:\mathrm{and}\:{y} \\ $$$${x}^{\mathrm{2}} \:+\:\mathrm{2}{x}\:\mathrm{sin}\left({xy}\right)\:+\:\mathrm{1}\:=\:\mathrm{0} \\ $$

Question Number 15294 Answers: 0 Comments: 1

A straigth conductor of length L is charged with q charge. What will be the electric field in the equatorial line at a distance d ? Ans: ((2q)/(4Πε_0 d(√(L^2 +4d^2 ))))

$$\mathrm{A}\:\mathrm{straigth}\:\mathrm{conductor}\:\mathrm{of}\:\mathrm{length} \\ $$$$\mathrm{L}\:\mathrm{is}\:\mathrm{charged}\:\mathrm{with}\:\mathrm{q}\:\mathrm{charge}.\:\mathrm{What} \\ $$$$\mathrm{will}\:\mathrm{be}\:\mathrm{the}\:\mathrm{electric}\:\mathrm{field}\:\mathrm{in}\:\mathrm{the} \\ $$$$\mathrm{equatorial}\:\mathrm{line}\:\mathrm{at}\:\mathrm{a}\:\mathrm{distance}\:\mathrm{d}\:? \\ $$$$\mathrm{Ans}:\:\frac{\mathrm{2q}}{\mathrm{4}\Pi\epsilon_{\mathrm{0}} \mathrm{d}\sqrt{\mathrm{L}^{\mathrm{2}} +\mathrm{4d}^{\mathrm{2}} }} \\ $$

Question Number 15288 Answers: 0 Comments: 1

Question Number 15284 Answers: 1 Comments: 3

Question Number 15266 Answers: 0 Comments: 0

calculate the pH of 1(N) HCl.

$$\mathrm{calculate}\:\mathrm{the}\:\mathrm{pH}\:\mathrm{of}\:\mathrm{1}\left(\mathrm{N}\right)\:\mathrm{HCl}. \\ $$

Question Number 15264 Answers: 1 Comments: 0

The solution set of inequation cos x + (1/2) ≥ 0 is [−π, π] (1) [0, ((2π)/3)] (2) [−((2π)/3), ((2π)/3)] (3) [0, (π/2)] (4) [−(π/2), ((3π)/2)]

$$\mathrm{The}\:\mathrm{solution}\:\mathrm{set}\:\mathrm{of}\:\mathrm{inequation} \\ $$$$\mathrm{cos}\:{x}\:+\:\frac{\mathrm{1}}{\mathrm{2}}\:\geqslant\:\mathrm{0}\:\mathrm{is}\:\left[−\pi,\:\pi\right] \\ $$$$\left(\mathrm{1}\right)\:\left[\mathrm{0},\:\frac{\mathrm{2}\pi}{\mathrm{3}}\right] \\ $$$$\left(\mathrm{2}\right)\:\left[−\frac{\mathrm{2}\pi}{\mathrm{3}},\:\frac{\mathrm{2}\pi}{\mathrm{3}}\right] \\ $$$$\left(\mathrm{3}\right)\:\left[\mathrm{0},\:\frac{\pi}{\mathrm{2}}\right] \\ $$$$\left(\mathrm{4}\right)\:\left[−\frac{\pi}{\mathrm{2}},\:\frac{\mathrm{3}\pi}{\mathrm{2}}\right] \\ $$

Question Number 15263 Answers: 1 Comments: 0

The equation asinx + cos2x = 2a − 7 possesses a solution if (1) a > 6 (2) 2 ≤ a ≤ 6 (3) a > 2 (4) a

$$\mathrm{The}\:\mathrm{equation}\:{a}\mathrm{sin}{x}\:+\:\mathrm{cos2}{x}\:=\:\mathrm{2}{a}\:−\:\mathrm{7} \\ $$$$\mathrm{possesses}\:\mathrm{a}\:\mathrm{solution}\:\mathrm{if} \\ $$$$\left(\mathrm{1}\right)\:{a}\:>\:\mathrm{6} \\ $$$$\left(\mathrm{2}\right)\:\mathrm{2}\:\leqslant\:{a}\:\leqslant\:\mathrm{6} \\ $$$$\left(\mathrm{3}\right)\:{a}\:>\:\mathrm{2} \\ $$$$\left(\mathrm{4}\right)\:{a} \\ $$

Question Number 15262 Answers: 0 Comments: 7

Solve : x^2 − 6x + [x] + 7 = 0.

$$\mathrm{Solve}\::\:{x}^{\mathrm{2}} \:−\:\mathrm{6}{x}\:+\:\left[{x}\right]\:+\:\mathrm{7}\:=\:\mathrm{0}. \\ $$

Question Number 15267 Answers: 2 Comments: 6

In an acute angled ΔABC, the minimum value of tan A tan B tan C is?

$$\mathrm{In}\:\mathrm{an}\:\mathrm{acute}\:\mathrm{angled}\:\Delta{ABC},\:\mathrm{the} \\ $$$$\mathrm{minimum}\:\mathrm{value}\:\mathrm{of}\:\mathrm{tan}\:{A}\:\mathrm{tan}\:{B}\:\mathrm{tan}\:{C}\:\mathrm{is}? \\ $$

Question Number 15292 Answers: 3 Comments: 0

Light version of Q#13724 Expansion of 100! has 24, 0′s at the end. Find the first non-zero digit from right. 100!=.....d000...00 What is the value of d?

$$\mathrm{Light}\:\mathrm{version}\:\mathrm{of}\:\mathcal{Q}#\mathrm{13724} \\ $$$$\mathcal{E}\mathrm{xpansion}\:\mathrm{of}\:\mathrm{100}!\:\mathrm{has}\:\mathrm{24},\:\mathrm{0}'\mathrm{s}\:\:\mathrm{at}\:\mathrm{the}\:\mathrm{end}. \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{first}\:\mathrm{non}-\mathrm{zero}\:\mathrm{digit}\:\mathrm{from}\:\mathrm{right}. \\ $$$$\mathrm{100}!=.....\mathrm{d000}...\mathrm{00} \\ $$$$\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\mathrm{d}? \\ $$

Question Number 15234 Answers: 0 Comments: 2

A question related to Q.15184 Find the maximum of f(x)=(ln x)^(1/x)

$$\mathrm{A}\:\mathrm{question}\:\mathrm{related}\:\mathrm{to}\:\mathrm{Q}.\mathrm{15184} \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{maximum}\:\mathrm{of}\:\mathrm{f}\left(\mathrm{x}\right)=\left(\mathrm{ln}\:\mathrm{x}\right)^{\frac{\mathrm{1}}{\mathrm{x}}} \\ $$

Question Number 15233 Answers: 1 Comments: 0

Find the general solution of the following homogeneous system of equation x_1 + x_2 − 2x_3 = 0 3x_1 − x_2 − 6x_3 = 0 −2x_1 + 3x_(2 ) + 4x_3 = 0

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{general}\:\mathrm{solution}\:\mathrm{of}\:\mathrm{the}\:\mathrm{following}\:\mathrm{homogeneous}\:\mathrm{system}\:\mathrm{of} \\ $$$$\mathrm{equation}\:\: \\ $$$$\mathrm{x}_{\mathrm{1}} \:+\:\mathrm{x}_{\mathrm{2}} \:−\:\mathrm{2x}_{\mathrm{3}} \:=\:\mathrm{0} \\ $$$$\mathrm{3x}_{\mathrm{1}} \:−\:\mathrm{x}_{\mathrm{2}} \:−\:\mathrm{6x}_{\mathrm{3}} \:=\:\mathrm{0} \\ $$$$−\mathrm{2x}_{\mathrm{1}} \:+\:\mathrm{3x}_{\mathrm{2}\:} +\:\mathrm{4x}_{\mathrm{3}} \:=\:\mathrm{0} \\ $$

Pg 1922 Pg 1923 Pg 1924 Pg 1925 Pg 1926 Pg 1927 Pg 1928 Pg 1929 Pg 1930 Pg 1931

Contact: info@tinkutara.com