
AllQuestion and Answers: Page 1907
Question Number 17027 Answers: 0 Comments: 0

Question Number 17019 Answers: 1 Comments: 0
Question Number 17018 Answers: 0 Comments: 8
Question Number 17017 Answers: 1 Comments: 0
Question Number 17011 Answers: 1 Comments: 0
Question Number 17010 Answers: 1 Comments: 0
Question Number 17009 Answers: 0 Comments: 1
Question Number 17008 Answers: 1 Comments: 0
Question Number 17053 Answers: 1 Comments: 0
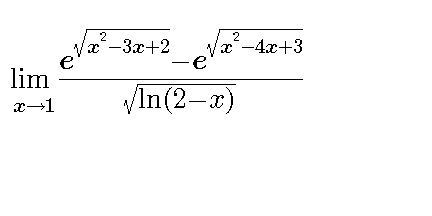
Question Number 19167 Answers: 1 Comments: 1
Question Number 17169 Answers: 2 Comments: 0
Question Number 16983 Answers: 1 Comments: 0
Question Number 17096 Answers: 1 Comments: 0
Question Number 16980 Answers: 0 Comments: 1
Question Number 16973 Answers: 1 Comments: 0
Question Number 16990 Answers: 1 Comments: 4
Question Number 16958 Answers: 0 Comments: 0
Question Number 16951 Answers: 0 Comments: 0
Question Number 16947 Answers: 0 Comments: 0
Question Number 16946 Answers: 0 Comments: 0
Question Number 16944 Answers: 0 Comments: 2
Question Number 16969 Answers: 0 Comments: 0

Question Number 16942 Answers: 1 Comments: 0
Question Number 16940 Answers: 1 Comments: 1
Question Number 16938 Answers: 1 Comments: 2
Question Number 16936 Answers: 0 Comments: 5
Pg 1902 Pg 1903 Pg 1904 Pg 1905 Pg 1906 Pg 1907 Pg 1908 Pg 1909 Pg 1910 Pg 1911
