Question and Answers Forum

AllQuestion and Answers: Page 1900

Question Number 18411 Answers: 1 Comments: 0

A glass bulb contains 2.24 L of H_2 and 1.12 L of D_2 at S.T.P. It is connected to a fully evacuated bulb by a stopcock with a small opening. The stopcock is opened for sometime and then closed. The first bulb now contains 0.1 g of D_2 . Calculate the percentage composition by weight of the gases in the second bulb.

$$\mathrm{A}\:\mathrm{glass}\:\mathrm{bulb}\:\mathrm{contains}\:\mathrm{2}.\mathrm{24}\:\mathrm{L}\:\mathrm{of}\:\mathrm{H}_{\mathrm{2}} \:\mathrm{and} \\ $$$$\mathrm{1}.\mathrm{12}\:\mathrm{L}\:\mathrm{of}\:\mathrm{D}_{\mathrm{2}} \:\mathrm{at}\:\mathrm{S}.\mathrm{T}.\mathrm{P}.\:\mathrm{It}\:\mathrm{is}\:\mathrm{connected}\:\mathrm{to} \\ $$$$\mathrm{a}\:\mathrm{fully}\:\mathrm{evacuated}\:\mathrm{bulb}\:\mathrm{by}\:\mathrm{a}\:\mathrm{stopcock} \\ $$$$\mathrm{with}\:\mathrm{a}\:\mathrm{small}\:\mathrm{opening}.\:\mathrm{The}\:\mathrm{stopcock}\:\mathrm{is} \\ $$$$\mathrm{opened}\:\mathrm{for}\:\mathrm{sometime}\:\mathrm{and}\:\mathrm{then}\:\mathrm{closed}. \\ $$$$\mathrm{The}\:\mathrm{first}\:\mathrm{bulb}\:\mathrm{now}\:\mathrm{contains}\:\mathrm{0}.\mathrm{1}\:\mathrm{g}\:\mathrm{of}\:\mathrm{D}_{\mathrm{2}} . \\ $$$$\mathrm{Calculate}\:\mathrm{the}\:\mathrm{percentage}\:\mathrm{composition} \\ $$$$\mathrm{by}\:\mathrm{weight}\:\mathrm{of}\:\mathrm{the}\:\mathrm{gases}\:\mathrm{in}\:\mathrm{the}\:\mathrm{second} \\ $$$$\mathrm{bulb}. \\ $$

Question Number 20955 Answers: 1 Comments: 0

lemme join miss Tawa Tawa here. It takes 8 painters working at the same rate ,5 hours to paint a house.If 6 painters are working at 2/3 the rate of the 8 painters,how long would it take them to paint the same house?

$$\mathrm{lemme}\:\mathrm{join}\:\mathrm{miss}\:\mathrm{Tawa}\:\mathrm{Tawa}\:\mathrm{here}. \\ $$$$ \\ $$$$\mathrm{It}\:\mathrm{takes}\:\mathrm{8}\:\mathrm{painters}\:\mathrm{working}\:\mathrm{at}\:\mathrm{the} \\ $$$$\mathrm{same}\:\mathrm{rate}\:,\mathrm{5}\:\mathrm{hours}\:\mathrm{to}\:\mathrm{paint}\:\mathrm{a} \\ $$$$\mathrm{house}.\mathrm{If}\:\mathrm{6}\:\mathrm{painters}\:\mathrm{are}\:\mathrm{working}\:\mathrm{at} \\ $$$$\mathrm{2}/\mathrm{3}\:\mathrm{the}\:\mathrm{rate}\:\mathrm{of}\:\mathrm{the}\:\mathrm{8}\:\mathrm{painters},\mathrm{how} \\ $$$$\mathrm{long}\:\mathrm{would}\:\mathrm{it}\:\mathrm{take}\:\mathrm{them}\:\mathrm{to}\:\mathrm{paint} \\ $$$$\mathrm{the}\:\mathrm{same}\:\mathrm{house}? \\ $$

Question Number 18429 Answers: 0 Comments: 6

30cm^3 of hydrogen at s.t.p combines with 20cm^3 of oxygen to form steam according to the following equation, 2H_2 (g) + O_2 (g) → 2H_2 O (g). Calculate the total volume of gaseous mixture at the end of the reaction.

$$\mathrm{30cm}^{\mathrm{3}} \:\mathrm{of}\:\mathrm{hydrogen}\:\mathrm{at}\:\mathrm{s}.\mathrm{t}.\mathrm{p}\:\mathrm{combines}\:\mathrm{with}\:\mathrm{20cm}^{\mathrm{3}} \:\mathrm{of}\:\mathrm{oxygen}\:\mathrm{to}\:\mathrm{form}\:\mathrm{steam}\: \\ $$$$\mathrm{according}\:\mathrm{to}\:\mathrm{the}\:\mathrm{following}\:\mathrm{equation},\:\:\mathrm{2H}_{\mathrm{2}} \:\left(\mathrm{g}\right)\:+\:\mathrm{O}_{\mathrm{2}} \:\left(\mathrm{g}\right)\:\rightarrow\:\mathrm{2H}_{\mathrm{2}} \mathrm{O}\:\left(\mathrm{g}\right). \\ $$$$\mathrm{Calculate}\:\mathrm{the}\:\mathrm{total}\:\mathrm{volume}\:\mathrm{of}\:\mathrm{gaseous}\:\mathrm{mixture}\:\mathrm{at}\:\mathrm{the}\:\mathrm{end}\:\mathrm{of}\:\mathrm{the}\:\mathrm{reaction}. \\ $$

Question Number 18402 Answers: 1 Comments: 0

If P_n = cos^n θ + sin^n θ, θ ∈ [0, (π/2)], n ∈ (−∞, 2), then minimum of P_n will be (1) 1 (2) (1/2) (3) (√2) (4) (1/(√2))

$$\mathrm{If}\:{P}_{{n}} \:=\:\mathrm{cos}^{{n}} \:\theta\:+\:\mathrm{sin}^{{n}} \:\theta,\:\theta\:\in\:\left[\mathrm{0},\:\frac{\pi}{\mathrm{2}}\right],\:{n}\:\in \\ $$$$\left(−\infty,\:\mathrm{2}\right),\:\mathrm{then}\:\mathrm{minimum}\:\mathrm{of}\:{P}_{{n}} \:\mathrm{will}\:\mathrm{be} \\ $$$$\left(\mathrm{1}\right)\:\mathrm{1} \\ $$$$\left(\mathrm{2}\right)\:\frac{\mathrm{1}}{\mathrm{2}} \\ $$$$\left(\mathrm{3}\right)\:\sqrt{\mathrm{2}} \\ $$$$\left(\mathrm{4}\right)\:\frac{\mathrm{1}}{\sqrt{\mathrm{2}}} \\ $$

Question Number 18400 Answers: 0 Comments: 1

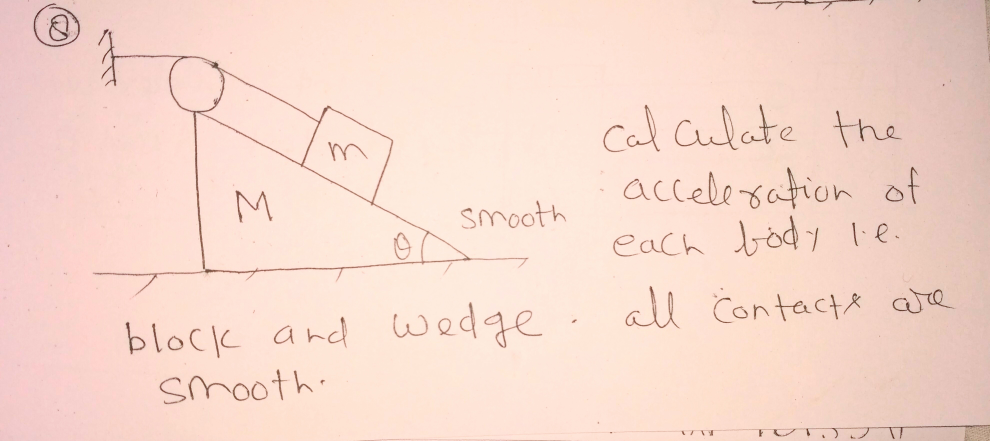
Question Number 18397 Answers: 0 Comments: 0

Prove : ∀n ∈ N, n ≥ 2, so ∃ x,y,z ∣ x,y,z ∈ N such that (4/n) = (1/x) + (1/y) + (1/z) Example: choose n = 2 (4/2) = (1/x) + (1/y) + (1/z) If x = 1 , y = 2 and z = 2, the equa- tion is correct!

$${Prove}\::\:\forall{n}\:\in\:\mathbb{N},\:{n}\:\geqslant\:\mathrm{2},\:{so}\:\exists\:{x},{y},{z}\:\mid\: \\ $$$${x},{y},{z}\:\in\:\mathbb{N}\:{such}\:{that}\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\mathrm{4}}{{n}}\:=\:\frac{\mathrm{1}}{{x}}\:+\:\frac{\mathrm{1}}{{y}}\:+\:\frac{\mathrm{1}}{{z}} \\ $$$${Example}: \\ $$$${choose}\:{n}\:=\:\mathrm{2} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\frac{\mathrm{4}}{\mathrm{2}}\:=\:\frac{\mathrm{1}}{{x}}\:+\:\frac{\mathrm{1}}{{y}}\:+\:\frac{\mathrm{1}}{{z}} \\ $$$${If}\:{x}\:=\:\mathrm{1}\:\:,\:\:\:{y}\:=\:\mathrm{2}\:\:{and}\:\:\:{z}\:=\:\mathrm{2},\:{the}\:{equa}- \\ $$$${tion}\:{is}\:{correct}! \\ $$

Question Number 18396 Answers: 1 Comments: 0

∫ (√(1 + (1/x^2 ) + (1/((x + 1)^2 )))) dx

$$\int\:\sqrt{\mathrm{1}\:+\:\frac{\mathrm{1}}{{x}^{\mathrm{2}} }\:+\:\frac{\mathrm{1}}{\left({x}\:+\:\mathrm{1}\right)^{\mathrm{2}} }}\:\:{dx} \\ $$

Question Number 18394 Answers: 0 Comments: 0

Question Number 18392 Answers: 1 Comments: 1

Question Number 18388 Answers: 1 Comments: 0

Solve simultaneously. x + y = 5 ....... (i) 5^x + y = 15 ...... (ii)

$$\mathrm{Solve}\:\mathrm{simultaneously}.\: \\ $$$$\mathrm{x}\:+\:\mathrm{y}\:=\:\mathrm{5}\:\:\:\:\:.......\:\left(\mathrm{i}\right) \\ $$$$\mathrm{5}^{\mathrm{x}} \:+\:\mathrm{y}\:=\:\mathrm{15}\:\:\:\:......\:\left(\mathrm{ii}\right) \\ $$

Question Number 18386 Answers: 1 Comments: 1

Prove that ((2 + (√5)))^(1/3) + ((2 − (√5)))^(1/3) is a rational number.

$$\mathrm{Prove}\:\mathrm{that}\:\sqrt[{\mathrm{3}}]{\mathrm{2}\:+\:\sqrt{\mathrm{5}}}\:+\:\sqrt[{\mathrm{3}}]{\mathrm{2}\:−\:\sqrt{\mathrm{5}}}\:\mathrm{is}\:\mathrm{a} \\ $$$$\mathrm{rational}\:\mathrm{number}. \\ $$

Question Number 18385 Answers: 1 Comments: 0

Find all the integers which are equal to 11 times the sum of their digits.

$$\mathrm{Find}\:\mathrm{all}\:\mathrm{the}\:\mathrm{integers}\:\mathrm{which}\:\mathrm{are}\:\mathrm{equal}\:\mathrm{to} \\ $$$$\mathrm{11}\:\mathrm{times}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{their}\:\mathrm{digits}. \\ $$

Question Number 18384 Answers: 1 Comments: 0

Let a, b, c ∈ R, a ≠ 0, such that a and 4a + 3b + 2c have the same sign. Show that the equation ax^2 + bx + c = 0 can not have both roots in the interval (1, 2).

$$\mathrm{Let}\:{a},\:{b},\:{c}\:\in\:{R},\:{a}\:\neq\:\mathrm{0},\:\mathrm{such}\:\mathrm{that}\:{a}\:\mathrm{and} \\ $$$$\mathrm{4}{a}\:+\:\mathrm{3}{b}\:+\:\mathrm{2}{c}\:\mathrm{have}\:\mathrm{the}\:\mathrm{same}\:\mathrm{sign}.\:\mathrm{Show} \\ $$$$\mathrm{that}\:\mathrm{the}\:\mathrm{equation}\:{ax}^{\mathrm{2}} \:+\:{bx}\:+\:{c}\:=\:\mathrm{0}\:\mathrm{can} \\ $$$$\mathrm{not}\:\mathrm{have}\:\mathrm{both}\:\mathrm{roots}\:\mathrm{in}\:\mathrm{the}\:\mathrm{interval} \\ $$$$\left(\mathrm{1},\:\mathrm{2}\right). \\ $$

Question Number 19200 Answers: 0 Comments: 5

A river of width d is flowing with speed u as shown in the figure. John can swim with maximum speed v relative to the river and can cross it in shortest time T. John starts at A. B is the point directly opposite to A on the other bank of the river. If t be the time John takes to reach the opposite bank, match the situation in the column I to the possibilities in column II. Column I (A) John reaches to the left of B (B) John reaches to the right of B (C) John reaches the point B (D) John drifts along the bank while minimizing the time Column II (p) t = T (q) t > T (r) u < v (s) u > v

Question Number 18379 Answers: 1 Comments: 0

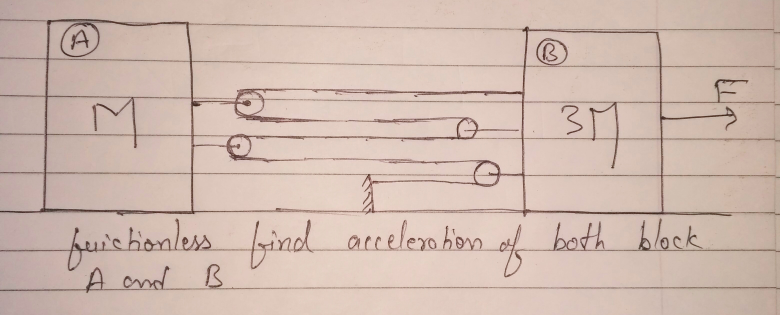
Question Number 18361 Answers: 0 Comments: 0

N propositions are judged by 2k−1 people. Each person assigns “true” to exactly M propositions and “false” to the other N−M (M ≤ N). To say a proposition is “approved” means it is true according to at least k judges. Find the minimum and maximum numbers of approved propositions given N, M and k.

$${N}\:\mathrm{propositions}\:\mathrm{are}\:\mathrm{judged}\:\mathrm{by}\:\mathrm{2}{k}−\mathrm{1}\:\mathrm{people}. \\ $$$$\mathrm{Each}\:\mathrm{person}\:\mathrm{assigns}\:``\mathrm{true}''\:\mathrm{to} \\ $$$$\mathrm{exactly}\:{M}\:\mathrm{propositions}\:\mathrm{and}\:``\mathrm{false}'' \\ $$$$\mathrm{to}\:\mathrm{the}\:\mathrm{other}\:{N}−{M}\:\left({M}\:\leqslant\:{N}\right). \\ $$$$\mathrm{To}\:\mathrm{say}\:\mathrm{a}\:\mathrm{proposition}\:\mathrm{is}\:``\mathrm{approved}''\:\mathrm{means} \\ $$$$\mathrm{it}\:\mathrm{is}\:\mathrm{true}\:\mathrm{according}\:\mathrm{to}\:\mathrm{at}\:\mathrm{least}\:{k}\:\mathrm{judges}. \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{minimum}\:\mathrm{and}\:\mathrm{maximum}\:\mathrm{numbers} \\ $$$$\mathrm{of}\:\mathrm{approved}\:\mathrm{propositions}\:\mathrm{given}\:{N},\:{M}\:\mathrm{and}\:{k}. \\ $$

Question Number 18377 Answers: 0 Comments: 0

Suppose one is given two vector field A and B in region of space such that, A(x,y,z) = 4xi + zj + y^2 z^2 k B(x,y,z) = yi +3j − yzk Find: C(x,y,z) if C = A ∧ B Also prove that, C(x,y,z) is perpendicular to A(x,y,z)

$$\mathrm{Suppose}\:\mathrm{one}\:\mathrm{is}\:\mathrm{given}\:\mathrm{two}\:\mathrm{vector}\:\mathrm{field}\:\mathrm{A}\:\mathrm{and}\:\mathrm{B}\:\mathrm{in}\:\mathrm{region}\:\mathrm{of}\:\mathrm{space}\:\mathrm{such}\:\mathrm{that}, \\ $$$$\mathrm{A}\left(\mathrm{x},\mathrm{y},\mathrm{z}\right)\:=\:\mathrm{4xi}\:+\:\mathrm{zj}\:+\:\mathrm{y}^{\mathrm{2}} \mathrm{z}^{\mathrm{2}} \mathrm{k} \\ $$$$\mathrm{B}\left(\mathrm{x},\mathrm{y},\mathrm{z}\right)\:=\:\mathrm{yi}\:+\mathrm{3j}\:−\:\mathrm{yzk} \\ $$$$\mathrm{Find}:\:\:\:\mathrm{C}\left(\mathrm{x},\mathrm{y},\mathrm{z}\right)\:\mathrm{if}\:\mathrm{C}\:=\:\mathrm{A}\:\wedge\:\mathrm{B} \\ $$$$\mathrm{Also}\:\mathrm{prove}\:\mathrm{that},\:\:\mathrm{C}\left(\mathrm{x},\mathrm{y},\mathrm{z}\right)\:\mathrm{is}\:\mathrm{perpendicular}\:\mathrm{to}\:\mathrm{A}\left(\mathrm{x},\mathrm{y},\mathrm{z}\right) \\ $$

Question Number 18721 Answers: 1 Comments: 0

Let ABC and ABC′ be two non- congruent triangles with sides AB = 4, AC = AC′ = 2(√2) and angle B = 30°. The absolute value of the difference between the areas of these triangles is

$$\mathrm{Let}\:{ABC}\:\mathrm{and}\:{ABC}'\:\mathrm{be}\:\mathrm{two}\:\mathrm{non}- \\ $$$$\mathrm{congruent}\:\mathrm{triangles}\:\mathrm{with}\:\mathrm{sides}\:{AB}\:=\:\mathrm{4}, \\ $$$${AC}\:=\:{AC}'\:=\:\mathrm{2}\sqrt{\mathrm{2}}\:\mathrm{and}\:\mathrm{angle}\:{B}\:=\:\mathrm{30}°. \\ $$$$\mathrm{The}\:\mathrm{absolute}\:\mathrm{value}\:\mathrm{of}\:\mathrm{the}\:\mathrm{difference} \\ $$$$\mathrm{between}\:\mathrm{the}\:\mathrm{areas}\:\mathrm{of}\:\mathrm{these}\:\mathrm{triangles}\:\mathrm{is} \\ $$

Question Number 18357 Answers: 0 Comments: 0

From the topic transformer prove that: e = (√2) ε cos(ωt)

$$\mathrm{From}\:\mathrm{the}\:\mathrm{topic}\:\mathrm{transformer} \\ $$$$ \\ $$$$\mathrm{prove}\:\mathrm{that}:\:\:\mathrm{e}\:=\:\sqrt{\mathrm{2}}\:\varepsilon\:\mathrm{cos}\left(\omega\mathrm{t}\right) \\ $$

Question Number 18355 Answers: 0 Comments: 0

Question Number 18349 Answers: 0 Comments: 0

Consider the iteration x_(k+1) =x_k −(([f(x)]^2 )/(f(x_k +f(x_k ))−f(x_k ))), k=0,1,2,... for the solution of f(x)=0. Explain the connection with Newton′s method, and show that (x_k ) converges quadratically if x_0 is sufficiently close to the solution.

$${Consider}\:{the}\:{iteration} \\ $$$${x}_{{k}+\mathrm{1}} ={x}_{{k}} −\frac{\left[{f}\left({x}\right)\right]^{\mathrm{2}} }{{f}\left({x}_{{k}} +{f}\left({x}_{{k}} \right)\right)−{f}\left({x}_{{k}} \right)},\:\:\:\:\:{k}=\mathrm{0},\mathrm{1},\mathrm{2},... \\ $$$${for}\:{the}\:{solution}\:{of}\:{f}\left({x}\right)=\mathrm{0}.\:{Explain}\:{the} \\ $$$${connection}\:{with}\:{Newton}'{s}\:{method},\:{and}\:{show} \\ $$$${that}\:\left({x}_{{k}} \right)\:{converges}\:{quadratically}\:{if}\:{x}_{\mathrm{0}} \:{is} \\ $$$${sufficiently}\:{close}\:{to}\:{the}\:{solution}. \\ $$$$ \\ $$

Question Number 18342 Answers: 2 Comments: 0

Question Number 19199 Answers: 1 Comments: 0

y=tan x^(tan x^(tan x) )

$$\mathrm{y}=\mathrm{tan}\:\mathrm{x}^{\mathrm{tan}\:\mathrm{x}^{\mathrm{tan}\:\mathrm{x}} } \\ $$

Question Number 18369 Answers: 1 Comments: 0

Prove that a^4 + b^4 + c^4 ≥ abc(a + b + c)

$$\mathrm{Prove}\:\mathrm{that}\:{a}^{\mathrm{4}} \:+\:{b}^{\mathrm{4}} \:+\:{c}^{\mathrm{4}} \:\geqslant\:{abc}\left({a}\:+\:{b}\:+\:{c}\right) \\ $$

Question Number 18366 Answers: 1 Comments: 0

Question Number 18365 Answers: 1 Comments: 0

Pg 1895 Pg 1896 Pg 1897 Pg 1898 Pg 1899 Pg 1900 Pg 1901 Pg 1902 Pg 1903 Pg 1904

Contact: info@tinkutara.com