
AllQuestion and Answers: Page 1898
Question Number 18556 Answers: 1 Comments: 0

Question Number 18551 Answers: 1 Comments: 0
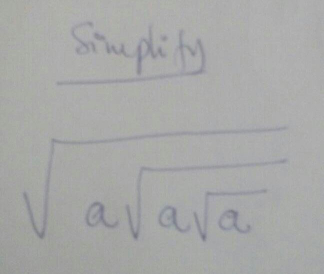
Question Number 18550 Answers: 1 Comments: 1

Question Number 18549 Answers: 0 Comments: 0
Question Number 18546 Answers: 0 Comments: 2
Question Number 18545 Answers: 1 Comments: 0
Question Number 18543 Answers: 1 Comments: 0

Question Number 18538 Answers: 0 Comments: 0

Question Number 18530 Answers: 1 Comments: 0
Question Number 19238 Answers: 0 Comments: 4
Question Number 19236 Answers: 1 Comments: 0

Question Number 18527 Answers: 0 Comments: 0
Question Number 18524 Answers: 2 Comments: 0
Question Number 18523 Answers: 0 Comments: 0
Question Number 18502 Answers: 1 Comments: 0
Question Number 18498 Answers: 5 Comments: 1
Question Number 18499 Answers: 0 Comments: 1
Question Number 18493 Answers: 1 Comments: 1
Question Number 18492 Answers: 1 Comments: 1

Question Number 18486 Answers: 0 Comments: 0
Question Number 18477 Answers: 0 Comments: 0
Question Number 18474 Answers: 1 Comments: 0
Question Number 18472 Answers: 1 Comments: 0
Question Number 18466 Answers: 1 Comments: 0
Question Number 18465 Answers: 1 Comments: 0
Question Number 18464 Answers: 1 Comments: 0
Pg 1893 Pg 1894 Pg 1895 Pg 1896 Pg 1897 Pg 1898 Pg 1899 Pg 1900 Pg 1901 Pg 1902
