Question and Answers Forum

AllQuestion and Answers: Page 1897

Question Number 18642 Answers: 1 Comments: 0

What is the area of the region bounded by coordinate axis and the line tangent to the graph, y = (1/8)x^2 + (1/2)x + 1, at the point (0, 1)

$$\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{area}\:\mathrm{of}\:\mathrm{the}\:\mathrm{region}\:\mathrm{bounded}\:\mathrm{by}\:\mathrm{coordinate}\:\mathrm{axis}\:\mathrm{and}\:\mathrm{the}\:\mathrm{line} \\ $$$$\mathrm{tangent}\:\mathrm{to}\:\mathrm{the}\:\mathrm{graph},\:\:\mathrm{y}\:=\:\frac{\mathrm{1}}{\mathrm{8}}\mathrm{x}^{\mathrm{2}} \:+\:\frac{\mathrm{1}}{\mathrm{2}}\mathrm{x}\:+\:\mathrm{1},\:\mathrm{at}\:\mathrm{the}\:\mathrm{point}\:\:\left(\mathrm{0},\:\mathrm{1}\right) \\ $$

Question Number 18640 Answers: 1 Comments: 1

Question Number 18639 Answers: 0 Comments: 0

∫(√(1+x^4 )) dx please solve this question.

$$\int\sqrt{\mathrm{1}+{x}^{\mathrm{4}} }\:{dx} \\ $$$${please}\:{solve}\:{this}\:{question}. \\ $$

Question Number 18632 Answers: 0 Comments: 0

x − 5x + 3 = 7 −4x = 4 x = −1

$${x}\:−\:\mathrm{5}{x}\:+\:\mathrm{3}\:=\:\mathrm{7} \\ $$$$−\mathrm{4}{x}\:=\:\mathrm{4} \\ $$$${x}\:=\:−\mathrm{1} \\ $$

Question Number 18625 Answers: 1 Comments: 1

x−5×+3=7

$$\mathrm{x}−\mathrm{5}×+\mathrm{3}=\mathrm{7} \\ $$

Question Number 18624 Answers: 1 Comments: 0

Argon diffuses through a hole under prescribed condition of temperature and pressure at the rate of 3cm^3 per velocity. At what velocity will helium diffuse through the same hole under the same condition (Ar = 29.94 g, He = 4g).

$$\mathrm{Argon}\:\mathrm{diffuses}\:\mathrm{through}\:\mathrm{a}\:\mathrm{hole}\:\mathrm{under}\:\mathrm{prescribed}\:\mathrm{condition}\:\mathrm{of}\:\mathrm{temperature} \\ $$$$\mathrm{and}\:\mathrm{pressure}\:\mathrm{at}\:\mathrm{the}\:\mathrm{rate}\:\mathrm{of}\:\:\mathrm{3cm}^{\mathrm{3}} \:\mathrm{per}\:\mathrm{velocity}.\:\mathrm{At}\:\mathrm{what}\:\mathrm{velocity}\:\mathrm{will}\:\mathrm{helium}\: \\ $$$$\mathrm{diffuse}\:\mathrm{through}\:\mathrm{the}\:\mathrm{same}\:\mathrm{hole}\:\mathrm{under}\:\mathrm{the}\:\mathrm{same}\:\mathrm{condition}\: \\ $$$$\left(\mathrm{Ar}\:=\:\mathrm{29}.\mathrm{94}\:\mathrm{g},\:\:\mathrm{He}\:=\:\mathrm{4g}\right). \\ $$

Question Number 18627 Answers: 1 Comments: 1

lim_(x→0) (((sin x)/x))^((sin x)/(x−sin x))

$$\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left(\frac{\mathrm{sin}\:\mathrm{x}}{\mathrm{x}}\right)^{\frac{\mathrm{sin}\:\mathrm{x}}{\mathrm{x}−\mathrm{sin}\:\mathrm{x}}} \\ $$

Question Number 18614 Answers: 0 Comments: 1

Question Number 18607 Answers: 1 Comments: 1

A block of mass M is pulled vertically upward through a rope of mass m by applying force F on-one end of the rope. What force does the rope exert on the block?

$$\mathrm{A}\:\mathrm{block}\:\mathrm{of}\:\mathrm{mass}\:{M}\:\mathrm{is}\:\mathrm{pulled}\:\mathrm{vertically} \\ $$$$\mathrm{upward}\:\mathrm{through}\:\mathrm{a}\:\mathrm{rope}\:\mathrm{of}\:\mathrm{mass}\:{m}\:\mathrm{by} \\ $$$$\mathrm{applying}\:\mathrm{force}\:{F}\:\mathrm{on}-\mathrm{one}\:\mathrm{end}\:\mathrm{of}\:\mathrm{the}\:\mathrm{rope}. \\ $$$$\mathrm{What}\:\mathrm{force}\:\mathrm{does}\:\mathrm{the}\:\mathrm{rope}\:\mathrm{exert}\:\mathrm{on}\:\mathrm{the} \\ $$$$\mathrm{block}? \\ $$

Question Number 18606 Answers: 0 Comments: 0

(1/3)+(3/(3×7))+(5/(3×7×11))+(7/(3×7×11×15))+...n terms

$$\frac{\mathrm{1}}{\mathrm{3}}+\frac{\mathrm{3}}{\mathrm{3}×\mathrm{7}}+\frac{\mathrm{5}}{\mathrm{3}×\mathrm{7}×\mathrm{11}}+\frac{\mathrm{7}}{\mathrm{3}×\mathrm{7}×\mathrm{11}×\mathrm{15}}+...{n}\: \\ $$$$\:{terms} \\ $$

Question Number 18604 Answers: 0 Comments: 0

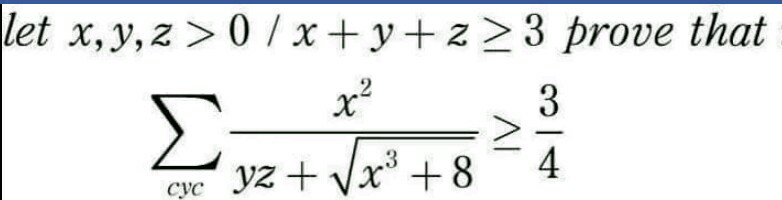
Question Number 18603 Answers: 0 Comments: 0

The work function of a metal is 4 eV. If light of frequency 2.3 × 10^(15) Hz is incident on metal surface, then, (1) No photoelectron will be ejected (2) 2 photoelectron of zero kinetic energy are ejected (3) 1 photoelectron of zero kinetic energy is ejected (4) 1 photoelectron is ejected, which required the stopping potential of 5.52 volt

$$\mathrm{The}\:\mathrm{work}\:\mathrm{function}\:\mathrm{of}\:\mathrm{a}\:\mathrm{metal}\:\mathrm{is}\:\mathrm{4}\:\mathrm{eV}.\:\mathrm{If} \\ $$$$\mathrm{light}\:\mathrm{of}\:\mathrm{frequency}\:\mathrm{2}.\mathrm{3}\:×\:\mathrm{10}^{\mathrm{15}} \:\mathrm{Hz}\:\mathrm{is} \\ $$$$\mathrm{incident}\:\mathrm{on}\:\mathrm{metal}\:\mathrm{surface},\:\mathrm{then}, \\ $$$$\left(\mathrm{1}\right)\:\mathrm{No}\:\mathrm{photoelectron}\:\mathrm{will}\:\mathrm{be}\:\mathrm{ejected} \\ $$$$\left(\mathrm{2}\right)\:\mathrm{2}\:\mathrm{photoelectron}\:\mathrm{of}\:\mathrm{zero}\:\mathrm{kinetic} \\ $$$$\mathrm{energy}\:\mathrm{are}\:\mathrm{ejected} \\ $$$$\left(\mathrm{3}\right)\:\mathrm{1}\:\mathrm{photoelectron}\:\mathrm{of}\:\mathrm{zero}\:\mathrm{kinetic} \\ $$$$\mathrm{energy}\:\mathrm{is}\:\mathrm{ejected} \\ $$$$\left(\mathrm{4}\right)\:\mathrm{1}\:\mathrm{photoelectron}\:\mathrm{is}\:\mathrm{ejected},\:\mathrm{which} \\ $$$$\mathrm{required}\:\mathrm{the}\:\mathrm{stopping}\:\mathrm{potential}\:\mathrm{of}\:\mathrm{5}.\mathrm{52} \\ $$$$\mathrm{volt} \\ $$

Question Number 18611 Answers: 1 Comments: 1

Without using L Hospital′s rule prove that lim_(x→0) ((sin x)/x)=1

$$\mathrm{Without}\:\mathrm{using}\:\mathrm{L}\:\mathrm{Hospital}'\mathrm{s} \\ $$$$\mathrm{rule}\:\mathrm{prove}\:\mathrm{that}\:\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{sin}\:\mathrm{x}}{\mathrm{x}}=\mathrm{1} \\ $$

Question Number 18593 Answers: 1 Comments: 0

Question Number 18590 Answers: 1 Comments: 0

∫ ((sin x)/(1 + cos^2 x)) dx

$$\int\:\frac{\mathrm{sin}\:{x}}{\mathrm{1}\:+\:\mathrm{cos}^{\mathrm{2}} \:{x}}\:{dx} \\ $$

Question Number 18588 Answers: 1 Comments: 0

sin x = ((2a + 3)/(a + 1)) How many a that can satisfy the equation above?

$$\mathrm{sin}\:{x}\:=\:\frac{\mathrm{2}{a}\:+\:\mathrm{3}}{{a}\:+\:\mathrm{1}} \\ $$$$\mathrm{How}\:\mathrm{many}\:{a}\:\mathrm{that}\:\mathrm{can}\:\mathrm{satisfy}\:\mathrm{the} \\ $$$$\mathrm{equation}\:\mathrm{above}? \\ $$

Question Number 18587 Answers: 0 Comments: 2

lim_(x→0) ((x . tan x)/(x sin x − cos x + 1))

$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{{x}\:.\:\mathrm{tan}\:{x}}{{x}\:\mathrm{sin}\:{x}\:−\:\mathrm{cos}\:{x}\:+\:\mathrm{1}} \\ $$

Question Number 18584 Answers: 1 Comments: 0

Prove sin (((3π)/(10)))=((1+(√5))/4)

$${Prove} \\ $$$$\mathrm{sin}\:\left(\frac{\mathrm{3}\pi}{\mathrm{10}}\right)=\frac{\mathrm{1}+\sqrt{\mathrm{5}}}{\mathrm{4}} \\ $$

Question Number 18575 Answers: 1 Comments: 0

If ( ) represents the least integer function, then the value of (10) + (10 + (1/(20))) + (10 + (2/(20))) + ... + (10 + ((19)/(20))) is equal to (1) 219 (2) 200 (3) 220 (4) 221

$$\mathrm{If}\:\left(\:\right)\:\mathrm{represents}\:\mathrm{the}\:\mathrm{least}\:\mathrm{integer}\:\mathrm{function}, \\ $$$$\mathrm{then}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\left(\mathrm{10}\right)\:+\:\left(\mathrm{10}\:+\:\frac{\mathrm{1}}{\mathrm{20}}\right)\:+ \\ $$$$\left(\mathrm{10}\:+\:\frac{\mathrm{2}}{\mathrm{20}}\right)\:+\:...\:+\:\left(\mathrm{10}\:+\:\frac{\mathrm{19}}{\mathrm{20}}\right)\:\mathrm{is}\:\mathrm{equal}\:\mathrm{to} \\ $$$$\left(\mathrm{1}\right)\:\mathrm{219} \\ $$$$\left(\mathrm{2}\right)\:\mathrm{200} \\ $$$$\left(\mathrm{3}\right)\:\mathrm{220} \\ $$$$\left(\mathrm{4}\right)\:\mathrm{221} \\ $$

Question Number 18574 Answers: 1 Comments: 0

The maximum value of f(x) = 2 − ∣x∣^2 − 2x is equal to (1) 6 (2) 4 (3) 5 (4) 3

$$\mathrm{The}\:\mathrm{maximum}\:\mathrm{value}\:\mathrm{of}\:{f}\left({x}\right)\:=\:\mathrm{2}\:−\:\mid{x}\mid^{\mathrm{2}} \\ $$$$−\:\mathrm{2}{x}\:\mathrm{is}\:\mathrm{equal}\:\mathrm{to} \\ $$$$\left(\mathrm{1}\right)\:\mathrm{6} \\ $$$$\left(\mathrm{2}\right)\:\mathrm{4} \\ $$$$\left(\mathrm{3}\right)\:\mathrm{5} \\ $$$$\left(\mathrm{4}\right)\:\mathrm{3} \\ $$

Question Number 18568 Answers: 0 Comments: 0

The energy required to dislodge electron from excited isolated H-atom, IE_1 = 13.6 eV is (1) = 13.6 eV (2) > 13.6 eV (3) < 13.6 eV and > 3.4 eV (4) ≤ 3.4 eV

$$\mathrm{The}\:\mathrm{energy}\:\mathrm{required}\:\mathrm{to}\:\mathrm{dislodge}\:\mathrm{electron} \\ $$$$\mathrm{from}\:\mathrm{excited}\:\mathrm{isolated}\:\mathrm{H}-\mathrm{atom},\:\mathrm{IE}_{\mathrm{1}} \:= \\ $$$$\mathrm{13}.\mathrm{6}\:\mathrm{eV}\:\mathrm{is} \\ $$$$\left(\mathrm{1}\right)\:=\:\mathrm{13}.\mathrm{6}\:\mathrm{eV} \\ $$$$\left(\mathrm{2}\right)\:>\:\mathrm{13}.\mathrm{6}\:\mathrm{eV} \\ $$$$\left(\mathrm{3}\right)\:<\:\mathrm{13}.\mathrm{6}\:\mathrm{eV}\:\mathrm{and}\:>\:\mathrm{3}.\mathrm{4}\:\mathrm{eV} \\ $$$$\left(\mathrm{4}\right)\:\leqslant\:\mathrm{3}.\mathrm{4}\:\mathrm{eV} \\ $$

Question Number 18581 Answers: 1 Comments: 0

In moving a body of mass m up and down a rough incline plane of inclination θ, work done is (S is length of the planck, and μ is coefficient of friction).

$$\mathrm{In}\:\mathrm{moving}\:\mathrm{a}\:\mathrm{body}\:\mathrm{of}\:\mathrm{mass}\:{m}\:\mathrm{up}\:\mathrm{and} \\ $$$$\mathrm{down}\:\mathrm{a}\:\mathrm{rough}\:\mathrm{incline}\:\mathrm{plane}\:\mathrm{of}\:\mathrm{inclination} \\ $$$$\theta,\:\mathrm{work}\:\mathrm{done}\:\mathrm{is}\:\left(\mathrm{S}\:\mathrm{is}\:\mathrm{length}\:\mathrm{of}\:\mathrm{the}\:\mathrm{planck},\right. \\ $$$$\left.\mathrm{and}\:\mu\:\mathrm{is}\:\mathrm{coefficient}\:\mathrm{of}\:\mathrm{friction}\right). \\ $$

Question Number 18561 Answers: 0 Comments: 2

The acceleration of an object is given by a(t) = cos(nπ), and its velocity at time t = 0 is (1/(2π)). Find both the net and the total distance traveled in the first 1.5 seconds.

$$\mathrm{The}\:\mathrm{acceleration}\:\mathrm{of}\:\mathrm{an}\:\mathrm{object}\:\mathrm{is}\:\mathrm{given}\:\mathrm{by}\:\mathrm{a}\left(\mathrm{t}\right)\:=\:\mathrm{cos}\left(\mathrm{n}\pi\right),\:\mathrm{and}\:\mathrm{its}\:\mathrm{velocity} \\ $$$$\mathrm{at}\:\mathrm{time}\:\mathrm{t}\:=\:\mathrm{0}\:\mathrm{is}\:\:\:\frac{\mathrm{1}}{\mathrm{2}\pi}.\:\mathrm{Find}\:\mathrm{both}\:\mathrm{the}\:\mathrm{net}\:\mathrm{and}\:\mathrm{the}\:\mathrm{total}\:\mathrm{distance}\:\mathrm{traveled}\:\mathrm{in}\:\mathrm{the} \\ $$$$\mathrm{first}\:\:\mathrm{1}.\mathrm{5}\:\mathrm{seconds}. \\ $$

Question Number 18556 Answers: 1 Comments: 0

Question Number 18551 Answers: 1 Comments: 0

Question Number 18550 Answers: 1 Comments: 1

Pg 1892 Pg 1893 Pg 1894 Pg 1895 Pg 1896 Pg 1897 Pg 1898 Pg 1899 Pg 1900 Pg 1901

Contact: info@tinkutara.com