
AllQuestion and Answers: Page 1895
Question Number 18823 Answers: 0 Comments: 2
Question Number 18818 Answers: 2 Comments: 0
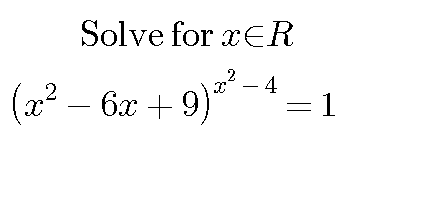
Question Number 18808 Answers: 1 Comments: 0
Question Number 18801 Answers: 0 Comments: 1

Question Number 18800 Answers: 0 Comments: 0
Question Number 18799 Answers: 1 Comments: 0

Question Number 18798 Answers: 1 Comments: 0
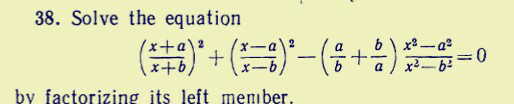
Question Number 18797 Answers: 1 Comments: 0
Question Number 18834 Answers: 1 Comments: 0

Question Number 18783 Answers: 1 Comments: 0
Question Number 18779 Answers: 0 Comments: 2
Question Number 18763 Answers: 0 Comments: 3

Question Number 18762 Answers: 1 Comments: 0
Question Number 18761 Answers: 0 Comments: 1
Question Number 18760 Answers: 0 Comments: 1
Question Number 18759 Answers: 2 Comments: 0
Question Number 18758 Answers: 1 Comments: 0
Question Number 18757 Answers: 0 Comments: 0
Question Number 18755 Answers: 1 Comments: 0
Question Number 18803 Answers: 1 Comments: 1

Question Number 18752 Answers: 0 Comments: 0
Question Number 18749 Answers: 1 Comments: 1
Question Number 18748 Answers: 1 Comments: 0

Question Number 18747 Answers: 2 Comments: 0

Question Number 18746 Answers: 2 Comments: 0

Question Number 18744 Answers: 0 Comments: 1

Pg 1890 Pg 1891 Pg 1892 Pg 1893 Pg 1894 Pg 1895 Pg 1896 Pg 1897 Pg 1898 Pg 1899
