
AllQuestion and Answers: Page 1894
Question Number 18907 Answers: 0 Comments: 3
Question Number 18906 Answers: 1 Comments: 0
Question Number 18905 Answers: 0 Comments: 0
Question Number 18904 Answers: 1 Comments: 0
Question Number 18893 Answers: 1 Comments: 0
Question Number 18892 Answers: 1 Comments: 0
Question Number 18891 Answers: 2 Comments: 0
Question Number 18889 Answers: 0 Comments: 2
$$\int\:\frac{{d}\left(\sqrt{\mathrm{3}{x}}\right)}{\sqrt{\sqrt{{x}}\:+\:\mathrm{7}}} \\ $$
Question Number 18872 Answers: 2 Comments: 0

Question Number 18871 Answers: 0 Comments: 1

Question Number 18866 Answers: 1 Comments: 0

Question Number 18864 Answers: 2 Comments: 1

Question Number 18859 Answers: 1 Comments: 0
Question Number 18854 Answers: 0 Comments: 0
Question Number 18847 Answers: 1 Comments: 0
Question Number 18846 Answers: 0 Comments: 0
Question Number 18885 Answers: 0 Comments: 0

Question Number 18886 Answers: 0 Comments: 0

Question Number 18841 Answers: 1 Comments: 0

Question Number 18830 Answers: 1 Comments: 2
Question Number 18827 Answers: 0 Comments: 3

Question Number 18851 Answers: 0 Comments: 0

Question Number 18823 Answers: 0 Comments: 2
Question Number 18818 Answers: 2 Comments: 0
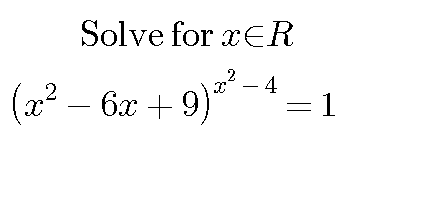
Question Number 18808 Answers: 1 Comments: 0
Question Number 18801 Answers: 0 Comments: 1

Pg 1889 Pg 1890 Pg 1891 Pg 1892 Pg 1893 Pg 1894 Pg 1895 Pg 1896 Pg 1897 Pg 1898
