
AllQuestion and Answers: Page 1869
Question Number 21707 Answers: 0 Comments: 3
Question Number 21702 Answers: 0 Comments: 1
Question Number 21701 Answers: 2 Comments: 1
Question Number 21686 Answers: 0 Comments: 0
Question Number 21685 Answers: 0 Comments: 0
Question Number 21683 Answers: 1 Comments: 2
Question Number 21682 Answers: 1 Comments: 0
Question Number 21670 Answers: 1 Comments: 1
Question Number 21662 Answers: 1 Comments: 0
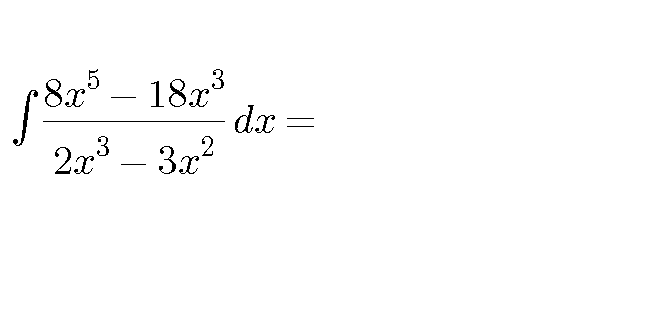
Question Number 21661 Answers: 0 Comments: 2

Question Number 21679 Answers: 1 Comments: 0
$$\int\frac{\mathrm{sec}\theta\:\mathrm{d}\theta}{\mathrm{1}−\mathrm{sec}\theta} \\ $$
Question Number 21656 Answers: 0 Comments: 4
Question Number 21655 Answers: 1 Comments: 0
Question Number 21654 Answers: 0 Comments: 0
Question Number 21653 Answers: 0 Comments: 0
Question Number 21651 Answers: 0 Comments: 4
Question Number 21650 Answers: 0 Comments: 0
Question Number 21680 Answers: 1 Comments: 0
Question Number 21634 Answers: 1 Comments: 0
Question Number 21708 Answers: 1 Comments: 0
$$\int_{\mathrm{0}} ^{\mathrm{0}.\mathrm{5}} \mathrm{2}{tan}^{\mathrm{2}} \mathrm{2}{tdt} \\ $$
Question Number 21628 Answers: 1 Comments: 0
Question Number 21626 Answers: 0 Comments: 1
Question Number 21622 Answers: 2 Comments: 0
Question Number 21643 Answers: 0 Comments: 1
Question Number 21612 Answers: 0 Comments: 0
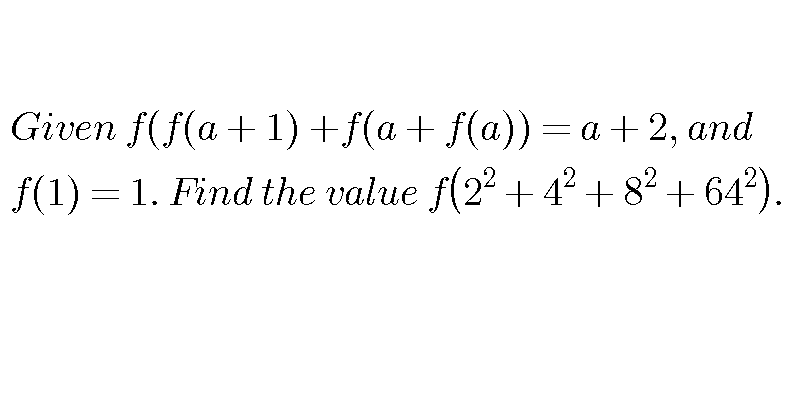
Question Number 21611 Answers: 1 Comments: 0
Pg 1864 Pg 1865 Pg 1866 Pg 1867 Pg 1868 Pg 1869 Pg 1870 Pg 1871 Pg 1872 Pg 1873
