
AllQuestion and Answers: Page 1854
Question Number 22941 Answers: 1 Comments: 3

Question Number 22893 Answers: 1 Comments: 0
Question Number 22892 Answers: 0 Comments: 3
Question Number 22883 Answers: 1 Comments: 1
Question Number 22881 Answers: 0 Comments: 3
Question Number 22879 Answers: 1 Comments: 0
Question Number 22872 Answers: 1 Comments: 4

Question Number 22856 Answers: 0 Comments: 1
$$\mathrm{solve}:\:\mathrm{sin}\left({x}\right)=\mathrm{2},\:\:\:{x}\in\mathbb{C} \\ $$
Question Number 22855 Answers: 1 Comments: 1
Question Number 22884 Answers: 1 Comments: 0
Question Number 22837 Answers: 0 Comments: 0
Question Number 22871 Answers: 1 Comments: 0
Question Number 22843 Answers: 1 Comments: 0
Question Number 22820 Answers: 0 Comments: 2
Question Number 22874 Answers: 4 Comments: 0

Question Number 22813 Answers: 1 Comments: 0
Question Number 22797 Answers: 1 Comments: 0
Question Number 22787 Answers: 0 Comments: 2
Question Number 22782 Answers: 0 Comments: 6
Question Number 22778 Answers: 0 Comments: 2

Question Number 22769 Answers: 1 Comments: 0
Question Number 22768 Answers: 1 Comments: 2
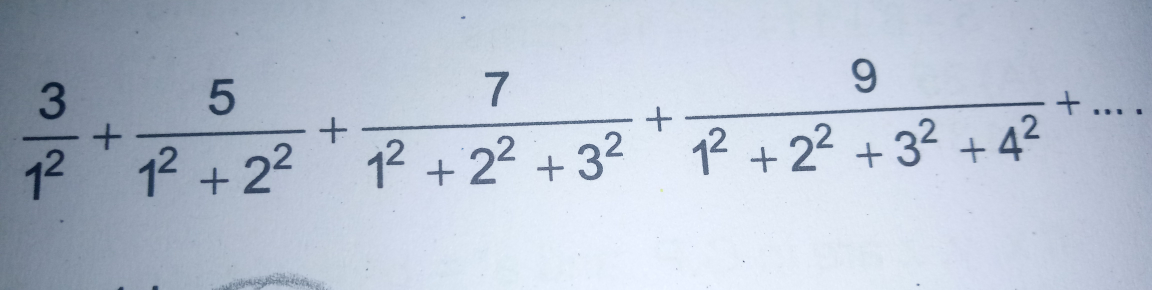
Question Number 22745 Answers: 1 Comments: 0
Question Number 22744 Answers: 0 Comments: 1
Question Number 22743 Answers: 1 Comments: 0
Question Number 22742 Answers: 2 Comments: 0

Pg 1849 Pg 1850 Pg 1851 Pg 1852 Pg 1853 Pg 1854 Pg 1855 Pg 1856 Pg 1857 Pg 1858
