Question and Answers Forum

AllQuestion and Answers: Page 1848

Question Number 24303 Answers: 1 Comments: 0

Assertion: Enthalpy of combustion is negative. Reason: Combustion reaction can be exothermic or endothermic.

$$\boldsymbol{\mathrm{Assertion}}:\:\mathrm{Enthalpy}\:\mathrm{of}\:\mathrm{combustion}\:\mathrm{is} \\ $$$$\mathrm{negative}. \\ $$$$\boldsymbol{\mathrm{Reason}}:\:\mathrm{Combustion}\:\mathrm{reaction}\:\mathrm{can}\:\mathrm{be} \\ $$$$\mathrm{exothermic}\:\mathrm{or}\:\mathrm{endothermic}. \\ $$

Question Number 24301 Answers: 0 Comments: 4

In the figure shown below, all surfaces are smooth, strings and pulley are ideal. If the wedge is moving with acceleration a towards the right, then the acceleration of the block with respect to the wedge at that instant is

$$\mathrm{In}\:\mathrm{the}\:\mathrm{figure}\:\mathrm{shown}\:\mathrm{below},\:\mathrm{all}\:\mathrm{surfaces} \\ $$$$\mathrm{are}\:\mathrm{smooth},\:\mathrm{strings}\:\mathrm{and}\:\mathrm{pulley}\:\mathrm{are}\:\mathrm{ideal}. \\ $$$$\mathrm{If}\:\mathrm{the}\:\mathrm{wedge}\:\mathrm{is}\:\mathrm{moving}\:\mathrm{with}\:\mathrm{acceleration} \\ $$$${a}\:\mathrm{towards}\:\mathrm{the}\:\mathrm{right},\:\mathrm{then}\:\mathrm{the}\:\mathrm{acceleration} \\ $$$$\mathrm{of}\:\mathrm{the}\:\mathrm{block}\:\mathrm{with}\:\mathrm{respect}\:\mathrm{to}\:\mathrm{the}\:\mathrm{wedge} \\ $$$$\mathrm{at}\:\mathrm{that}\:\mathrm{instant}\:\mathrm{is} \\ $$

Question Number 24294 Answers: 1 Comments: 0

Find value(s) of x if sin [2cos^(−1) {cot (2tan^(−1) x)}]=0 .

$${Find}\:{value}\left({s}\right)\:{of}\:\boldsymbol{{x}}\:{if} \\ $$$$\:\:\mathrm{sin}\:\left[\mathrm{2cos}^{−\mathrm{1}} \left\{\mathrm{cot}\:\left(\mathrm{2tan}^{−\mathrm{1}} {x}\right)\right\}\right]=\mathrm{0}\:. \\ $$

Question Number 24293 Answers: 1 Comments: 2

Find the centre of mass of a uniform (a) half-disc, (b) quarter-disc.

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{centre}\:\mathrm{of}\:\mathrm{mass}\:\mathrm{of}\:\mathrm{a}\:\mathrm{uniform} \\ $$$$\left({a}\right)\:\mathrm{half}-\mathrm{disc},\:\left({b}\right)\:\mathrm{quarter}-\mathrm{disc}. \\ $$

Question Number 24287 Answers: 0 Comments: 0

In a fuel cell, methanol is used as a fuel and O_2 is used as oxidizer. The standard enthalpy of combustion of methanol is −726 kJ mol^(−1) . The standard free energies of formation of CH_3 OH(l), CO_2 (g) and H_2 O(l) are −166.3, −394.4 and −237.1 kJ mol^(−1) respectively. The standard internal energy change of the cell reaction will be

$$\mathrm{In}\:\mathrm{a}\:\mathrm{fuel}\:\mathrm{cell},\:\mathrm{methanol}\:\mathrm{is}\:\mathrm{used}\:\mathrm{as}\:\mathrm{a}\:\mathrm{fuel} \\ $$$$\mathrm{and}\:\mathrm{O}_{\mathrm{2}} \:\mathrm{is}\:\mathrm{used}\:\mathrm{as}\:\mathrm{oxidizer}.\:\mathrm{The}\:\mathrm{standard} \\ $$$$\mathrm{enthalpy}\:\mathrm{of}\:\mathrm{combustion}\:\mathrm{of}\:\mathrm{methanol}\:\mathrm{is} \\ $$$$−\mathrm{726}\:\mathrm{kJ}\:\mathrm{mol}^{−\mathrm{1}} .\:\mathrm{The}\:\mathrm{standard}\:\mathrm{free} \\ $$$$\mathrm{energies}\:\mathrm{of}\:\mathrm{formation}\:\mathrm{of}\:\mathrm{CH}_{\mathrm{3}} \mathrm{OH}\left(\mathrm{l}\right), \\ $$$$\mathrm{CO}_{\mathrm{2}} \left(\mathrm{g}\right)\:\mathrm{and}\:\mathrm{H}_{\mathrm{2}} \mathrm{O}\left(\mathrm{l}\right)\:\mathrm{are}\:−\mathrm{166}.\mathrm{3},\:−\mathrm{394}.\mathrm{4} \\ $$$$\mathrm{and}\:−\mathrm{237}.\mathrm{1}\:\mathrm{kJ}\:\mathrm{mol}^{−\mathrm{1}} \:\mathrm{respectively}.\:\mathrm{The} \\ $$$$\mathrm{standard}\:\mathrm{internal}\:\mathrm{energy}\:\mathrm{change}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{cell}\:\mathrm{reaction}\:\mathrm{will}\:\mathrm{be} \\ $$

Question Number 24286 Answers: 1 Comments: 0

If ∣x∣ < 1 then (x + 1)(x^2 + 1)(x^4 + 1)(x^8 + 1)(x^(16) + 1)..... is equal to

$$\mathrm{If}\:\mid{x}\mid\:<\:\mathrm{1}\:\mathrm{then} \\ $$$$\left({x}\:+\:\mathrm{1}\right)\left({x}^{\mathrm{2}} \:+\:\mathrm{1}\right)\left({x}^{\mathrm{4}} \:+\:\mathrm{1}\right)\left({x}^{\mathrm{8}} \:+\:\mathrm{1}\right)\left({x}^{\mathrm{16}} \:+\:\mathrm{1}\right)..... \\ $$$$\mathrm{is}\:\mathrm{equal}\:\mathrm{to} \\ $$

Question Number 24263 Answers: 0 Comments: 5

The reversible expansion of an ideal gas under adiabatic and isothermal conditions is shown in the figure. Which of the following statement(s) is (are) correct? (1) T_1 = T_2 (2) T_3 > T_1 (3) w_(isothermal) > w_(adiabatic) (3) ΔU_(isothermal) > ΔU_(adiabatic)

$$\mathrm{The}\:\mathrm{reversible}\:\mathrm{expansion}\:\mathrm{of}\:\mathrm{an}\:\mathrm{ideal} \\ $$$$\mathrm{gas}\:\mathrm{under}\:\mathrm{adiabatic}\:\mathrm{and}\:\mathrm{isothermal} \\ $$$$\mathrm{conditions}\:\mathrm{is}\:\mathrm{shown}\:\mathrm{in}\:\mathrm{the}\:\mathrm{figure}.\:\mathrm{Which} \\ $$$$\mathrm{of}\:\mathrm{the}\:\mathrm{following}\:\mathrm{statement}\left(\mathrm{s}\right)\:\mathrm{is}\:\left(\mathrm{are}\right) \\ $$$$\mathrm{correct}? \\ $$$$\left(\mathrm{1}\right)\:\mathrm{T}_{\mathrm{1}} \:=\:\mathrm{T}_{\mathrm{2}} \\ $$$$\left(\mathrm{2}\right)\:\mathrm{T}_{\mathrm{3}} \:>\:\mathrm{T}_{\mathrm{1}} \\ $$$$\left(\mathrm{3}\right)\:\mathrm{w}_{\mathrm{isothermal}} \:>\:\mathrm{w}_{\mathrm{adiabatic}} \\ $$$$\left(\mathrm{3}\right)\:\Delta\mathrm{U}_{\mathrm{isothermal}} \:>\:\Delta\mathrm{U}_{\mathrm{adiabatic}} \\ $$

Question Number 24260 Answers: 0 Comments: 3

When a system of forces acts on a body moving in a circular path what happens to the resultant force when: 1)there′s no friction 2)there′s friction

$${When}\:{a}\:{system}\:{of}\:{forces}\:{acts}\:{on} \\ $$$${a}\:{body}\:{moving}\:{in}\:{a}\:{circular}\:{path} \\ $$$${what}\:{happens}\:{to}\:{the}\:{resultant} \\ $$$${force}\:{when}: \\ $$$$\left.\mathrm{1}\right){there}'{s}\:{no}\:{friction} \\ $$$$\left.\mathrm{2}\right){there}'{s}\:{friction} \\ $$$$ \\ $$$$ \\ $$

Question Number 24259 Answers: 0 Comments: 0

Which of the following reaction is/are exothermic reaction/s? (1) CaCO_3 → CaO + CO_2 (2) Fe + S → FeS (3) NaOH + HCl → NaCl + H_2 O (4) CH_4 + O_2 → CO_2 + 2H_2 O.

$$\mathrm{Which}\:\mathrm{of}\:\mathrm{the}\:\mathrm{following}\:\mathrm{reaction}\:\mathrm{is}/\mathrm{are} \\ $$$$\mathrm{exothermic}\:\mathrm{reaction}/\mathrm{s}? \\ $$$$\left(\mathrm{1}\right)\:\mathrm{CaCO}_{\mathrm{3}} \:\rightarrow\:\mathrm{CaO}\:+\:\mathrm{CO}_{\mathrm{2}} \\ $$$$\left(\mathrm{2}\right)\:\mathrm{Fe}\:+\:\mathrm{S}\:\rightarrow\:\mathrm{FeS} \\ $$$$\left(\mathrm{3}\right)\:\mathrm{NaOH}\:+\:\mathrm{HCl}\:\rightarrow\:\mathrm{NaCl}\:+\:\mathrm{H}_{\mathrm{2}} \mathrm{O} \\ $$$$\left(\mathrm{4}\right)\:\mathrm{CH}_{\mathrm{4}} \:+\:\mathrm{O}_{\mathrm{2}} \:\rightarrow\:\mathrm{CO}_{\mathrm{2}} \:+\:\mathrm{2H}_{\mathrm{2}} \mathrm{O}. \\ $$

Question Number 24324 Answers: 0 Comments: 2

Find the minimum possible least common multiple (lcm) of twenty (not necessarily distinct) natural numbers whose sum is 801.

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{minimum}\:\mathrm{possible}\:\mathrm{least} \\ $$$$\mathrm{common}\:\mathrm{multiple}\:\left(\mathrm{lcm}\right)\:\mathrm{of}\:\mathrm{twenty}\:\left(\mathrm{not}\right. \\ $$$$\left.\mathrm{necessarily}\:\mathrm{distinct}\right)\:\mathrm{natural}\:\mathrm{numbers} \\ $$$$\mathrm{whose}\:\mathrm{sum}\:\mathrm{is}\:\mathrm{801}. \\ $$

Question Number 24351 Answers: 0 Comments: 0

Question Number 24347 Answers: 0 Comments: 2

Question Number 24352 Answers: 0 Comments: 1

for what value of y will ((x^2 + 4)/(6x − 8)) lies between a positive integer.

$$\mathrm{for}\:\mathrm{what}\:\mathrm{value}\:\mathrm{of}\:\mathrm{y}\:\mathrm{will}\:\:\frac{\mathrm{x}^{\mathrm{2}} \:+\:\mathrm{4}}{\mathrm{6x}\:−\:\mathrm{8}}\:\:\mathrm{lies}\:\mathrm{between}\:\mathrm{a}\:\mathrm{positive}\:\mathrm{integer}. \\ $$

Question Number 24233 Answers: 2 Comments: 0

if (d^2 y/dx^2 )=ksiny then y=?

$${if}\:\frac{{d}^{\mathrm{2}} {y}}{{dx}^{\mathrm{2}} }={ksiny}\:{then}\:{y}=? \\ $$

Question Number 24227 Answers: 3 Comments: 1

Question Number 24186 Answers: 0 Comments: 4

(n − 1) equal point masses each of mass m are placed at the vertices of a regular n-polygon. The vacant vertex has a position vector a with respect to the centre of the polygon. Find the position vector of centre of mass.

$$\left({n}\:−\:\mathrm{1}\right)\:\mathrm{equal}\:\mathrm{point}\:\mathrm{masses}\:\mathrm{each}\:\mathrm{of}\:\mathrm{mass} \\ $$$${m}\:\mathrm{are}\:\mathrm{placed}\:\mathrm{at}\:\mathrm{the}\:\mathrm{vertices}\:\mathrm{of}\:\mathrm{a}\:\mathrm{regular} \\ $$$${n}-\mathrm{polygon}.\:\mathrm{The}\:\mathrm{vacant}\:\mathrm{vertex}\:\mathrm{has}\:\mathrm{a} \\ $$$$\mathrm{position}\:\mathrm{vector}\:{a}\:\mathrm{with}\:\mathrm{respect}\:\mathrm{to}\:\mathrm{the} \\ $$$$\mathrm{centre}\:\mathrm{of}\:\mathrm{the}\:\mathrm{polygon}.\:\mathrm{Find}\:\mathrm{the}\:\mathrm{position} \\ $$$$\mathrm{vector}\:\mathrm{of}\:\mathrm{centre}\:\mathrm{of}\:\mathrm{mass}. \\ $$

Question Number 24184 Answers: 0 Comments: 9

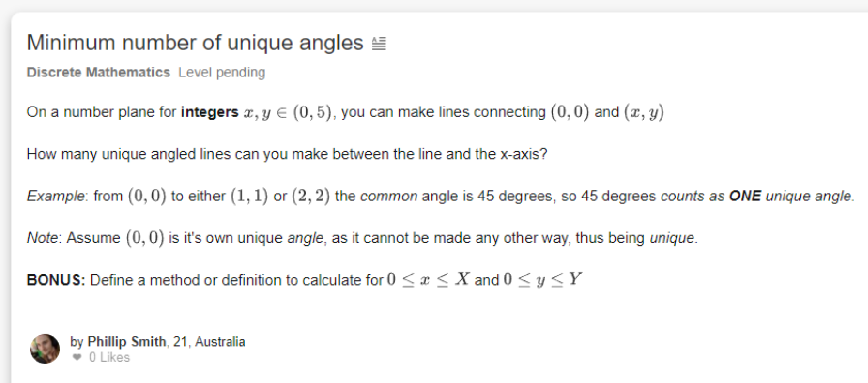
Question Number 24181 Answers: 1 Comments: 0

if the points C(−1,2) divides internally the line segment joining the points A(2,5) and B(x,y) in the ratio 3:4 find the value of x^2 +y^2

$${if}\:{the}\:{points}\:{C}\left(−\mathrm{1},\mathrm{2}\right)\:{divides}\:{internally}\:{the}\:{line}\:{segment}\:{joining}\:{the}\:{points}\:{A}\left(\mathrm{2},\mathrm{5}\right)\:{and}\:{B}\left({x},{y}\right)\:{in}\:{the}\:{ratio}\:\mathrm{3}:\mathrm{4}\:{find}\:{the}\:{value}\:{of}\:{x}^{\mathrm{2}} +{y}^{\mathrm{2}} \\ $$

Question Number 24178 Answers: 0 Comments: 6

A block is released from rest at the top of a frictionless incline plane 16.00m long.It reaches the bottom 4.0s later.A second block is projected up the plane from the bottom at the instant the first one is released in such a way that it returns to the bottom simultaneously with the first block.Find: a)the acceleration of each block on the incline plane b)the initial velocity of the first block. c)how far up the incline plane did the second block travel. d)What angle does the plane makes with the horizontal. (g=10m/s^2 )

$${A}\:{block}\:{is}\:{released}\:{from}\:{rest}\:{at}\:{the} \\ $$$${top}\:{of}\:{a}\:{frictionless}\:{incline} \\ $$$${plane}\:\mathrm{16}.\mathrm{00}{m}\:{long}.{It}\:{reaches}\:{the} \\ $$$${bottom}\:\mathrm{4}.\mathrm{0}{s}\:{later}.{A}\:{second}\:{block} \\ $$$${is}\:{projected}\:{up}\:{the}\:{plane}\:{from}\:{the} \\ $$$${bottom}\:{at}\:{the}\:{instant}\:{the}\:{first}\:{one} \\ $$$${is}\:{released}\:{in}\:{such}\:{a}\:{way}\:{that}\:{it} \\ $$$${returns}\:{to}\:{the}\:{bottom}\:{simultaneously} \\ $$$${with}\:{the}\:{first}\:{block}.{Find}: \\ $$$$\left.{a}\right){the}\:{acceleration}\:{of}\:{each}\:{block} \\ $$$${on}\:{the}\:{incline}\:{plane} \\ $$$$\left.{b}\right){the}\:{initial}\:{velocity}\:{of}\:{the}\:{first} \\ $$$${block}. \\ $$$$\left.{c}\right){how}\:{far}\:{up}\:{the}\:{incline}\:{plane} \\ $$$${did}\:{the}\:{second}\:{block}\:{travel}. \\ $$$$\left.{d}\right){What}\:{angle}\:{does}\:{the}\:{plane}\:{makes} \\ $$$${with}\:{the}\:{horizontal}. \\ $$$$\left({g}=\mathrm{10}{m}/{s}^{\mathrm{2}} \right) \\ $$

Question Number 24177 Answers: 2 Comments: 1

A lorry goes round an unbanked curve.If the radius of the curve is 30m and the coefficient of friction between the ground and the tyre is 0.6. Calculate the maximum speed of the lorry. (g=10m/s^2 ) please buddies help

$${A}\:{lorry}\:{goes}\:{round}\:{an}\:{unbanked} \\ $$$${curve}.{If}\:{the}\:{radius}\:{of}\:{the}\:{curve} \\ $$$${is}\:\mathrm{30}{m}\:{and}\:{the}\:{coefficient}\:{of} \\ $$$${friction}\:{between}\:{the}\:{ground}\:{and} \\ $$$${the}\:{tyre}\:{is}\:\mathrm{0}.\mathrm{6}.\:{Calculate}\:{the} \\ $$$${maximum}\:{speed}\:{of}\:{the}\:{lorry}. \\ $$$$\left({g}=\mathrm{10}{m}/{s}^{\mathrm{2}} \right) \\ $$$$ \\ $$$$ \\ $$$${please}\:{buddies}\:{help} \\ $$

Question Number 24172 Answers: 1 Comments: 1

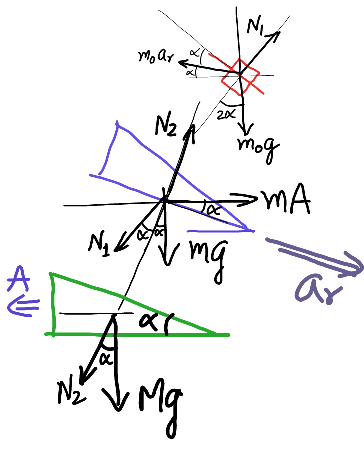
Question Number 24168 Answers: 0 Comments: 4

A plank of mass M kg is sliding on the smooth horizontal surface with constant velocity of 10 ms^(−1) . A another block of mass M kg is gently placed on it. The coefficient of friction between the block and the upper surface of the plank is 0.2. Assuming that plank is long enough such that the block does not fall from it. The velocity-time graph of the block is [Take g = 10 m/s^2 ]

$$\mathrm{A}\:\mathrm{plank}\:\mathrm{of}\:\mathrm{mass}\:{M}\:\mathrm{kg}\:\mathrm{is}\:\mathrm{sliding}\:\mathrm{on}\:\mathrm{the} \\ $$$$\mathrm{smooth}\:\mathrm{horizontal}\:\mathrm{surface}\:\mathrm{with}\:\mathrm{constant} \\ $$$$\mathrm{velocity}\:\mathrm{of}\:\mathrm{10}\:\mathrm{ms}^{−\mathrm{1}} .\:\mathrm{A}\:\mathrm{another}\:\mathrm{block}\:\mathrm{of} \\ $$$$\mathrm{mass}\:{M}\:\mathrm{kg}\:\mathrm{is}\:\mathrm{gently}\:\mathrm{placed}\:\mathrm{on}\:\mathrm{it}.\:\mathrm{The} \\ $$$$\mathrm{coefficient}\:\mathrm{of}\:\mathrm{friction}\:\mathrm{between}\:\mathrm{the} \\ $$$$\mathrm{block}\:\mathrm{and}\:\mathrm{the}\:\mathrm{upper}\:\mathrm{surface}\:\mathrm{of}\:\mathrm{the}\:\mathrm{plank} \\ $$$$\mathrm{is}\:\mathrm{0}.\mathrm{2}.\:\mathrm{Assuming}\:\mathrm{that}\:\mathrm{plank}\:\mathrm{is}\:\mathrm{long} \\ $$$$\mathrm{enough}\:\mathrm{such}\:\mathrm{that}\:\mathrm{the}\:\mathrm{block}\:\mathrm{does}\:\mathrm{not}\:\mathrm{fall} \\ $$$$\mathrm{from}\:\mathrm{it}.\:\mathrm{The}\:\mathrm{velocity}-\mathrm{time}\:\mathrm{graph}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{block}\:\mathrm{is}\:\left[\mathrm{Take}\:{g}\:=\:\mathrm{10}\:\mathrm{m}/\mathrm{s}^{\mathrm{2}} \right] \\ $$

Question Number 24164 Answers: 0 Comments: 1

Five moles of an ideal gas expand isothermally and reversibly from a pressure of 10 atm to 2 atm at 300 K. What is the largest mass (approx) which can be lifted through a height of 1 m in this expansion?

$$\mathrm{Five}\:\mathrm{moles}\:\mathrm{of}\:\mathrm{an}\:\mathrm{ideal}\:\mathrm{gas}\:\mathrm{expand} \\ $$$$\mathrm{isothermally}\:\mathrm{and}\:\mathrm{reversibly}\:\mathrm{from}\:\mathrm{a} \\ $$$$\mathrm{pressure}\:\mathrm{of}\:\mathrm{10}\:\mathrm{atm}\:\mathrm{to}\:\mathrm{2}\:\mathrm{atm}\:\mathrm{at}\:\mathrm{300}\:\mathrm{K}. \\ $$$$\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{largest}\:\mathrm{mass}\:\left(\mathrm{approx}\right) \\ $$$$\mathrm{which}\:\mathrm{can}\:\mathrm{be}\:\mathrm{lifted}\:\mathrm{through}\:\mathrm{a}\:\mathrm{height}\:\mathrm{of} \\ $$$$\mathrm{1}\:\mathrm{m}\:\mathrm{in}\:\mathrm{this}\:\mathrm{expansion}? \\ $$

Question Number 24161 Answers: 2 Comments: 1

A door is hinged at one end and is free to rotate about a vertical axis. Does its weight cause any torque about this axis? Give reason for your answer.

$$\mathrm{A}\:\mathrm{door}\:\mathrm{is}\:\mathrm{hinged}\:\mathrm{at}\:\mathrm{one}\:\mathrm{end}\:\mathrm{and}\:\mathrm{is}\:\mathrm{free} \\ $$$$\mathrm{to}\:\mathrm{rotate}\:\mathrm{about}\:\mathrm{a}\:\mathrm{vertical}\:\mathrm{axis}.\:\mathrm{Does}\:\mathrm{its} \\ $$$$\mathrm{weight}\:\mathrm{cause}\:\mathrm{any}\:\mathrm{torque}\:\mathrm{about}\:\mathrm{this} \\ $$$$\mathrm{axis}?\:\mathrm{Give}\:\mathrm{reason}\:\mathrm{for}\:\mathrm{your}\:\mathrm{answer}. \\ $$

Question Number 24179 Answers: 1 Comments: 0

the line segment joining the points (3,−4) and (1,2) is trisected at the points P and Q if the coordinates of P and Q are (p,−2) and (5/3,q) respectively find the values of p and q.

$${the}\:{line}\:{segment}\:{joining}\:{the}\:{points}\:\left(\mathrm{3},−\mathrm{4}\right)\:{and}\:\left(\mathrm{1},\mathrm{2}\right)\:{is}\:{trisected}\:{at}\:{the}\:{points}\:{P}\:{and}\:{Q}\:{if}\:{the}\:{coordinates}\:{of}\:{P}\:{and}\:{Q}\:{are}\:\left({p},−\mathrm{2}\right)\:{and}\:\left(\mathrm{5}/\mathrm{3},{q}\right)\:{respectively}\:{find}\:{the}\:{values}\:{of}\:{p}\:{and}\:{q}. \\ $$

Question Number 24152 Answers: 0 Comments: 1

Let matrice A = ((a,b),(c,d) ), and A^T = A^(−1) Find d − bc

$$\mathrm{Let}\:\mathrm{matrice}\:{A}\:=\:\begin{pmatrix}{{a}}&{{b}}\\{{c}}&{{d}}\end{pmatrix},\:\mathrm{and}\:{A}^{{T}} \:=\:{A}^{−\mathrm{1}} \\ $$$$\mathrm{Find}\:{d}\:−\:{bc} \\ $$

Pg 1843 Pg 1844 Pg 1845 Pg 1846 Pg 1847 Pg 1848 Pg 1849 Pg 1850 Pg 1851 Pg 1852

Contact: info@tinkutara.com