
AllQuestion and Answers: Page 1835
Question Number 25865 Answers: 1 Comments: 0
Question Number 25846 Answers: 0 Comments: 3
Question Number 25845 Answers: 2 Comments: 0
Question Number 25842 Answers: 2 Comments: 2
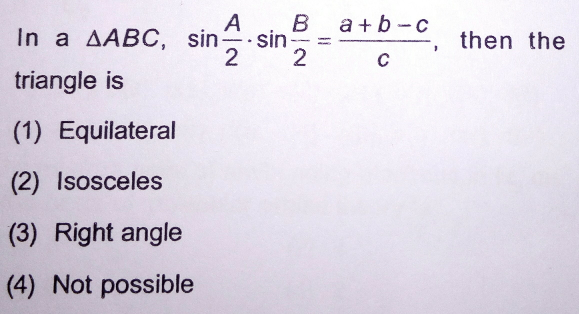
Question Number 25838 Answers: 0 Comments: 4

Question Number 25837 Answers: 1 Comments: 0
Question Number 25854 Answers: 1 Comments: 0
$${find}\:\frac{{dy}}{{dx}}\:{for}\:{y}=\sqrt{{x}+\sqrt{{x}+\sqrt{{x}+\sqrt{{x}.........}}}} \\ $$
Question Number 25828 Answers: 2 Comments: 1
Question Number 25825 Answers: 1 Comments: 0
Question Number 25824 Answers: 0 Comments: 0
Question Number 25823 Answers: 0 Comments: 1
Question Number 25822 Answers: 0 Comments: 0
Question Number 25821 Answers: 0 Comments: 0
Question Number 25814 Answers: 1 Comments: 0
Question Number 25811 Answers: 0 Comments: 1
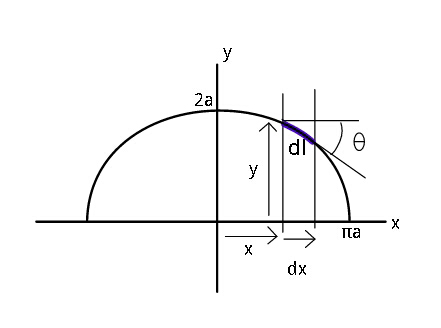
Question Number 25800 Answers: 2 Comments: 0
Question Number 25796 Answers: 0 Comments: 0
Question Number 26411 Answers: 1 Comments: 0
Question Number 26410 Answers: 1 Comments: 0
Question Number 25787 Answers: 0 Comments: 2
$$\mathrm{2}^{{x}} ={x}^{\mathrm{2}\:} ,{hence}\:{x}=\mathrm{2}\:.\:{prove} \\ $$
Question Number 25786 Answers: 1 Comments: 0
Question Number 25783 Answers: 1 Comments: 0
Question Number 26952 Answers: 1 Comments: 0

Question Number 26951 Answers: 1 Comments: 0

Question Number 25778 Answers: 1 Comments: 0
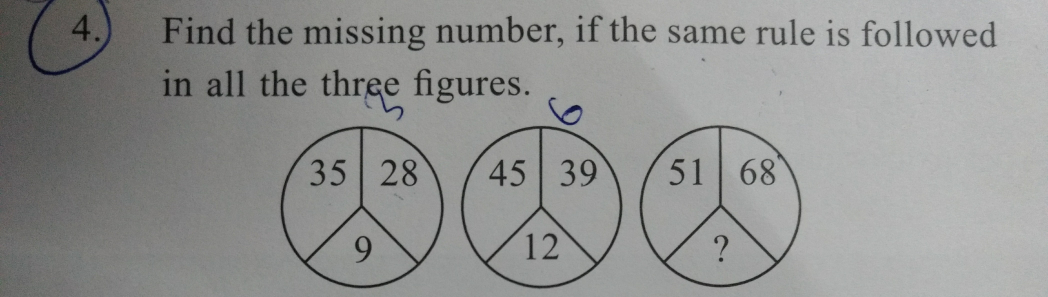
Question Number 25777 Answers: 1 Comments: 0
$$\int\frac{\mathrm{1}}{\mathrm{sin}\:{x}+\mathrm{cos}\:{x}}{dx} \\ $$
Pg 1830 Pg 1831 Pg 1832 Pg 1833 Pg 1834 Pg 1835 Pg 1836 Pg 1837 Pg 1838 Pg 1839
