
AllQuestion and Answers: Page 1833
Question Number 25949 Answers: 0 Comments: 0

Question Number 25948 Answers: 0 Comments: 1
$$\mathrm{Factorise}:\:\:{x}^{\mathrm{2}} +{y}^{\mathrm{2}} −{z}^{\mathrm{2}} −\mathrm{2}{xy} \\ $$
Question Number 25943 Answers: 1 Comments: 0

Question Number 26096 Answers: 0 Comments: 0

Question Number 25940 Answers: 0 Comments: 0
Question Number 25937 Answers: 1 Comments: 0
Question Number 25934 Answers: 1 Comments: 2
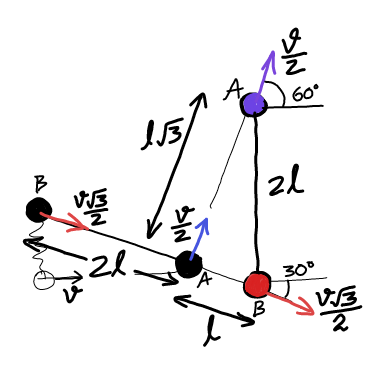
Question Number 25932 Answers: 0 Comments: 0
Question Number 25930 Answers: 2 Comments: 0
Question Number 25929 Answers: 0 Comments: 0
Question Number 25928 Answers: 0 Comments: 0
Question Number 25924 Answers: 1 Comments: 0
Question Number 25899 Answers: 1 Comments: 1
$$\mathrm{8}{a}^{\mathrm{6}} +\mathrm{5}{a}^{\mathrm{3}} +\mathrm{1}={factorize} \\ $$
Question Number 25895 Answers: 1 Comments: 0
Question Number 25894 Answers: 0 Comments: 1
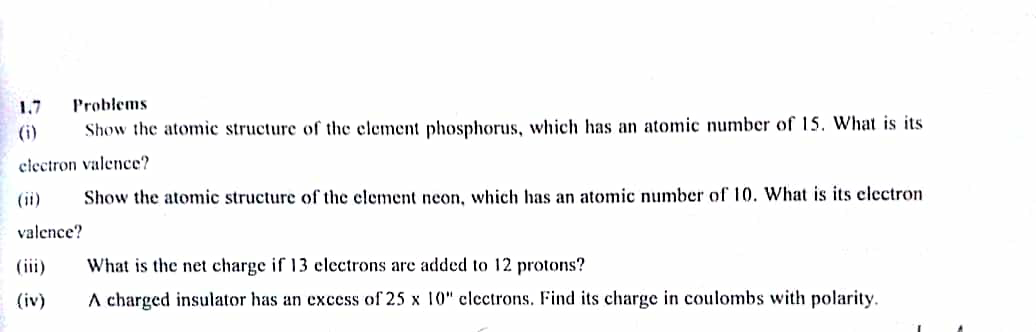
Question Number 25887 Answers: 1 Comments: 0

Question Number 25871 Answers: 0 Comments: 3

Question Number 25868 Answers: 0 Comments: 3

Question Number 25853 Answers: 0 Comments: 2
Question Number 25852 Answers: 0 Comments: 1
Question Number 25851 Answers: 0 Comments: 1
Question Number 25865 Answers: 1 Comments: 0
Question Number 25846 Answers: 0 Comments: 3
Question Number 25845 Answers: 2 Comments: 0
Question Number 25842 Answers: 2 Comments: 2
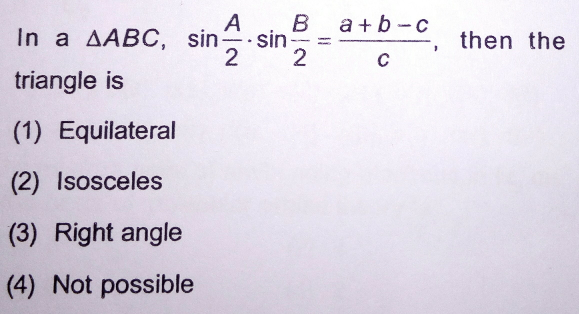
Question Number 25838 Answers: 0 Comments: 4

Pg 1828 Pg 1829 Pg 1830 Pg 1831 Pg 1832 Pg 1833 Pg 1834 Pg 1835 Pg 1836 Pg 1837
