
AllQuestion and Answers: Page 1832
Question Number 26059 Answers: 0 Comments: 0
Question Number 26058 Answers: 0 Comments: 0

Question Number 26057 Answers: 0 Comments: 1
Question Number 26055 Answers: 1 Comments: 0
Question Number 26054 Answers: 0 Comments: 0
Question Number 26053 Answers: 1 Comments: 0
Question Number 26050 Answers: 1 Comments: 0

Question Number 26047 Answers: 2 Comments: 6

Question Number 26046 Answers: 1 Comments: 0
Question Number 26042 Answers: 0 Comments: 2
Question Number 26040 Answers: 0 Comments: 0

Question Number 26110 Answers: 0 Comments: 0
Question Number 26036 Answers: 1 Comments: 0
Question Number 26031 Answers: 0 Comments: 0
Question Number 26024 Answers: 0 Comments: 0
Question Number 26023 Answers: 0 Comments: 0
Question Number 26021 Answers: 1 Comments: 1
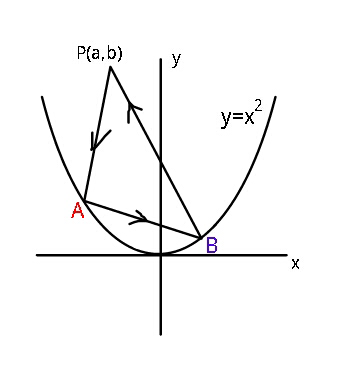
Question Number 26020 Answers: 0 Comments: 0
Question Number 26019 Answers: 0 Comments: 0

Question Number 26008 Answers: 2 Comments: 0

Question Number 26007 Answers: 1 Comments: 0

Question Number 25998 Answers: 1 Comments: 1

Question Number 26009 Answers: 1 Comments: 0

Question Number 25985 Answers: 1 Comments: 0
Question Number 26097 Answers: 0 Comments: 0
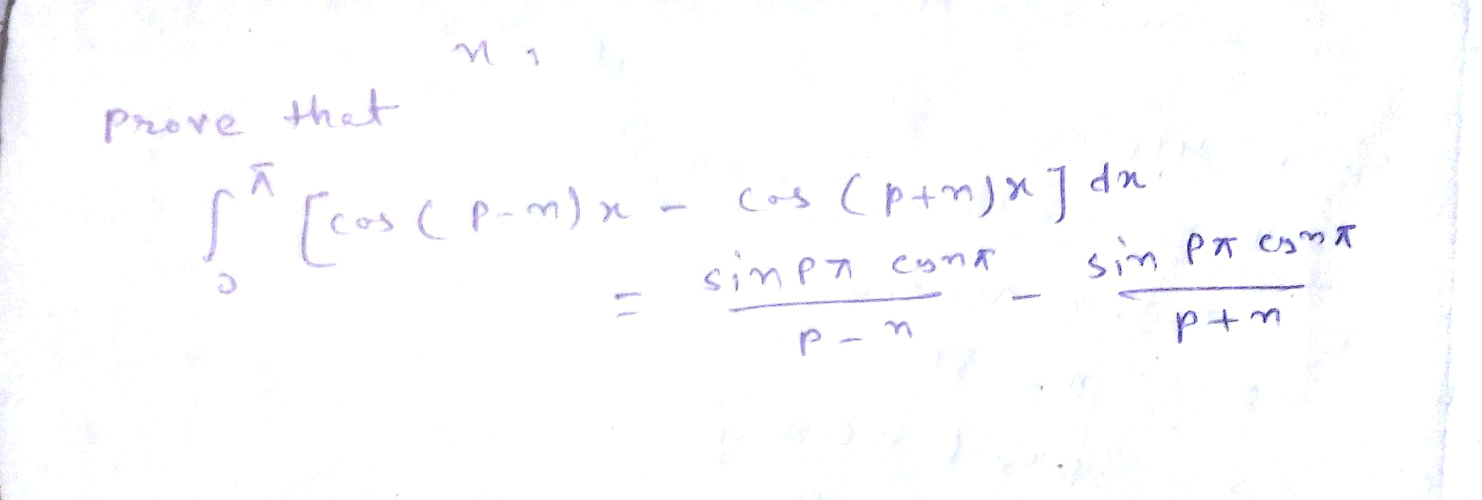
Question Number 25975 Answers: 1 Comments: 0

Pg 1827 Pg 1828 Pg 1829 Pg 1830 Pg 1831 Pg 1832 Pg 1833 Pg 1834 Pg 1835 Pg 1836
