Question and Answers Forum

AllQuestion and Answers: Page 1831

Question Number 26176 Answers: 0 Comments: 1

find the radius of convergence for the serie Σ_(n=0) ^∝ e^(−(√n)) z^n ...z from C.

$${find}\:{the}\:{radius}\:{of}\:{convergence}\:{for}\:{the} \\ $$$${serie}\:\sum_{{n}=\mathrm{0}} ^{\propto} {e}^{−\sqrt{{n}}} \:{z}^{{n}} \:\:...{z}\:{from}\:{C}. \\ $$

Question Number 26174 Answers: 0 Comments: 0

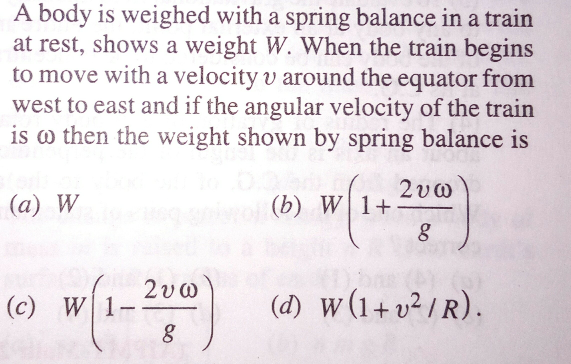
Question Number 26162 Answers: 1 Comments: 0

Find the volume of the solid generated when the region enclosed by y= (√x) y=0 and x=9 about the line x=9

$${Find}\:\:{the}\:{volume}\:\:{of}\:{the}\:{solid}\:{generated}\:{when}\:{the}\:{region}\:\:{enclosed} \\ $$$${by}\:\:{y}=\:\sqrt{{x}} \\ $$$$\:{y}=\mathrm{0}\:\:{and}\:\:{x}=\mathrm{9}\:{about}\:{the}\:\:{line}\:{x}=\mathrm{9} \\ $$

Question Number 26166 Answers: 0 Comments: 0

Question Number 26159 Answers: 1 Comments: 0

If x^2 + y^2 + 2xy + 2x + 2y + k = 0 represents pair of straight lines then find the value of k.

$$\mathrm{If}\:{x}^{\mathrm{2}} \:+\:{y}^{\mathrm{2}} \:+\:\mathrm{2}{xy}\:+\:\mathrm{2}{x}\:+\:\mathrm{2}{y}\:+\:{k}\:=\:\mathrm{0} \\ $$$$\mathrm{represents}\:\mathrm{pair}\:\mathrm{of}\:\mathrm{straight}\:\mathrm{lines}\:\mathrm{then} \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:{k}. \\ $$

Question Number 26156 Answers: 1 Comments: 0

a two digit number is 3 more than 4 times the sum of its digits. if 18 is added to this number .the sum is equal to number obtained by interchanging the digits.find the number

$${a}\:{two}\:{digit}\:{number}\:{is}\:\mathrm{3}\:{more}\:{than} \\ $$$$\mathrm{4}\:{times}\:{the}\:{sum}\:{of}\:{its}\:{digits}.\:{if} \\ $$$$\mathrm{18}\:{is}\:{added}\:{to}\:{this}\:{number}\:.{the}\: \\ $$$${sum}\:{is}\:{equal}\:{to}\:{number}\:{obtained} \\ $$$${by}\:{interchanging}\:{the}\:{digits}.{find}\:{the}\:{number} \\ $$

Question Number 26153 Answers: 1 Comments: 0

ratio of income of two persons is 9 is to 7.ratio of their expenses is 4 is to 3 .every person saves rupees 200. find income of each.

$${ratio}\:{of}\:{income}\:{of}\:{two}\:{persons}\:{is} \\ $$$$\mathrm{9}\:{is}\:{to}\:\mathrm{7}.{ratio}\:{of}\:{their}\:{expenses} \\ $$$${is}\:\mathrm{4}\:{is}\:{to}\:\mathrm{3}\:.{every}\:{person}\:{saves}\: \\ $$$${rupees}\:\mathrm{200}.\:{find}\:{income}\:{of}\:{each}. \\ $$

Question Number 26148 Answers: 2 Comments: 2

Question Number 26147 Answers: 1 Comments: 0

There are 5 more girls than boys in a class. If 2 boys join the class, the ratio of girls to boys will be 5:4. Find the number of of girls in the class.

$$\mathrm{There}\:\mathrm{are}\:\mathrm{5}\:\mathrm{more}\:\mathrm{girls}\:\mathrm{than}\:\mathrm{boys}\:\mathrm{in}\:\mathrm{a}\:\mathrm{class}.\:\mathrm{If}\:\mathrm{2}\:\mathrm{boys}\:\mathrm{join} \\ $$$$\mathrm{the}\:\mathrm{class},\:\mathrm{the}\:\mathrm{ratio}\:\mathrm{of}\:\mathrm{girls}\:\mathrm{to}\:\mathrm{boys}\:\mathrm{will}\:\mathrm{be}\:\mathrm{5}:\mathrm{4}.\:\mathrm{Find}\:\mathrm{the} \\ $$$$\mathrm{number}\:\mathrm{of}\:\mathrm{of}\:\mathrm{girls}\:\mathrm{in}\:\mathrm{the}\:\mathrm{class}. \\ $$

Question Number 26142 Answers: 0 Comments: 0

Prove that If f(x) is Riemann integrable on [a,b] and ∃M>0 s.t. ∀x∈[a,b] (f(x)≠0 and ∣f(x)∣<M and ∣(1/(f(x)))∣<M), then (1/(f(x))) is Riemann integrable on [a,b].

$$\mathrm{Prove}\:\mathrm{that}\: \\ $$$$\mathrm{If}\:{f}\left({x}\right)\:\mathrm{is}\:\mathrm{Riemann}\:\mathrm{integrable}\:\mathrm{on}\:\left[{a},{b}\right]\:\mathrm{and} \\ $$$$\:\:\:\:\:\exists{M}>\mathrm{0}\:{s}.{t}.\:\forall{x}\in\left[{a},{b}\right]\:\left({f}\left({x}\right)\neq\mathrm{0}\:{and}\:\mid{f}\left({x}\right)\mid<{M}\:{and}\:\mid\frac{\mathrm{1}}{{f}\left({x}\right)}\mid<{M}\right), \\ $$$$\mathrm{then}\:\frac{\mathrm{1}}{{f}\left({x}\right)}\:\mathrm{is}\:\mathrm{Riemann}\:\mathrm{integrable}\:\mathrm{on}\:\left[{a},{b}\right]. \\ $$

Question Number 26143 Answers: 2 Comments: 1

2000^(3000) vs 3000^(2000) who is stronger ?

$$\mathrm{2000}^{\mathrm{3000}} \:\:\boldsymbol{{vs}}\:\mathrm{3000}^{\mathrm{2000}} \\ $$$$ \\ $$$$\:\boldsymbol{{who}}\:\boldsymbol{{is}}\:\boldsymbol{{stronger}}\:? \\ $$

Question Number 26135 Answers: 1 Comments: 1

Prove that b=2asin^2 θ ; when acosθ−bsinθ=c and θ=45°

$$\mathrm{Prove}\:\mathrm{that}\:\mathrm{b}=\mathrm{2asin}^{\mathrm{2}} \theta\:; \\ $$$$\mathrm{when}\:\mathrm{acos}\theta−\mathrm{bsin}\theta=\mathrm{c}\:\mathrm{and}\:\theta=\mathrm{45}° \\ $$

Question Number 26133 Answers: 1 Comments: 1

find the value of (C_n ^(0 ) )^2 +(C_n ^1 )^2 +(C_n ^2 )^2 +...(C_n ^n )^2 .

$${find}\:{the}\:{value}\:{of}\:\:\left({C}_{{n}} ^{\mathrm{0}\:\:} \right)^{\mathrm{2}} \:+\left({C}_{{n}} ^{\mathrm{1}} \right)^{\mathrm{2}} \:+\left({C}_{{n}} ^{\mathrm{2}} \right)^{\mathrm{2}} \:+...\left({C}_{{n}} ^{{n}} \right)^{\mathrm{2}} . \\ $$

Question Number 26132 Answers: 0 Comments: 1

let put S_n =Σ_(k=1) ^(k=n) (((−1)^k )/k) find S_(n ) in terms of H_n then lim_(n−>∝) S_n H_n = Σ_(k=1) ^(k=n) (1/k) ( harmonic serie)

$${let}\:{put}\:{S}_{{n}} \:=\sum_{{k}=\mathrm{1}} ^{{k}={n}} \:\frac{\left(−\mathrm{1}\right)^{{k}} }{{k}} \\ $$$${find}\:{S}_{{n}\:} {in}\:{terms}\:{of}\:\:{H}_{{n}} \:{then}\:{lim}_{{n}−>\propto} \:{S}_{{n}} \\ $$$${H}_{{n}} \:=\:\sum_{{k}=\mathrm{1}} ^{{k}={n}} \frac{\mathrm{1}}{{k}}\:\:\:\left(\:{harmonic}\:{serie}\right) \\ $$

Question Number 26127 Answers: 1 Comments: 0

y+2y^3 y^((1)) =(x+4yln (y))y^((1))

$${y}+\mathrm{2}{y}^{\mathrm{3}} {y}^{\left(\mathrm{1}\right)} =\left({x}+\mathrm{4}{y}\mathrm{ln}\:\left({y}\right)\right){y}^{\left(\mathrm{1}\right)} \\ $$

Question Number 26125 Answers: 0 Comments: 1

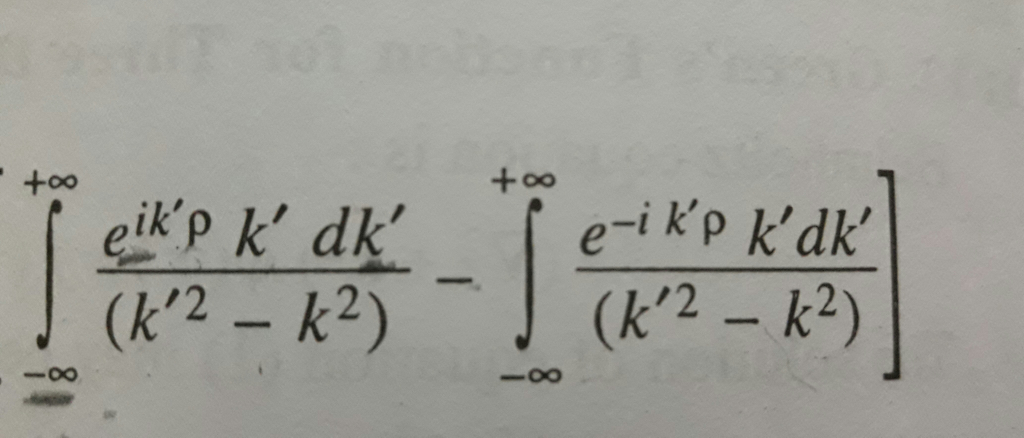
Question Number 26121 Answers: 0 Comments: 0

answer to26109 S_n = Σ_(k=1) ^(k=n) (1/(k^2 (k+1)^2 )) we decompose F(X) = (1/(X^2 (X+1)^2 )) = (a/X) +(b/X^2^ ) +(c/(X+1)) +(d/((X+1)^2 )) we find F(X) = ((−2)/X) +(1/X^2 ) + (2/(X+1)) + (1/((X+1)^2 )) so S_n = −2Σ_(k=1) ^(k=n) (1/k) +2Σ_(k=1) ^(k=n) (1/(k+1)) +Σ_(k=1) ^(k=n) (1/k^2^ ) + Σ_(k=1) ^(k=n) (1/((k+1)^2 )) but Σ_(k=1) ^(k=n) (1/k) = H_n Σ_(k=1) ^(k=n) (1/(k+1))= H_(n+1) −1 Σ_(k=1) ^(k=n) (1/((k+1)^2 )) = Σ_(k=1) ^(k=n) (1/k^2 ) + (1/((n+1)^2 )) −1⇒ S_n = 2(H_(n+1) −H_n ) +2 Σ_(k=1) ^(k=n) (1/k^2 ) −3 but lim_(n−>∝) (H_(n+1) − H_n ) =0 and Σ_(k=1) ^∝ (1/k^2 ) = (π^2 /6) ⇒ lim_(n−>∝) S_n = 2 (π^2 /6) −3 = (π^2 /3) −3 .

$${answer}\:{to}\mathrm{26109}\:\:\:{S}_{{n}} =\:\sum_{{k}=\mathrm{1}} ^{{k}={n}} \:\:\frac{\mathrm{1}}{{k}^{\mathrm{2}} \left({k}+\mathrm{1}\right)^{\mathrm{2}} }\:\:{we}\:{decompose} \\ $$$${F}\left({X}\right)\:\:=\:\:\frac{\mathrm{1}}{{X}^{\mathrm{2}} \left({X}+\mathrm{1}\right)^{\mathrm{2}} }\:=\:\:\frac{{a}}{{X}}\:\:\:+\frac{{b}}{{X}^{\mathrm{2}^{} } }\:\:+\frac{{c}}{{X}+\mathrm{1}}\:\:+\frac{{d}}{\left({X}+\mathrm{1}\right)^{\mathrm{2}} }\:\:{we}\:{find} \\ $$$${F}\left({X}\right)\:\:=\:\:\frac{−\mathrm{2}}{{X}}\:\:+\frac{\mathrm{1}}{{X}^{\mathrm{2}} }\:\:+\:\frac{\mathrm{2}}{{X}+\mathrm{1}}\:\:+\:\:\frac{\mathrm{1}}{\left({X}+\mathrm{1}\right)^{\mathrm{2}} }\:\:{so} \\ $$$$\:\:{S}_{{n}} \:\:=\:\:−\mathrm{2}\sum_{{k}=\mathrm{1}} ^{{k}={n}} \:\:\frac{\mathrm{1}}{{k}}\:\:+\mathrm{2}\sum_{{k}=\mathrm{1}} ^{{k}={n}} \:\frac{\mathrm{1}}{{k}+\mathrm{1}}\:\:+\sum_{{k}=\mathrm{1}} ^{{k}={n}} \:\:\frac{\mathrm{1}}{{k}^{\mathrm{2}^{} } }\:\:+\:\:\sum_{{k}=\mathrm{1}} ^{{k}={n}} \:\frac{\mathrm{1}}{\left({k}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$$${but}\:\:\:\sum_{{k}=\mathrm{1}} ^{{k}={n}} \:\frac{\mathrm{1}}{{k}}\:\:=\:{H}_{{n}} \\ $$$$\sum_{{k}=\mathrm{1}} ^{{k}={n}} \:\:\frac{\mathrm{1}}{{k}+\mathrm{1}}=\:\:{H}_{{n}+\mathrm{1}} \:\:−\mathrm{1} \\ $$$$\sum_{{k}=\mathrm{1}} ^{{k}={n}} \:\:\frac{\mathrm{1}}{\left({k}+\mathrm{1}\right)^{\mathrm{2}} }\:\:=\:\:\:\sum_{{k}=\mathrm{1}} ^{{k}={n}} \:\:\frac{\mathrm{1}}{{k}^{\mathrm{2}} }\:\:+\:\:\:\frac{\mathrm{1}}{\left({n}+\mathrm{1}\right)^{\mathrm{2}} }\:\:\:−\mathrm{1}\Rightarrow \\ $$$${S}_{{n}} \:\:=\:\:\mathrm{2}\left({H}_{{n}+\mathrm{1}} \:\:−{H}_{{n}} \right)\:\:+\mathrm{2}\:\:\sum_{{k}=\mathrm{1}} ^{{k}={n}} \:\:\frac{\mathrm{1}}{{k}^{\mathrm{2}} }\:\:\:−\mathrm{3} \\ $$$${but}\:\:\:{lim}_{{n}−>\propto} \left({H}_{{n}+\mathrm{1}} −\:{H}_{{n}} \right)\:\:=\mathrm{0}\:\:\:{and}\:\:\:\:\sum_{{k}=\mathrm{1}} ^{\propto} \:\:\frac{\mathrm{1}}{{k}^{\mathrm{2}} }\:\:=\:\frac{\pi^{\mathrm{2}} }{\mathrm{6}} \\ $$$$\Rightarrow\:\:\:{lim}_{{n}−>\propto} \:\:{S}_{{n}} \:\:=\:\mathrm{2}\:\frac{\pi^{\mathrm{2}} }{\mathrm{6}}\:\:−\mathrm{3}\:\:=\:\:\frac{\pi^{\mathrm{2}} }{\mathrm{3}}\:\:−\mathrm{3}\:\:\:\:\:. \\ $$

Question Number 26117 Answers: 1 Comments: 0

(1/6)(√((3log1728)/(1+(1/2)log36+(1/3)log8))) simplify the question above

$$\frac{\mathrm{1}}{\mathrm{6}}\sqrt{\frac{\mathrm{3}{log}\mathrm{1728}}{\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}}{log}\mathrm{36}+\frac{\mathrm{1}}{\mathrm{3}}{log}\mathrm{8}}} \\ $$$${simplify}\:{the}\:{question}\:{above} \\ $$

Question Number 26115 Answers: 1 Comments: 0

x^2 +(1/x^2 )=3 thrn find the valu of( x−(1/x))^2

$$\mathrm{x}^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{x}^{\mathrm{2}} }=\mathrm{3}\:\mathrm{thrn}\:\mathrm{find}\:\mathrm{the}\:\mathrm{valu}\:\mathrm{of}\left(\:\mathrm{x}−\frac{\mathrm{1}}{\mathrm{x}}\right)^{\mathrm{2}} \\ $$

Question Number 26126 Answers: 0 Comments: 1

using 1st principle find the derivative of y=x^x

$${using}\:\mathrm{1}{st}\:{principle}\:{find}\:{the} \\ $$$${derivative}\:{of} \\ $$$$\:\:\:\:\:\:\:\:{y}={x}^{{x}} \\ $$

Question Number 26113 Answers: 1 Comments: 0

Question Number 26107 Answers: 0 Comments: 0

answer to 26024 let put c= ∫_0 ^∞ cos(ax^2 )dx and c = ∫_0 ^∞ sin(ax^2 )dx ew have c−is = ∫_0 ^∞ e^(−iax^2 ) dx =2^(−1) ∫_R e^(−iax^2 ) dx and i put x^(1/2) =r(x)(notation) so 2(c−is) = ∫_R e^(−(r(ia)x)^2 ) dx and by the changement t= r(ia) x we find 2(c+is) = (r(ia))^(−1) ∫_R e^(−t^2 ) dt = r(π)/r(ia) but r(ia) =r(i) r(a) = r(a) e^ −−>2(c+is) = r(π) r(a)^(−1) e^(−iπ/4) ^) −−> c = r(2π)/_(4r(a)) and s = r(2π)/_(4r(a))

$${answer}\:{to}\:\mathrm{26024}\:\:\:\:{let}\:\:{put}\:{c}=\:\int_{\mathrm{0}} ^{\infty} \:{cos}\left({ax}^{\mathrm{2}} \right){dx}\:\:\:{and}\:\:\:{c}\:=\:\:\int_{\mathrm{0}} ^{\infty} \:{sin}\left({ax}^{\mathrm{2}} \right){dx} \\ $$$${ew}\:{have}\:\:{c}−{is}\:\:=\:\:\:\int_{\mathrm{0}} ^{\infty} \:\:{e}^{−{iax}^{\mathrm{2}} } {dx}\:\:\:=\mathrm{2}^{−\mathrm{1}} \:\:\int_{{R}} \:{e}^{−{iax}^{\mathrm{2}} } {dx}\:\:\:{and}\:\:{i}\:{put} \\ $$$${x}^{\mathrm{1}/\mathrm{2}} \:={r}\left({x}\right)\left({notation}\right)\:\:{so}\:\:\mathrm{2}\left({c}−{is}\right)\:\:=\:\:\:\int_{{R}} \:\:{e}^{−\left({r}\left({ia}\right){x}\right)^{\mathrm{2}} } {dx} \\ $$$${and}\:{by}\:{the}\:{changement}\:\:\:{t}=\:{r}\left({ia}\right)\:{x}\:\:\:{we}\:{find} \\ $$$$\mathrm{2}\left({c}+{is}\right)\:\:=\:\:\:\left({r}\left({ia}\right)\right)^{−\mathrm{1}} \:\int_{{R}} \:{e}^{−{t}^{\mathrm{2}} } {dt}\:\:\:=\:\:{r}\left(\pi\right)/{r}\left({ia}\right)\:\:{but} \\ $$$${r}\left({ia}\right)\:\:={r}\left({i}\right)\:{r}\left({a}\right)\:\:=\:\:{r}\left({a}\right)\:{e}^{} \:\:\:−−>\mathrm{2}\left({c}+{is}\right)\:\:\:\:=\:\:{r}\left(\pi\right)\:{r}\left({a}\right)^{−\mathrm{1}} \:\:{e}^{−{i}\pi/\mathrm{4}} \:\:^{\left.\right)} \\ $$$$−−>\:\:{c}\:=\:\:\:{r}\left(\mathrm{2}\pi\right)/_{\mathrm{4}{r}\left({a}\right)} \:\:{and}\:\:\:{s}\:\:=\:\:{r}\left(\mathrm{2}\pi\right)/_{\mathrm{4}{r}\left({a}\right)} \\ $$

Question Number 26111 Answers: 0 Comments: 2

find the radius of convergence for the serie Σ_(n=1) ^∝ H_n x^n H_n = Σ_(k=1) ^(k=n) (1/k) .

$${find}\:\:{the}\:{radius}\:{of}\:{convergence}\:{for}\:{the}\:{serie}\:\:\:\sum_{{n}=\mathrm{1}} ^{\propto} \:{H}_{{n}} \:{x}^{{n}} \\ $$$${H}_{{n}} \:\:=\:\:\:\sum_{{k}=\mathrm{1}} ^{{k}={n}} \:\:\frac{\mathrm{1}}{{k}}\:. \\ $$

Question Number 26223 Answers: 1 Comments: 1

let put ξ(x)= Σ_(n=1) ^∝ (1/n^x ) with x>1 and δ(x) =Σ_(n=1) ^∝ (((−1)^n )/n^x ) find a relation between ξ(x) and δ(x).

$${let}\:{put}\:\xi\left({x}\right)=\:\:\sum_{{n}=\mathrm{1}} ^{\propto} \:\:\frac{\mathrm{1}}{{n}^{{x}} }\:\:{with}\:{x}>\mathrm{1} \\ $$$${and}\:\:\delta\left({x}\right)\:\:=\sum_{{n}=\mathrm{1}} ^{\propto} \:\:\frac{\left(−\mathrm{1}\right)^{{n}} }{{n}^{{x}} }\:\:\:{find}\:{a}\:{relation} \\ $$$${between}\:\xi\left({x}\right)\:{and}\:\delta\left({x}\right). \\ $$

Question Number 26098 Answers: 0 Comments: 0

Question Number 26090 Answers: 1 Comments: 0

∫((asin^3 θ+bcos^3 θ)/(sin^2 θ.cos^2 θ))dθ

$$\int\frac{{a}\mathrm{sin}\:^{\mathrm{3}} \theta+{b}\mathrm{cos}\:^{\mathrm{3}} \theta}{\mathrm{sin}^{\mathrm{2}} \:\theta.\mathrm{cos}^{\mathrm{2}} \:\theta}{d}\theta \\ $$

Pg 1826 Pg 1827 Pg 1828 Pg 1829 Pg 1830 Pg 1831 Pg 1832 Pg 1833 Pg 1834 Pg 1835

Contact: info@tinkutara.com