
AllQuestion and Answers: Page 1830
Question Number 26243 Answers: 0 Comments: 1
Question Number 26242 Answers: 0 Comments: 1
Question Number 26241 Answers: 0 Comments: 2
$$\int\sqrt{\mathrm{sin}\:\theta}{d}\theta \\ $$$${integration}\:?? \\ $$$${solve}\:{quickly} \\ $$
Question Number 26240 Answers: 1 Comments: 1
Question Number 26237 Answers: 1 Comments: 1
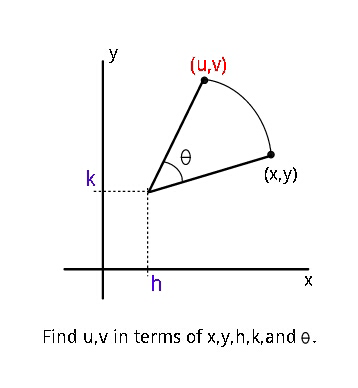
Question Number 26235 Answers: 1 Comments: 0
$${Find}\:{the}\:{real}\:{root}\:{of} \\ $$$$\:{x}^{\mathrm{2}} +\frac{\mathrm{1}}{{x}}={c}\:. \\ $$
Question Number 26229 Answers: 1 Comments: 1
Question Number 26228 Answers: 0 Comments: 1
Question Number 26227 Answers: 1 Comments: 0
Question Number 26222 Answers: 0 Comments: 2
Question Number 26224 Answers: 1 Comments: 1
Question Number 26218 Answers: 0 Comments: 2
$$\underset{\mathrm{2}} {\overset{\mathrm{4}} {\int}}\mathrm{sin}\theta\:{d}\theta \\ $$
Question Number 26207 Answers: 1 Comments: 0
Question Number 26205 Answers: 1 Comments: 1

Question Number 26200 Answers: 1 Comments: 0

Question Number 26198 Answers: 1 Comments: 0
Question Number 26192 Answers: 0 Comments: 3
Question Number 26191 Answers: 1 Comments: 0
$$\mathrm{2}\frac{{dy}}{{dx}}+{x}=\mathrm{4}\sqrt{{y}} \\ $$
Question Number 26181 Answers: 2 Comments: 0
Question Number 26179 Answers: 1 Comments: 0
Question Number 26176 Answers: 0 Comments: 1
Question Number 26174 Answers: 0 Comments: 0
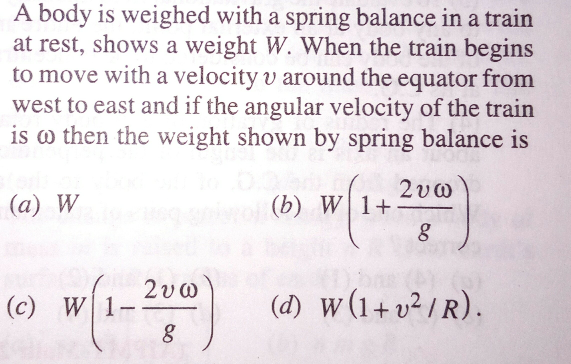
Question Number 26162 Answers: 1 Comments: 0
Question Number 26166 Answers: 0 Comments: 0

Question Number 26159 Answers: 1 Comments: 0
Question Number 26156 Answers: 1 Comments: 0
Pg 1825 Pg 1826 Pg 1827 Pg 1828 Pg 1829 Pg 1830 Pg 1831 Pg 1832 Pg 1833 Pg 1834
