Question and Answers Forum

AllQuestion and Answers: Page 1822

Question Number 27183 Answers: 1 Comments: 0

find the value of I= ∫_0 ^1 ((t−1)/(lnt))dt .

$${find}\:{the}\:{value}\:{of}\:{I}=\:\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{t}−\mathrm{1}}{{lnt}}{dt}\:. \\ $$

Question Number 27182 Answers: 0 Comments: 1

find the value of I_a = ∫∫_D_a e^(−((x^2 +y^2 )/2)) dxdy with D_a ={(x,y)∈R^2 / x^2 +y^2 ≤ a^2 }

$$\:{find}\:{the}\:{value}\:{of}\:{I}_{{a}} =\:\int\int_{{D}_{{a}} } {e}^{−\frac{{x}^{\mathrm{2}} \:+{y}^{\mathrm{2}} }{\mathrm{2}}} {dxdy}\:\:{with} \\ $$$${D}_{{a}} \:=\left\{\left({x},{y}\right)\in\mathbb{R}^{\mathrm{2}} \:/\:{x}^{\mathrm{2}} +{y}^{\mathrm{2}} \leqslant\:{a}^{\mathrm{2}} \:\:\right\} \\ $$

Question Number 27168 Answers: 0 Comments: 4

Question Number 27159 Answers: 0 Comments: 0

(√(1−x^(6 ) )) +(√(1−y^6 )) =k^3 (x^3 −y^3 ) then prove that (dy/dx)=((x^2 (√(1−x^2 )))/(y^2 (√(1−y^(2Δ) ))))

$$\sqrt{\mathrm{1}−{x}^{\mathrm{6}\:} \:}\:+\sqrt{\mathrm{1}−{y}^{\mathrm{6}} }\:={k}^{\mathrm{3}} \left({x}^{\mathrm{3}} −{y}^{\mathrm{3}} \right)\:\:\:{then}\:{prove}\:{that}\:\:\:\frac{{dy}}{{dx}}=\frac{{x}^{\mathrm{2}} \sqrt{\mathrm{1}−{x}^{\mathrm{2}} }}{{y}^{\mathrm{2}} \sqrt{\mathrm{1}−{y}^{\mathrm{2}\Delta} }} \\ $$$$ \\ $$$$ \\ $$

Question Number 27144 Answers: 1 Comments: 1

Let A={x,y,z} and B={1,2}. Find the number of relations from A to B.

$${Let}\:{A}=\left\{{x},{y},{z}\right\}\:{and}\:{B}=\left\{\mathrm{1},\mathrm{2}\right\}.\:{Find} \\ $$$${the}\:{number}\:{of}\:{relations}\:{from}\:{A}\:{to} \\ $$$${B}. \\ $$

Question Number 27128 Answers: 1 Comments: 0

A body resting on a rough horizontal plane require a pull of 18N inclined at 30° to the plane first to move it.It was found that a push of 22N inclined at 30° to the plane just moved the body. Determine the weight and coefficient of friction.

$${A}\:{body}\:{resting}\:{on}\:{a}\:{rough} \\ $$$${horizontal}\:{plane}\:{require}\:{a}\:{pull}\:{of} \\ $$$$\mathrm{18}{N}\:{inclined}\:{at}\:\mathrm{30}°\:{to}\:{the}\:{plane} \\ $$$${first}\:{to}\:{move}\:{it}.{It}\:{was}\:{found} \\ $$$${that}\:{a}\:{push}\:{of}\:\mathrm{22}{N}\:{inclined}\:{at}\:\mathrm{30}° \\ $$$${to}\:{the}\:{plane}\:{just}\:{moved}\:{the}\:{body}. \\ $$$${Determine}\:{the}\:{weight}\:{and}\: \\ $$$${coefficient}\:{of}\:{friction}. \\ $$

Question Number 27117 Answers: 1 Comments: 0

Question Number 27112 Answers: 0 Comments: 2

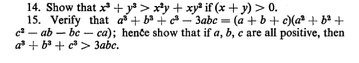
Question Number 27104 Answers: 0 Comments: 1

sin45^(o ) cos45^o +(√(3 sin 60°=?))

$$\mathrm{sin45}^{{o}\:} \mathrm{cos45}^{{o}} +\sqrt{\mathrm{3}\:\:\:\mathrm{sin}\:\mathrm{60}°=?} \\ $$

Question Number 27103 Answers: 0 Comments: 1

the intrest on a certain sum of money at the end of 6.25 year was (5/(16)) of the sum itself.what is the rate percent?

$$\mathrm{the}\:\mathrm{intrest}\:\mathrm{on}\:\mathrm{a}\:\mathrm{certain}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{money}\:\mathrm{at}\:\mathrm{the} \\ $$$$\mathrm{end}\:\mathrm{of}\:\mathrm{6}.\mathrm{25}\:\mathrm{year}\:\mathrm{was}\:\frac{\mathrm{5}}{\mathrm{16}}\:\mathrm{of}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{itself}.\mathrm{what} \\ $$$$\mathrm{is}\:\mathrm{the}\:\mathrm{rate}\:\mathrm{percent}? \\ $$

Question Number 27102 Answers: 1 Comments: 0

Question Number 27101 Answers: 1 Comments: 0

Question Number 27111 Answers: 1 Comments: 0

Question Number 27098 Answers: 0 Comments: 2

let give S(x) = Σ_(n=1) ^∝ (x^n /n) and W(x)= Σ_(n=1) ^∝ (((−1)^n x^n )/n^2 ) calculate S(x).W(x). in that we know /x/<1.

$${let}\:{give}\:{S}\left({x}\right)\:=\:\sum_{{n}=\mathrm{1}} ^{\propto} \frac{{x}^{{n}} }{{n}}\:\:{and}\:\:{W}\left({x}\right)=\:\:\sum_{{n}=\mathrm{1}} ^{\propto} \frac{\left(−\mathrm{1}\right)^{{n}} {x}^{{n}} }{{n}^{\mathrm{2}} } \\ $$$${calculate}\:\:\:{S}\left({x}\right).{W}\left({x}\right).\:\:\:{in}\:{that}\:{we}\:{know}\:/{x}/<\mathrm{1}. \\ $$

Question Number 27097 Answers: 1 Comments: 2

let give H_n = Σ_(k=1) ^(n ) (1/k) for p fixed from N find lim_(n−>∝) H_(n+p) − H_n .

$${let}\:{give}\:\:\:{H}_{{n}} \:=\:\sum_{{k}=\mathrm{1}} ^{{n}\:\:} \:\frac{\mathrm{1}}{{k}}\:\:\:\:{for}\:{p}\:\:{fixed}\:{from}\:\mathbb{N}\: \\ $$$${find}\:\:{lim}_{{n}−>\propto} \:\:{H}_{{n}+{p}} \:\:\:−\:\:{H}_{{n}} \:\:. \\ $$

Question Number 27094 Answers: 1 Comments: 2

if 1+x+x^2 =0 find the value of A= (x+(1/x))^6 +( x^2 +(1/x^2 ))^6 +... ( x^(100) +(1/x^(100) ))^6 .

$${if}\:\mathrm{1}+{x}+{x}^{\mathrm{2}} =\mathrm{0}\:{find}\:{the}\:{value}\:{of}\: \\ $$$${A}=\:\left({x}+\frac{\mathrm{1}}{{x}}\right)^{\mathrm{6}} \:+\left(\:{x}^{\mathrm{2}} +\frac{\mathrm{1}}{{x}^{\mathrm{2}} }\right)^{\mathrm{6}} \:\:+...\:\left(\:\:{x}^{\mathrm{100}} +\frac{\mathrm{1}}{{x}^{\mathrm{100}} }\right)^{\mathrm{6}} \:. \\ $$

Question Number 27083 Answers: 1 Comments: 0

∫3x^2 /x^6 +1

$$\int\mathrm{3}{x}^{\mathrm{2}} /{x}^{\mathrm{6}} +\mathrm{1} \\ $$$$ \\ $$

Question Number 27081 Answers: 1 Comments: 0

let give f(x)= (x/(4x^2 −1)) find f^((n)) (x) .

$${let}\:{give}\:{f}\left({x}\right)=\:\:\frac{{x}}{\mathrm{4}{x}^{\mathrm{2}} −\mathrm{1}}\:\:{find}\:{f}^{\left({n}\right)} \left({x}\right)\:\:. \\ $$

Question Number 27076 Answers: 0 Comments: 1

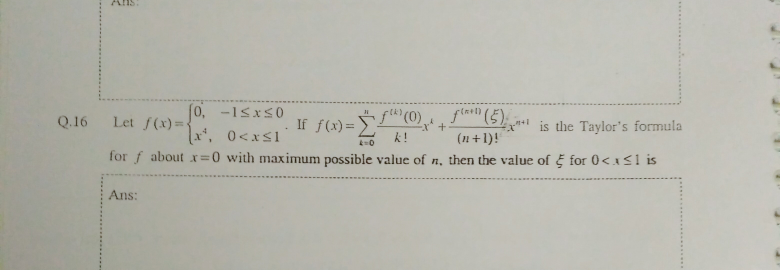
Question Number 27073 Answers: 1 Comments: 0

∫ln x×cos 2ln xdx

$$\int\mathrm{ln}\:{x}×\mathrm{cos}\:\mathrm{2ln}\:{xdx} \\ $$

Question Number 27075 Answers: 0 Comments: 0

Laws of Motion question at ibb.co/cqq1NG I tried uploading here but it doesn′t get uploaded.

$$\mathrm{Laws}\:\mathrm{of}\:\mathrm{Motion}\:\mathrm{question}\:\mathrm{at} \\ $$$$\mathrm{ibb}.\mathrm{co}/\mathrm{cqq1NG} \\ $$$$\mathrm{I}\:\mathrm{tried}\:\mathrm{uploading}\:\mathrm{here}\:\mathrm{but}\:\mathrm{it}\:\mathrm{doesn}'\mathrm{t} \\ $$$$\mathrm{get}\:\mathrm{uploaded}. \\ $$

Question Number 27074 Answers: 2 Comments: 0

Question Number 27065 Answers: 0 Comments: 0

Question Number 27057 Answers: 1 Comments: 3

Try to write new year number (2018)as: (i) Sum of two primes (ii)Sum of three primes (iii)Sum of primes (iv)Sum of as many distinct primes as possible.

$$\mathrm{Try}\:\mathrm{to}\:\mathrm{write}\:\mathrm{new}\:\mathrm{year}\:\mathrm{number} \\ $$$$\left(\mathrm{2018}\right)\mathrm{as}: \\ $$$$\left(\mathrm{i}\right)\:\mathrm{Sum}\:\mathrm{of}\:\mathrm{two}\:\mathrm{primes} \\ $$$$\left(\mathrm{ii}\right)\mathrm{Sum}\:\mathrm{of}\:\mathrm{three}\:\mathrm{primes} \\ $$$$\left(\mathrm{iii}\right)\mathrm{Sum}\:\mathrm{of}\:\mathrm{primes} \\ $$$$\left(\mathrm{iv}\right)\mathrm{Sum}\:\mathrm{of}\:\mathrm{as}\:\mathrm{many}\:\mathrm{distinct}\:\mathrm{primes}\:\mathrm{as} \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{possible}. \\ $$

Question Number 27055 Answers: 1 Comments: 1

Question Number 27050 Answers: 0 Comments: 2

Pg 1817 Pg 1818 Pg 1819 Pg 1820 Pg 1821 Pg 1822 Pg 1823 Pg 1824 Pg 1825 Pg 1826

Contact: info@tinkutara.com