Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 182

Question Number 201286 Answers: 0 Comments: 1

Question Number 201282 Answers: 1 Comments: 0

Question Number 201281 Answers: 0 Comments: 0

Question Number 201267 Answers: 0 Comments: 1

find ∫_0 ^1 (dx/((1+x)^(3/2) +(x−1)^(3/2) ))

$${find}\:\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{dx}}{\left(\mathrm{1}+{x}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} +\left({x}−\mathrm{1}\right)^{\frac{\mathrm{3}}{\mathrm{2}}} } \\ $$

Question Number 201266 Answers: 2 Comments: 0

calculate ∫_1 ^∞ (dx/( (√(1+x^3 ))))

$${calculate}\:\int_{\mathrm{1}} ^{\infty} \frac{{dx}}{\:\sqrt{\mathrm{1}+{x}^{\mathrm{3}} }} \\ $$

Question Number 201263 Answers: 2 Comments: 0

Q: the equation , sin^( 2) (x)−sin(mx)cos^2 (x)=1 has four distinct roots in ( 0 , 2π ) find the values of , m . (m ∈ N )

$$ \\ $$$$\:\:\:\:{Q}:\:{the}\:{equation}\:,\:{sin}^{\:\mathrm{2}} \left({x}\right)−{sin}\left({mx}\right){cos}\:^{\mathrm{2}} \left({x}\right)=\mathrm{1} \\ $$$$\:\:\:\:\:\:\:{has}\:\:{four}\:{distinct}\:{roots} \\ $$$$\:\:\:\:\:\:\:{in}\:\left(\:\mathrm{0}\:,\:\mathrm{2}\pi\:\right)\:\:{find}\:{the}\:{values} \\ $$$$\:\:\:\:\:\:\:\:{of}\:\:,\:\:\:{m}\:\:\:.\:\:\left({m}\:\in\:\mathbb{N}\:\right) \\ $$$$ \\ $$

Question Number 201258 Answers: 0 Comments: 0

Question Number 201257 Answers: 2 Comments: 0

Biggest prime factor of (3^(14) + 3^(13) − 12) = ?

$$\mathrm{Biggest}\:\mathrm{prime}\:\mathrm{factor}\:\mathrm{of}\:\left(\mathrm{3}^{\mathrm{14}} \:+\:\mathrm{3}^{\mathrm{13}} \:−\:\mathrm{12}\right)\:=\:? \\ $$

Question Number 201253 Answers: 2 Comments: 0

find the sum of n terms of the serice s_n =5+11+19+29+41+..........

$${find}\:{the}\:{sum}\:{of}\:{n}\:{terms}\:{of}\:{the}\:{serice} \\ $$$${s}_{{n}} =\mathrm{5}+\mathrm{11}+\mathrm{19}+\mathrm{29}+\mathrm{41}+.......... \\ $$

Question Number 201241 Answers: 0 Comments: 0

Question Number 201237 Answers: 1 Comments: 3

If , a ∣ 5b^( 2) −10b +1 ⇒ [ a , 5b ]_(lcm) = ?

$$ \\ $$$$\:\:\:\:{If}\:\:,\:\:\:{a}\:\mid\:\mathrm{5}{b}^{\:\mathrm{2}} −\mathrm{10}{b}\:+\mathrm{1}\: \\ $$$$\:\:\:\:\:\Rightarrow\:\:\:\left[\:{a}\:\:,\:\mathrm{5}{b}\:\right]_{\mathrm{lc}{m}} \:=\:\:?\: \\ $$$$ \\ $$$$ \\ $$

Question Number 201229 Answers: 1 Comments: 2

∫ (1/( (√(1+Inx))))dx

$$\int\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\boldsymbol{{Inx}}}}\boldsymbol{{dx}} \\ $$

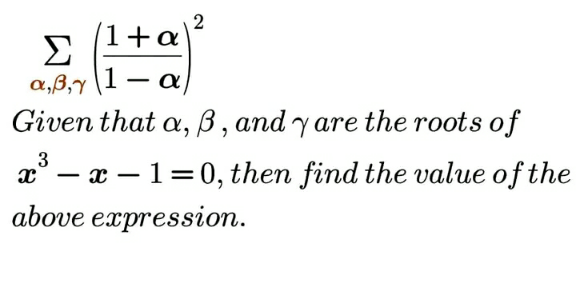
Question Number 201228 Answers: 0 Comments: 0

∫(√(x+(√(x+(√x))))) dx

$$\int\sqrt{\boldsymbol{{x}}+\sqrt{\boldsymbol{{x}}+\sqrt{\boldsymbol{{x}}}}}\:\boldsymbol{{dx}} \\ $$

Question Number 201227 Answers: 1 Comments: 0

∫ (((x^4 +x^7 )^(1/4) )/x^2 )dx

$$\:\int\:\frac{\left(\boldsymbol{{x}}^{\mathrm{4}} +\boldsymbol{{x}}^{\mathrm{7}} \right)^{\frac{\mathrm{1}}{\mathrm{4}}} }{\boldsymbol{{x}}^{\mathrm{2}} }\boldsymbol{{dx}} \\ $$

Question Number 201224 Answers: 2 Comments: 0

∫ (1/( (√(1+sinx))))dx

$$\int\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+\boldsymbol{{sinx}}}}\boldsymbol{{dx}} \\ $$

Question Number 201223 Answers: 1 Comments: 0

∫(√(1+(√(1+x)))) dx

$$\int\sqrt{\mathrm{1}+\sqrt{\mathrm{1}+\boldsymbol{{x}}}}\:\boldsymbol{{dx}} \\ $$

Question Number 201222 Answers: 1 Comments: 0

∫ (x^6 +x^9 )^(1/6) dx

$$\:\int\:\left(\boldsymbol{{x}}^{\mathrm{6}} +\boldsymbol{{x}}^{\mathrm{9}} \right)^{\frac{\mathrm{1}}{\mathrm{6}}} \boldsymbol{{dx}} \\ $$

Question Number 201221 Answers: 2 Comments: 0

Question Number 201214 Answers: 1 Comments: 0

A ball lies on the function z=xy at the point (1,2,2). Find the point in the xy−plane where the ball will touch it. (an unsolved old question Q200929)

$$\mathrm{A}\:\mathrm{ball}\:\mathrm{lies}\:\mathrm{on}\:\mathrm{the}\:\mathrm{function}\:{z}={xy}\:\mathrm{at} \\ $$$$\mathrm{the}\:\mathrm{point}\:\left(\mathrm{1},\mathrm{2},\mathrm{2}\right).\:\mathrm{Find}\:\mathrm{the}\:\mathrm{point}\:\mathrm{in} \\ $$$$\mathrm{the}\:{xy}−\mathrm{plane}\:\mathrm{where}\:\mathrm{the}\:\mathrm{ball}\:\mathrm{will} \\ $$$$\mathrm{touch}\:\mathrm{it}. \\ $$$$ \\ $$$$\left({an}\:{unsolved}\:{old}\:{question}\:{Q}\mathrm{200929}\right) \\ $$

Question Number 201209 Answers: 0 Comments: 4

Question Number 201200 Answers: 3 Comments: 2

Question Number 201192 Answers: 1 Comments: 0

Question Number 201190 Answers: 1 Comments: 0

Question Number 201185 Answers: 1 Comments: 0

Question Number 201172 Answers: 1 Comments: 0

Question Number 201166 Answers: 1 Comments: 0

Pg 177 Pg 178 Pg 179 Pg 180 Pg 181 Pg 182 Pg 183 Pg 184 Pg 185 Pg 186

Contact: info@tinkutara.com