Question and Answers Forum

AllQuestion and Answers: Page 1818

Question Number 27065 Answers: 0 Comments: 0

Question Number 27057 Answers: 1 Comments: 3

Try to write new year number (2018)as: (i) Sum of two primes (ii)Sum of three primes (iii)Sum of primes (iv)Sum of as many distinct primes as possible.

$$\mathrm{Try}\:\mathrm{to}\:\mathrm{write}\:\mathrm{new}\:\mathrm{year}\:\mathrm{number} \\ $$$$\left(\mathrm{2018}\right)\mathrm{as}: \\ $$$$\left(\mathrm{i}\right)\:\mathrm{Sum}\:\mathrm{of}\:\mathrm{two}\:\mathrm{primes} \\ $$$$\left(\mathrm{ii}\right)\mathrm{Sum}\:\mathrm{of}\:\mathrm{three}\:\mathrm{primes} \\ $$$$\left(\mathrm{iii}\right)\mathrm{Sum}\:\mathrm{of}\:\mathrm{primes} \\ $$$$\left(\mathrm{iv}\right)\mathrm{Sum}\:\mathrm{of}\:\mathrm{as}\:\mathrm{many}\:\mathrm{distinct}\:\mathrm{primes}\:\mathrm{as} \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{possible}. \\ $$

Question Number 27055 Answers: 1 Comments: 1

Question Number 27050 Answers: 0 Comments: 2

Question Number 27059 Answers: 0 Comments: 2

Question Number 27046 Answers: 0 Comments: 0

Considering y=x^3 +px+q If (dy/dx)∣_(x=α) =0 ⇒ α^2 =−(p/3) if ((d(y/x))/dx)∣_(x=β) =0 ⇒ β^( 3) =(q/2) roots of the cubic eq^n are: x=[−β^( 3) ±(√(β^( 6) −α^6 )) ]^(1/3) −[β^( 3) ±(√(β^( 6) −α^6 )) ]^(1/3) . Why such a connection? If equation is quadratic even_ y=ax^2 +bx+c (dy/dx)∣_(x=α) =0 ⇒ α=−(b/(2a)) ((d(y/x))/dx)∣_(x=β) =0 ⇒ β^( 2) =(c/a) roots of quadratic eq. are: x=𝛂±(√(𝛂^2 −𝛃^( 2) )) why such a connection ?

$${Considering}\:\boldsymbol{{y}}=\boldsymbol{{x}}^{\mathrm{3}} +\boldsymbol{{px}}+\boldsymbol{{q}} \\ $$$${If}\:\:\:\:\:\frac{{dy}}{{dx}}\mid_{{x}=\alpha} =\mathrm{0}\:\:\Rightarrow\:\:\alpha^{\mathrm{2}} =−\frac{{p}}{\mathrm{3}} \\ $$$${if}\:\:\:\frac{{d}\left({y}/{x}\right)}{{dx}}\mid_{{x}=\beta} =\mathrm{0}\:\:\:\Rightarrow\:\beta^{\:\mathrm{3}} =\frac{{q}}{\mathrm{2}} \\ $$$${roots}\:{of}\:{the}\:{cubic}\:\:{eq}^{{n}} \:{are}: \\ $$$$\:\:\:\:{x}=\left[−\beta^{\:\mathrm{3}} \pm\sqrt{\beta^{\:\mathrm{6}} −\alpha^{\mathrm{6}} }\:\right]^{\mathrm{1}/\mathrm{3}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:−\left[\beta^{\:\mathrm{3}} \pm\sqrt{\beta^{\:\mathrm{6}} −\alpha^{\mathrm{6}} }\:\right]^{\mathrm{1}/\mathrm{3}} \:. \\ $$$$\:{Why}\:{such}\:{a}\:{connection}? \\ $$$${If}\:{equation}\:{is}\:{quadratic}\:{even\_} \\ $$$$\:\:\:\:\boldsymbol{{y}}=\boldsymbol{{ax}}^{\mathrm{2}} +\boldsymbol{{bx}}+\boldsymbol{{c}} \\ $$$$\frac{{dy}}{{dx}}\mid_{{x}=\alpha} =\mathrm{0}\:\:\:\Rightarrow\:\:\alpha=−\frac{{b}}{\mathrm{2}{a}} \\ $$$$\:\:\:\:\:\:\frac{{d}\left({y}/{x}\right)}{{dx}}\mid_{{x}=\beta} =\mathrm{0}\:\:\Rightarrow\:\beta^{\:\mathrm{2}} =\frac{{c}}{{a}} \\ $$$${roots}\:{of}\:{quadratic}\:{eq}.\:{are}: \\ $$$$\:\:\:\:{x}=\boldsymbol{\alpha}\pm\sqrt{\boldsymbol{\alpha}^{\mathrm{2}} −\boldsymbol{\beta}^{\:\mathrm{2}} }\: \\ $$$${why}\:{such}\:{a}\:{connection}\:?\: \\ $$

Question Number 27044 Answers: 1 Comments: 0

Question Number 27061 Answers: 2 Comments: 1

Question Number 27060 Answers: 1 Comments: 1

Question Number 27045 Answers: 0 Comments: 0

find the value of ∫_(2/π) ^(6/π) x^3 cos([(1/x)])dx

$${find}\:{the}\:{value}\:{of}\:\int_{\frac{\mathrm{2}}{\pi}} ^{\frac{\mathrm{6}}{\pi}} \:{x}^{\mathrm{3}} \:{cos}\left(\left[\frac{\mathrm{1}}{{x}}\right]\right){dx} \\ $$

Question Number 27033 Answers: 1 Comments: 0

Question Number 27032 Answers: 0 Comments: 1

xy=(1−x^2 )(dy/dx) x=0 y=1

$${xy}=\left(\mathrm{1}−{x}^{\mathrm{2}} \right)\frac{{dy}}{{dx}}\:\:\:\:{x}=\mathrm{0}\:{y}=\mathrm{1} \\ $$

Question Number 27031 Answers: 1 Comments: 0

xy=(1−x^2 )(dy/dx) x=0 y=1

$${xy}=\left(\mathrm{1}−{x}^{\mathrm{2}} \right)\frac{{dy}}{{dx}}\:\:\:\:{x}=\mathrm{0}\:{y}=\mathrm{1} \\ $$

Question Number 27028 Answers: 1 Comments: 0

y^((2)) +4y=sinh x×sin 2x

$${y}^{\left(\mathrm{2}\right)} +\mathrm{4}{y}=\mathrm{sinh}\:{x}×\mathrm{sin}\:\mathrm{2}{x} \\ $$

Question Number 27016 Answers: 0 Comments: 1

the intrest on surtain sum of money at the end of 6.25 year was (5/(16)) of the itself.what is the the rate percent?

$${the}\:{intrest}\:{on}\:{surtain}\:{sum}\:{of}\:{money}\:{at}\:{the}\: \\ $$$${end}\:{of}\:\mathrm{6}.\mathrm{25}\:{year}\:{was}\:\frac{\mathrm{5}}{\mathrm{16}}\:{of}\:{the}\:{itself}.{what}\:{is}\:{the} \\ $$$${the}\:{rate}\:{percent}? \\ $$

Question Number 27015 Answers: 0 Comments: 1

find the simple intrest on rs 840 for 3 year at at 5% per annum?

$${find}\:{the}\:{simple}\:{intrest}\:{on}\:{rs}\:\mathrm{840}\:{for}\:\mathrm{3}\:{year}\:{at}\: \\ $$$${at}\:\mathrm{5\%}\:{per}\:{annum}? \\ $$

Question Number 27003 Answers: 0 Comments: 2

∫_(1/8) ^(1/2) ⌊ln ⌈(1/x)⌉⌋ dx

$$\underset{\mathrm{1}/\mathrm{8}} {\overset{\mathrm{1}/\mathrm{2}} {\int}}\:\lfloor\mathrm{ln}\:\lceil\frac{\mathrm{1}}{{x}}\rceil\rfloor\:{dx} \\ $$

Question Number 26999 Answers: 1 Comments: 0

calculate Π_(k=1) ^n cos((a/2^k )) and0<a<π then find the value of lim_(n−>∝) Σ_(k=1) ^n ln(cos((a/2^k ))).

$${calculate}\:\prod_{{k}=\mathrm{1}} ^{{n}} {cos}\left(\frac{{a}}{\mathrm{2}^{{k}} }\right)\:\:{and}\mathrm{0}<{a}<\pi\:\:{then}\:{find}\:{the}\:{value}\:{of} \\ $$$${lim}_{{n}−>\propto} \:\sum_{{k}=\mathrm{1}} ^{{n}} {ln}\left({cos}\left(\frac{{a}}{\mathrm{2}^{{k}} }\right)\right). \\ $$

Question Number 26998 Answers: 0 Comments: 0

smlify X= Π_(p=2) ^n ((p^3 −1)/(p^3 +1)) by using 1,j,j^2 and j=e^((i2π)/3) .

$${smlify}\:{X}=\:\:\prod_{{p}=\mathrm{2}} ^{{n}} \frac{{p}^{\mathrm{3}} −\mathrm{1}}{{p}^{\mathrm{3}} \:+\mathrm{1}}\:{by}\:{using}\:\mathrm{1},{j},{j}^{\mathrm{2}} {and}\:{j}={e}^{\frac{{i}\mathrm{2}\pi}{\mathrm{3}}} . \\ $$

Question Number 26997 Answers: 0 Comments: 3

let give ξ ∈C and ξ^n =1 (ξ is the n^(me) root of 1) simplify A= 1+ξ^p +ξ^(2p) +... +ξ^((n−1)p) and B= 1+2ξ +3ξ^2 +...+nξ^(n−1) .

$${let}\:{give}\:\xi\:\in\mathbb{C}\:{and}\:\xi^{{n}} =\mathrm{1}\:\left(\xi\:{is}\:{the}\:{n}^{{me}} \:{root}\:{of}\:\mathrm{1}\right) \\ $$$${simplify}\:\:{A}=\:\mathrm{1}+\xi^{{p}} +\xi^{\mathrm{2}{p}} +...\:+\xi^{\left({n}−\mathrm{1}\right){p}} \\ $$$${and}\:{B}=\:\mathrm{1}+\mathrm{2}\xi\:+\mathrm{3}\xi^{\mathrm{2}} +...+{n}\xi^{{n}−\mathrm{1}} . \\ $$

Question Number 27153 Answers: 0 Comments: 2

find the value of Π_(k=1) ^(n−1) sin(((kπ)/(2n)) ) .

$${find}\:{the}\:{value}\:{of}\:\prod_{{k}=\mathrm{1}} ^{{n}−\mathrm{1}} \:{sin}\left(\frac{{k}\pi}{\mathrm{2}{n}}\:\right)\:. \\ $$

Question Number 26993 Answers: 0 Comments: 5

Question Number 26983 Answers: 1 Comments: 0

Find (dy/dx) x^y =y^x

$$\mathrm{Find}\:\frac{\mathrm{dy}}{\mathrm{dx}}\:\:\:\: \\ $$$$\:\:\:\:\mathrm{x}^{\mathrm{y}} =\mathrm{y}^{\mathrm{x}} \\ $$

Question Number 26982 Answers: 1 Comments: 0

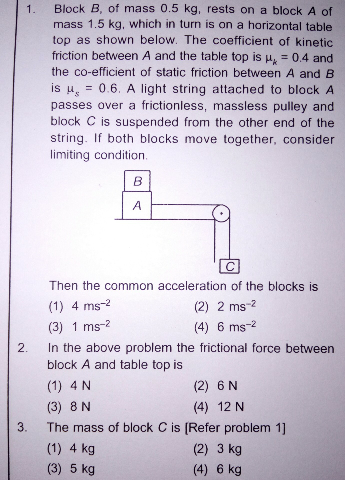
Question Number 26981 Answers: 1 Comments: 0

If the value of y satisfying the equations xsin^3 y + 3xsin y cos^2 y = 63 and xcos^3 y + 3xcosy sin^2 y = 62 simultaneously. Then tan y is equal to

$$\mathrm{If}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:{y}\:\mathrm{satisfying}\:\mathrm{the}\:\mathrm{equations} \\ $$$${x}\mathrm{sin}^{\mathrm{3}} {y}\:+\:\mathrm{3}{x}\mathrm{sin}\:{y}\:\mathrm{cos}^{\mathrm{2}} {y}\:=\:\mathrm{63}\:\mathrm{and}\:{x}\mathrm{cos}^{\mathrm{3}} {y} \\ $$$$+\:\mathrm{3}{x}\mathrm{cos}{y}\:\mathrm{sin}^{\mathrm{2}} {y}\:=\:\mathrm{62}\:\mathrm{simultaneously}. \\ $$$$\mathrm{Then}\:\mathrm{tan}\:{y}\:\mathrm{is}\:\mathrm{equal}\:\mathrm{to} \\ $$

Question Number 26959 Answers: 3 Comments: 0

Two balls, each of radius R, equal mass and density are placed in contact, then the force of gravitation between them is proportional to (1) F ∝ (1/R^2 ) (2) F ∝ R (3) F ∝ R^4 (4) F ∝ (1/R)

$$\mathrm{Two}\:\mathrm{balls},\:\mathrm{each}\:\mathrm{of}\:\mathrm{radius}\:{R},\:\mathrm{equal}\:\mathrm{mass} \\ $$$$\mathrm{and}\:\mathrm{density}\:\mathrm{are}\:\mathrm{placed}\:\mathrm{in}\:\mathrm{contact},\:\mathrm{then} \\ $$$$\mathrm{the}\:\mathrm{force}\:\mathrm{of}\:\mathrm{gravitation}\:\mathrm{between}\:\mathrm{them} \\ $$$$\mathrm{is}\:\mathrm{proportional}\:\mathrm{to} \\ $$$$\left(\mathrm{1}\right)\:{F}\:\propto\:\frac{\mathrm{1}}{{R}^{\mathrm{2}} } \\ $$$$\left(\mathrm{2}\right)\:{F}\:\propto\:{R} \\ $$$$\left(\mathrm{3}\right)\:{F}\:\propto\:{R}^{\mathrm{4}} \\ $$$$\left(\mathrm{4}\right)\:{F}\:\propto\:\frac{\mathrm{1}}{{R}} \\ $$

Pg 1813 Pg 1814 Pg 1815 Pg 1816 Pg 1817 Pg 1818 Pg 1819 Pg 1820 Pg 1821 Pg 1822

Contact: info@tinkutara.com