
AllQuestion and Answers: Page 1817
Question Number 25783 Answers: 1 Comments: 0
Question Number 26952 Answers: 1 Comments: 0

Question Number 26951 Answers: 1 Comments: 0

Question Number 25778 Answers: 1 Comments: 0
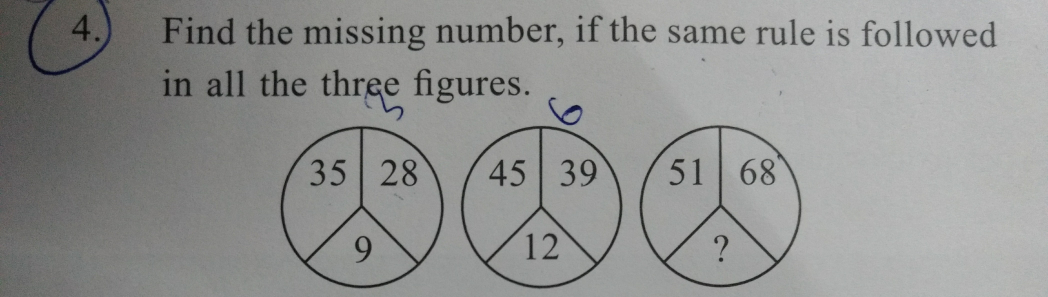
Question Number 25777 Answers: 1 Comments: 0
$$\int\frac{\mathrm{1}}{\mathrm{sin}\:{x}+\mathrm{cos}\:{x}}{dx} \\ $$
Question Number 25773 Answers: 1 Comments: 0
Question Number 25771 Answers: 1 Comments: 0
Question Number 25770 Answers: 1 Comments: 0

Question Number 25769 Answers: 0 Comments: 0
Question Number 25767 Answers: 1 Comments: 0

Question Number 25765 Answers: 0 Comments: 0
Question Number 25763 Answers: 1 Comments: 0
Question Number 25762 Answers: 0 Comments: 0
Question Number 25745 Answers: 1 Comments: 0
Question Number 25741 Answers: 1 Comments: 1
Question Number 25744 Answers: 1 Comments: 0
Question Number 25712 Answers: 1 Comments: 1

Question Number 25709 Answers: 0 Comments: 1
Question Number 25706 Answers: 0 Comments: 0

Question Number 25728 Answers: 1 Comments: 3
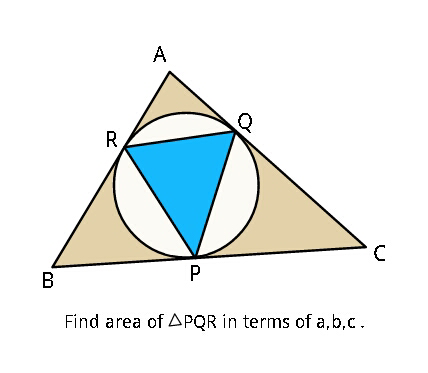
Question Number 25726 Answers: 0 Comments: 1
Question Number 25702 Answers: 1 Comments: 1

Question Number 25700 Answers: 0 Comments: 1

Question Number 25689 Answers: 0 Comments: 0

Question Number 25684 Answers: 1 Comments: 0
Question Number 25683 Answers: 0 Comments: 0
Pg 1812 Pg 1813 Pg 1814 Pg 1815 Pg 1816 Pg 1817 Pg 1818 Pg 1819 Pg 1820 Pg 1821
