
AllQuestion and Answers: Page 1816
Question Number 27294 Answers: 0 Comments: 1
Question Number 27293 Answers: 1 Comments: 0
$${L}^{−\mathrm{1}} \left(\frac{{s}^{\mathrm{3}} }{{s}^{\mathrm{4}} +\mathrm{4}}\right)=? \\ $$
Question Number 27287 Answers: 0 Comments: 1

Question Number 27283 Answers: 0 Comments: 0

Question Number 27282 Answers: 0 Comments: 1
$$\int{log}\left(\mathrm{2}+{x}^{\mathrm{2}} \right){dx} \\ $$
Question Number 27281 Answers: 1 Comments: 1
Question Number 27280 Answers: 1 Comments: 1
Question Number 27274 Answers: 0 Comments: 0

Question Number 27271 Answers: 1 Comments: 0
Question Number 27270 Answers: 0 Comments: 0
Question Number 27266 Answers: 1 Comments: 0
Question Number 27258 Answers: 0 Comments: 1
Question Number 27254 Answers: 1 Comments: 0

Question Number 27253 Answers: 0 Comments: 0

Question Number 27235 Answers: 1 Comments: 0
Question Number 27224 Answers: 1 Comments: 4

Question Number 27215 Answers: 1 Comments: 0
Question Number 27213 Answers: 1 Comments: 1
Question Number 27204 Answers: 1 Comments: 0
Question Number 27198 Answers: 1 Comments: 0

Question Number 27197 Answers: 1 Comments: 1

Question Number 27203 Answers: 2 Comments: 0
Question Number 27202 Answers: 1 Comments: 0
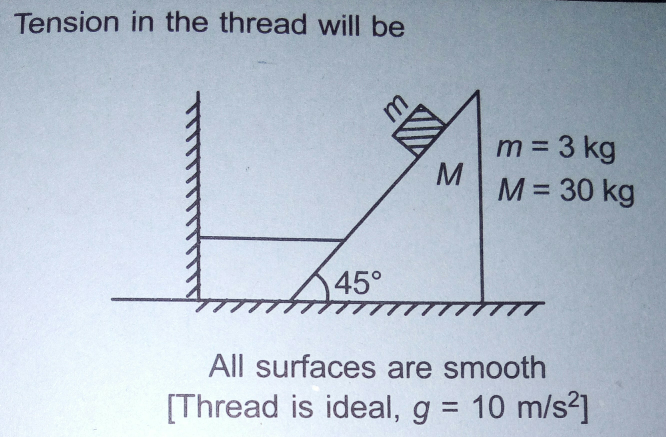
Question Number 27527 Answers: 0 Comments: 1
Question Number 27526 Answers: 1 Comments: 0
Question Number 27189 Answers: 1 Comments: 0
Pg 1811 Pg 1812 Pg 1813 Pg 1814 Pg 1815 Pg 1816 Pg 1817 Pg 1818 Pg 1819 Pg 1820
