
AllQuestion and Answers: Page 1810
Question Number 28166 Answers: 0 Comments: 0
Question Number 28165 Answers: 0 Comments: 0
Question Number 28164 Answers: 1 Comments: 0
Question Number 28163 Answers: 0 Comments: 0
Question Number 28162 Answers: 0 Comments: 1
Question Number 28161 Answers: 0 Comments: 0
Question Number 28160 Answers: 0 Comments: 2
Question Number 28159 Answers: 0 Comments: 1
Question Number 28158 Answers: 1 Comments: 0
Question Number 28185 Answers: 1 Comments: 0
Question Number 28151 Answers: 0 Comments: 4
Question Number 28139 Answers: 1 Comments: 0

Question Number 28138 Answers: 0 Comments: 1
Question Number 28202 Answers: 1 Comments: 1
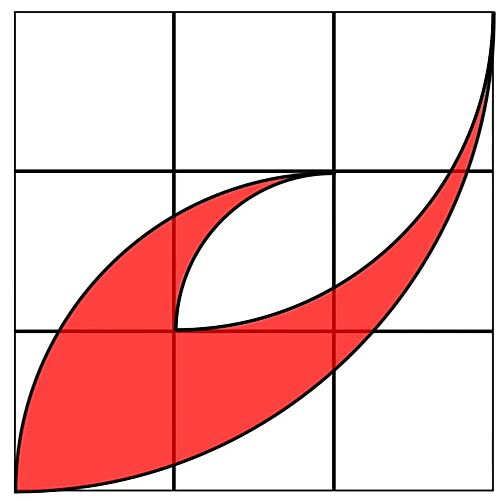
Question Number 28201 Answers: 0 Comments: 0
Question Number 28143 Answers: 1 Comments: 0
Question Number 28124 Answers: 0 Comments: 3
Question Number 28116 Answers: 0 Comments: 0

Question Number 28105 Answers: 0 Comments: 1

Question Number 28098 Answers: 1 Comments: 0
Question Number 28097 Answers: 0 Comments: 3
Question Number 28096 Answers: 0 Comments: 2
Question Number 28095 Answers: 0 Comments: 2
Question Number 28093 Answers: 0 Comments: 2
Question Number 28113 Answers: 1 Comments: 1
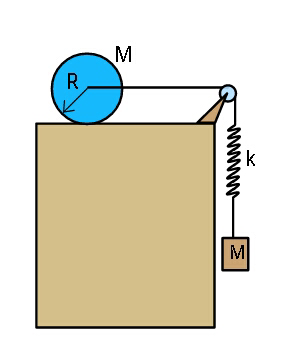
Question Number 28110 Answers: 0 Comments: 1

Pg 1805 Pg 1806 Pg 1807 Pg 1808 Pg 1809 Pg 1810 Pg 1811 Pg 1812 Pg 1813 Pg 1814
